Optical manipulation of macroscopic curved objects

Abstract

Laser has become a powerful tool to manipulate micro-particles and atoms by radiation pressure or photophoretic force, but its effectiveness for large objects is less noticeable. Here, we report the direct observation of unusual light-induced attractive forces that allow manipulating centimeter-sized curved absorbing objects by a light beam. This force is attributed to the radiometric effect caused by the curvature of the vane and its magnitude and temporal responses are directly measured with a pendulum. Simulations suggest that the force arises from the bending of the vane, which results in a temperature difference of gas molecules between the concave and convex sides due to unbalanced gas convection. This large force (~4.4 μN) is sufficient to rotate a motor with four curved vanes at speeds up to 600 r/min and even lifting a large vane. Manipulating macroscopic objects by light could have significant applications for solar radiation-powered near-space propulsion systems and for understanding the mechanisms of negative photophoretic forces.

Graphical abstract

Keywords

optical manipulation / radiometric force / geometry effect / unbalanced gas convection

Can a light beam lift and pull a centimeter-sized or larger object towards its source? While optical forces have been extensively used for manipulating microscopic objects [1−6], they are usually unnoticeable on macroscopic objects because their magnitude is usually much weaker than the gravitational force of the large objects. Generally, when an object immersed in a gaseous environment is illuminated by light, two types of optically-induced forces are generated by the light-matter interaction. One is radiation pressure force (F_RP) arising from direct momentum transfer between the object and the incident light [1, 2], which is in pN or nN range and is not sufficient to lift large objects. The other is photophoretic or radiometric force (F_RM) due to the photo-heating effect, in which the photon energy of the incident light is first converted into the thermal energy of the object due to absorption and then asymmetrical momentum transfer between the heated object and the surrounding gas molecules produces F_RM [7, 8], which can be several orders of magnitude larger than the radiation force F_RP [9, 10]. Negative optical forces have been shown to pull microscopic objects over long distances [11, 12]. The generation of optically-induced forces on macroscopic objects could trigger a new era of optomechanical applications.

Surprisingly, only a few experiments reported on the observation of optically-induced forces on centimeter-sized objects [13−17]. Magallanes and Brasselet [13] recently observed a lateral optical force of ~1 nN on a birefringence object, causing a lateral displacement of 0.5 mm in ~100 s. Wolfe et al. [14] reported a light-induced transverse force of ~400 nN acting on a horizontal vane radiometer due to the thermal creep effect. Crookes [15, 16] demonstrated a radiometric force on black-white flat vanes or cup-shaped vanes when illuminated by light, but the magnitude and dynamic properties of Crookes radiometric forces have never been directly measured [18]. Han et al. [17] investigated the radiometric force on curved vanes, focusing exclusively on enhancing the temperature gradient by coating one side with a nanoporous polymer embedded with gold nanoparticles that generate heat through light absorption. Recently, light levitation of nanostructured thin polymer films has been demonstrated by Azadi et al. [19], which might find application for sun-powered near-space flight.

Here we report a direct measurement of an optically-induced negative force on a curved light-absorbing metal vane on the order of μN that allows manipulating centimeter-sized objects by a light beam. This force allows pulling the object towards the light source by adjusting the orientation of the concave surface of the thin vane. The experiment indicates that the optically-induced force acting on macroscopic objects could be controlled by changing the surface geometry, which provides a new way to control the magnitude and direction of radiometric force.

Our experiment relies on radiometric force F_RM that acts on a thin curved vane immersed in a rarefied gas, on which a layer of black absorber is coated to increase light absorption. Generally, F_RM could be classified into two types based on the variation in surface temperature or in heat exchange coefficient of the vane, like photophoretic forces [7, 8]. The first type ($F_{\mathrm{\Delta }T}$) is caused by the temperature difference between two sides of the vane, pointing from the hot to the cold side and being repulsive from the light. The second type ($F_{\mathrm{\Delta }\alpha }$) is caused by the unbalanced heat exchange between two sides of the vane with the gas molecules even if two sides are heated at the same temperature, due to the difference in accommodation coefficient or surface geometry. The $F_{\mathrm{\Delta }\alpha }$-force could be attractive or repulsive in the light propagation direction [7−9]. Since Crookes’ pioneering work [15, 16], extensive efforts, including those by Maxwell, Reynolds, and Einstein [20−23] have been made to understand the mechanisms of radiometric forces. However, most of these efforts have focused on the force exerted on flat vanes [14, 17, 24, 25]. There are still some confusions about the dominant mechanisms in various regimes and configurations [17, 18, 23, 24, 26, 27]. Recent applications in near-space propulsion systems driven by solar radiation [28−30], micromachines [13, 17], and computational techniques [31−33] renewed the interest in F_RM. Our experiment deals with the F_RM-force created on a curved vane, which does not require a temperature gradient or a difference in accommodation coefficient on the vane and is seldom considered previously.

The experimental setup is described in detail in the electronic supplementary materials (ESM). In Fig.1, we show direct observation of a light-induced pulling or pushing force acting on a thin absorbing curved metal vane. To determine the magnitude and direction of the radiometric force F_RM, a single cylindrical vane is suspended with an ultrafine copper wire as a pendulum in a vacuum chamber and illuminated by a light beam. The Black 2.0 paint absorbs the incident light and transfers the thermal energy to the metal vane, resulting in both sides of the vane heated to an identical temperature since the used aluminum or gold vanes are very thin and excellent heat conductors. Therefore, F_RM acting on a curved metal vane is caused by unbalanced heat exchange due to the curved surface geometry. The magnitude of F_RM is determined by F_RM = mgsin$\theta$, where m is the mass of the vane, g is the gravitation acceleration, and $\theta$ is the angle displacement. When a laser beam is incident on the concave side of the vane, it is pulled towards the light source [Fig.1(b) and (c), and Supplementary Video S1]. Once the laser is turned off, the vane returns to its equilibrium position. However, when the laser beam is incident on the convex side, the vane is pushed along the light propagation direction [Fig.1(e)]. Fig.1(d) shows the dependence of the force magnitude on the gas pressure with a constant laser power. The maximum angle displacement is 0.172 rad at 0.1‒0.2 Torr corresponding to Knudsen number Kn~0.1, and thus the maximum pulling force is ~4.4 μN from the known density and volume of the vane, where Kn = $\lambda$/W is defined as the ratio of the molecular mean free path $\lambda$ of the surrounding gas to the characteristic length W of the vane [17]. It should be noted that the radiation pressure force (=P/c) is ~2.3 nN, about three orders of magnitude smaller than F_RM, where P is the absorbing power of incident laser, and c is the speed of light.

Fig.1 Laser manipulation of a curved vane. (a) Schematic of the setup for pulling a curved vane with a laser beam. The light-absorbing cylindrical aluminum vane (8 mm × 8 mm in size, 15 μm in thickness) is suspended as a pendulum by an ultrafine copper wire and is pulled toward the laser beam. (b) Position of the black curved vane without laser illumination. (c) Position of the curved vane under laser illumination. (d) Angular displacement of the pendulum as a function of gas pressure. (e) Angular displacement as a function of the central angle of the vane at a pressure of 0.1 Torr. The laser power is 0.7 W at a wavelength of 450 nm.

Full size|PPT slide

Fig.1(e) shows that for a given size of the cylindrical vane, F_RM depends on the curvature or central angle ${\Theta }_0$ of the cylinder surface, where ${\Theta }_0$ = W₀/R in radian, in which W₀ is the arc length and R is the radius. F_RM is attractive for the illumination on the concave side (${\Theta }_0$ > 0). F_RM is repulsive for the illumination on the convex side (${\Theta }_0$ < 0). For a flat vane (${\Theta }_0$ = 0), F_RM is zero so that it is not deflected by the laser beam, and this is a proof of no temperature variation between the opposite sides of the aluminum vane.

We explain the negative $F_{\mathrm{R}\mathrm{M}}$ observed on the curved vane as resulting from a combination of pressure and shear forces, in contrast to the area force, edge force, and shear force acting on a flat vane with a temperature gradient [18, 31]. When a heated vane at a temperature T_s is immersed in a rarefied gas with a temperature T₀ (T₀<T_s), heat exchange between the vane and surrounding molecules occurs via molecular collisions and convectional gas flow (Fig.2). The pressure force (like the area force and edge force) is caused by the difference in momentum flux between the gas molecules leaving the two sides of the vane and acts in the normal direction to the surface [31]. The shear force is in the tangential direction of the surface and is caused by the interaction of thermal creep flow due to the Reynolds effect [21], since the force on the gas is equal and opposite to the force on the surface.

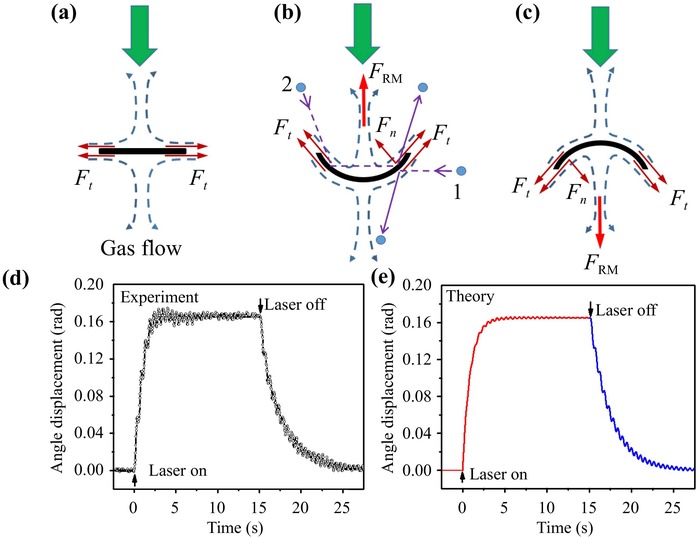
Fig.2 Radiometric force ($F_{\mathrm{R}\mathrm{M}}$) on a heated curved vane due to molecular collisions. (a) Schematic showing tangential shear forces ($F_t$) on the surface of a hot flat vane caused by gas flow. The net $F_{\mathrm{R}\mathrm{M}}$ on the flat vane is zero due to geometric balance. (b) Normal pressure force ($F_n$) and shear force on a surface element of a concave vane, with the total $F_{\mathrm{R}\mathrm{M}}$ pointing in the upward direction. Molecules incident on the convex side experience single collisions, while molecules incident on the concave side undergo multiple collisions. (c) $F_{\mathrm{R}\mathrm{M}}$ acting on a convex vane. (d) Experimental angular displacement versus time of a pendulum (with an 8 mm × 8 mm cylindrical vane) when a 1 W laser beam is turned on or off at a pressure of 0.1 Torr. (e) Theoretical modeling of the dynamic angular displacement of the pendulum, with rise time ${\tau }_r$ = 0.9 s, fall time ${\tau }_f$ = 2.4 s, damping coefficient $\beta$ = 0.12 s−1, and pendulum length $L$ = 70 mm.

Full size|PPT slide

It has been shown that the gas temperature near the edge is lower than that near the center of a hot vane, so that a gas flow parallel to the surface moves from the edge to the center region [14, 32]. If the vane is flat [Fig.2(a)], the net F_RM is zero because F_n and F_t are cancelled due to the balance in heat exchange between two sides [31, 32]. However, if the vane is curved and the concave side is illuminated [Fig.2(b)], a non-zero F_RM is generated in the upward direction. As shown in the Supplementary Materials, F_n acting on a curved vane is generated by the unbalanced momentum flux between the molecules leaving the concave and convex sides of the vane and acts in the direction towards the concave side, because the molecules incident on the convex side only experience single reflection but the molecules incident on the concave side may experience multiple reflections due to the geometry effect. In the free-molecular regime (Kn > 10 at low gas pressure), the pressure force is the dominant mechanism of F_RM [20]. When the pressure increases to transitional flow region (0.01 < Kn < 10), the molecule flux hitting on the vane increases, but the molecular mean free path is reduced so that the collisions between the incoming molecules and the reflected molecules will equilibrate the momentum difference due to the multiple reflections on the concave side. Thus, the pressure force would be optimum at Kn~0.1 and decreases in the continuum flow regime. On the other hand, in the transitional flow region, the shear force plays an important role since the thermal creep flow of gas molecules occurs at a low Kn value [14, 31]. As shown in Fig.2(b), the net shear force is toward the concave side. Therefore, the integration of the pressure force and shear force over the entire surface generates a non-zero attractive F_RM pointing to the upward direction. Similarly, if the convex side of the vane is illuminated [see Fig.2(c)], F_RM acting on it is repulsive, pointing to the downward direction.

When the illumination light is turned on or off, F_RM is changed in response to the change in temperature T_s of the vane. To determine how fast F_RM is changed temporally, we measure the dynamic motion of the vane in a pendulum. Fig.2(d) shows the angle displacement−time graph of the pendulum when the laser beam is turned on or off, indicating that the vane moves to the steady-state position with a fast rise time and moves back with a slower fall time and with an oscillation frequency of 1.88 Hz. The dynamic motion of the vane can be described by the pendulum equation

(1)$mL\ddot{\theta }=F_{\mathrm{R}\mathrm{M}}-F_{\mathrm{R}\mathrm{P}}-mg\mathrm{s}\mathrm{i}\mathrm{n}\theta -\gamma L\dot{\theta },$

where L is the length of the pendulum, F_RP is the radiation pressure force that can be neglected as F_RP $\ll$ F_RM, and $\gamma$ is Stokes’ drag constant. In a steady state after the laser beam is turned on, F_RM reaches the maximum F_RM,0 = mgsin${\theta }_0$, where ${\theta }_0$ is the steady-state deflection angle. Since F_RM is proportional to (T_s−T₀) [7, 8], which changes exponentially when the laser power is modulated [9], it can be expressed as F_RM(t) = F_RM,0[1−exp(−t/${\tau }_r$)] or F_RM(t) = F_RM,0exp(−t/${\tau }_f$), where ${\tau }_r$ is the rise time and ${\tau }_f$ is the fall time of the vane’s temperature when the laser beam is turned on or off, respectively. Equation (1) can be expressed as

(2)$\ddot{\theta }+2\beta \dot{\theta }+{\omega }_0^2\theta =f_0(1-{\mathrm{e}}^{-t/{\tau }_r}),$

or

(3)$\ddot{\theta }+2\beta \dot{\theta }+{\omega }_0^2\theta =f_0{\mathrm{e}}^{-t/{\tau }_f},$

where $\beta$ = $\gamma$/(2m), f₀ = F_RM,₀/(mL), and ${\omega }_0=\sqrt{g/L}$ is the natural frequency, $\omega =\sqrt{{\omega }_0^2-{\beta }^2}$ is the oscillation frequency of the pendulum. Fig.2(e) shows the theoretical modeling of dynamic angular displacement of the pendulum with ${\tau }_r$ = 0.9 s, ${\tau }_f$ = 2.4 s, and $\beta$ = 0.12 s⁻¹, where ${\omega }_0$ = 2π × 1.884 Hz calculated from the length L = 70 mm, which is consistent with the experimental observation [Fig.2(d)].

Using numerical simulation, we can study the influence of the vane’s geometry on the velocity distribution and temperature distribution of the gas molecules surrounding the vane and thus simulate pressure force and shear force (see ESM for details). The Comsol Multiphysics^TM simulation software is adopted to simulate the metal vane in the slip flow regime (0.001 < Kn ≤ 0.1) [34]. It is found that the bending of the vane restrains gas convection on the concave side, resulting in different gas temperature distribution from that on the convex side (shown in Fig.3). The bending of the vane causes an unbalanced temperature gradient (dT/dx) in gas molecules between the concave and convex sides.

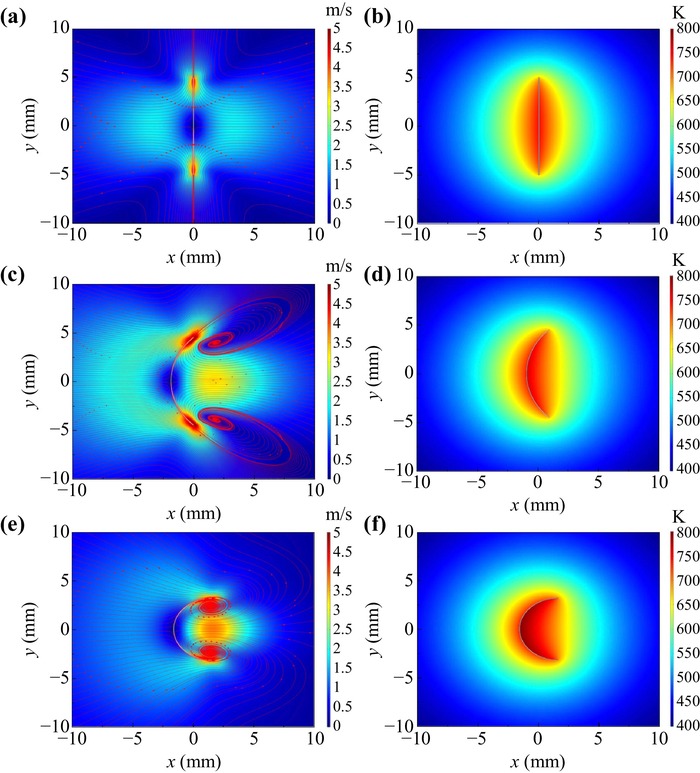
Fig.3 The distribution of the velocity and temperature of gas molecules near the vane on the horizontal plane bisecting the vane. (a, b) The velocity and temperature distribution of gas molecules near a flat vane. (c, d) The velocity and temperature distribution of gas molecules near a curved vane with the center angle of 90°. (e, f) The velocity and temperature distribution of gas molecules near a curved vane with the center angle of 180°. These three vanes have the same size with different degrees of bending.

Full size|PPT slide

The Chapman‒Enskog method was used by Scandurra et al. [35] to derive the pressure force and shear force acting on the unit area of the vane surface from the temperature gradients (dT/dx) of gas molecules perpendicular and parallel to the vane surface (see ESM). Thus, the net radiometric force F_RM acting on the curved vane can be numerically calculated from the temperature gradient of gas molecules, which consists of pressure force F_n and shear force F_t. The simulation results show that the dependence of radiometric force on the bending degree of the vane (Fig. S6) is in good agreement with the experimental results [Fig.1(e)]. In the slip flow regime with Kn~0.1, the pressure force and shear force have the same order of magnitude, and both increase with the center angle of the vane (Fig. S7).

Fig.4 shows that F_RM can be used to drive the rotation of a motor with four-curved vanes. When the concave side of the cylindrical aluminum vane is illuminated by a laser beam, the attractive F_RM pulls the vane towards the laser beam (Supplementary Video S2). When the convex side of the vane is illuminated, the repulsive F_RM turns the vane away from the beam, in the same rotation direction as the Crookes radiometer with black-white flat vanes [Fig.4(b)]. When the vane is flat, no rotation is observed, indicating no temperature difference between the two sides of the vane. Fig.4(f) shows the rotation speed of Crookes radiometer with concave, flat-plate, and convex aluminum vanes with the same size as the function of the laser power. Figure S4 shows the dependence of the rotation speed of a motor with smaller cylindrical vanes (10 mm × 10 mm) on the central angle and gas pressure, with a speed up to 600 r/min. It should be noted that the difference between our experiment and previous experiments [15, 17, 23] is that the spot size of the illumination light is smaller than the size of single curved vanes so that only one vane is illuminated at any time during the rotation. This excludes the complicated procedures to determine the force direction on cup-shaped vanes in Crookes experiment with a candle illumination [15], and excludes the possibility of the asymmetric heating that could result in a temperature gradient between two sides of the vanes in a micromotor [17].

Fig.4 Crookes radiometer with four-curved vanes or black-white flat vanes. (a) When a concave absorbing aluminum surface is illuminated by a laser beam, the pulling force turns the vane towards the beam. (b) When a black-white flat paper vane is illuminated, the pushing force turns the vane away from the beam. (c‒e) Laser-induced attractive, zero, or repulsive force on a concave (c), flat-plate (d), or convex surface (e). (f) Rotation speed of Crookes radiometer with concave, flat-plate, and convex aluminum vanes with the same size (16 mm × 16 mm × 0.05 mm) verse the laser power. The pressure is 0.02 Torr.

Full size|PPT slide

By reducing the thickness of the vane, the magnitude of the radiometric force can be greater than the gravitational force. In Figs. S2, S3, and Supplementary Video S3, we show that the attractive radiometric force is large enough to overcome the gravitational force F_G and lift an ultrathin curved gold vane off the ground by the laser illumination.

Radiometric force F_RM on macroscopic objects could be applied for near-space propulsion systems in the low-pressure environment [28−30]. It has been proposed that an array of multiple planar vanes with a thermal insulator between two surfaces powered by solar radiation could be employed in radiometric-based propulsion systems for a vehicle operating at an altitude from 40 to 80 km. However, this first-type F_RM is repulsive along solar radiation direction so that the vehicle is generally pushed in one direction. If the planar multiple vanes are replaced by multiple curved vanes, the generated force can be attractive or repulsive. Thus, the propulsion direction of the vehicle could be controlled by turning the angle of the curved vanes relative to the solar radiation direction.

In summary, we have measured the magnitude and dynamic properties of light-induced attractive and repulsive radiometric forces on macroscopic curved metal vanes. The radiometric force is caused by the curvature effect of the vane surface, which results in unbalanced temperature gradients of gas molecules between the concave and the convex sides due to the confinement of the gas convection on the concave side. We demonstrated that the radiometric force can be used to drive the rotation of a motor with four-curved vanes at a high speed. This attractive radiometric force can be greater than the gravitational force and lift a centimeter-sized gold leaf by a light beam. Light-induced attractive forces on macroscopic objects could find potential applications for radiometric-based near-space propulsion systems.

References

[1] A. Ashkin, J. M. Dziedzic, and T. Yamane, Optical trapping and manipulation of single cells using infrared laser beams, Nature 330(6150), 769 (1987)

[2] D. G. Grier, A revolution in optical manipulation, Nature 424(6950), 810 (2003)

[3] J. Stajic, E. Hand, and J. Yeston, Manipulating ultracold matter, Science 357(6355), 984 (2017)

[4] M. Wu, D. Ling, L. Ling, W. Li, and Y. Li, Stable optical trapping and sensitive characterization of nanostructures using standing-wave Raman tweezers, Sci. Rep. 7(1), 42930 (2017)

[5] D. E. Smalley,E. Nygaard,K. Squire,J. Van Wagoner,J. Rasmussen,S. Gneiting,K. Qaderi,J. Goodsell,W. Rogers,M. Lindsey,K. Costner,A. Monk, M. Pearson,B. Haymore,J. Peatross, A photophoretic-trap volumetric display, Nature 553(7689), 486 (2018)

[6] J. Lu, H. Yang, L. Zhou, Y. Yang, S. Luo, Q. Li, and M. Qiu, Light-induced pulling and pushing by the synergic effect of optical force and photophoretic force, Phys. Rev. Lett. 118(4), 043601 (2017)

[7] O. Jovanovic, Photophoresis — Light induced motion of particles suspended in gas, J. Quant. Spectrosc. Radiat. Transf. 110(11), 889 (2009)

[8] H. Horvath, Photophoresis — a forgotten force?, Kona 31, 181 (2014)

[9] G. Chen, L. He, M. Wu, and Y. Li, Temporal dependence of photophoretic force optically induced on absorbing airborne particles by a power-modulated laser, Phys. Rev. Appl. 10(5), 054027 (2018)

[10] V. G. Shvedov, A. V. Rode, Y. V. Izdebskaya, A. S. Desyatnikov, W. Krolikowski, and Y. S. Kivshar, Giant optical manipulation, Phys. Rev. Lett. 105(11), 118103 (2010)

[11] V. Shvedov,A. R. Davoyan,C. Hnatovsky,N. Engheta,W. Krolikowski, A long-range polarization-controlled optical tractor beam, Nat. Photonics 8(11), 846 (2014)

[12] J. Lin, A. G. Hart, and Y. Li, Optical pulling of airborne absorbing particles and smut spores over a meter-scale distance with negative photophoretic force, Appl. Phys. Lett. 106(17), 171906 (2015)

[13] H. Magallanes and E. Brasselet, Macroscopic direct observation of optical spin-dependent lateral forces and left-handed torques, Nat. Photonics 12(8), 461 (2018)

[14] D. Wolfe,A. Larraza,A. Garcia, A horizontal vane radiometer: Experiment, theory, and simulation, Phys. Fluids 28(3), 037103 (2016)

[15] W. Crookes,XV . On attraction and repulsion resulting from radiation, Philos. Trans. R. Soc. Lond. 164, 501 (1874)

[16] W. Crookes, On repulsion resulting from radiation. Parts III & IV, Philos. Trans. R. Soc. Lond. 166, 325 (1876)

[17] L. H. Han, S. Wu, J. C. Condit, N. J. Kemp, T. E. Milner, M. D. Feldman, and S. Chen, Light-powered micromotor driven by geometry-assisted, asymmetric photon-heating and subsequent gas convection, Appl. Phys. Lett. 96(21), 213509 (2010)

[18] A. Ketsdever, N. Gimelshein, S. Gimelshein, and N. Selden, Radiometric phenomena: From the 19th to the 21st century, Vacuum 86(11), 1644 (2012)

[19] M. Azadi, G. A. Popov, Z. Lu, A. G. Eskenazi, A. J. W. Bang, M. F. Campbell, H. Hu, and I. Bargatin, Controlled levitation of nanostructured thin films for sun-powered near-space flight, Sci. Adv. 7(7), eabe1127 (2021)

[20] J. C. Maxwell, VII. On stresses in rarified gases arising from inequalities of temperature, Philos. Trans. R. Soc. Lond. 170, 231 (1879)

[21] O. Reynolds, XVIII. On certain dimensional properties of matter in the gaseous state, Philos. Trans. R. Soc. Lond. 170, 727 (1879)

[22] A. Einstein, Zur Theorie der Radiometrerkrafte, Eur. Phys. J. A 27(1), 1 (1924)

[23] G. W. Evan, Radiometers with curved vanes, Science ns-2(29), 215 (1883)

[24] A. D. Strongrich, W. J. O’Neill, A. G. Cofer, and A. A. Alexeenko, Experimental measurements and numerical simulations of the Knudsen force on a non-uniformly heated beam, Vacuum 109, 405 (2014)

[25] A. Ventura, N. Gimelshein, S. Gimelshein, and A. Ketsdever, Effect of vane thickness on radiometric force, J. Fluid Mech. 735, 684 (2013)

[26] A. Passian, R. J. Warmack, T. L. Ferrell, and T. Thundat, Thermal transpiration at the microscale: a Crookes cantilever, Phys. Rev. Lett. 90(12), 124503 (2003)

[27] T. Zhu, W. Ye, and J. Zhang, Negative Knudsen force on heated microbeams, Phys. Rev. E 84(5), 056316 (2011)

[28] B. M. Cornella, A. D. Ketsdever, N. E. Gimelshein, and S. F. Gimelshein, Analysis of multivane radiometer arrays in high-altitude propulsion, J. Propuls. Power 28(4), 831 (2012)

[29] M. Young,S. Keith,A. Pancotti, An overview of advanced concepts for near space systems, in: 45th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, American Institute of Aeronautics and Astronautics, 2009

[30] I. Levchenko, K. Bazaka, S. Mazouffre, and S. Xu, Prospects and physical mechanisms for photonic space propulsion, Nat. Photonics 12(11), 649 (2018)

[31] R. W. Bosworth and A. D. Ketsdever, Determination of the effect of particle thermal conductivity on thermophoretic force, AIP Conf. Proc. 1786, 060003 (2016)

[32] S. Taguchi and K. Aoki, Motion of an array of plates in a rarefied gas caused by radiometric force, Phys. Rev. E 91(6), 063007 (2015)

[33] T. Baier, S. Hardt, V. Shahabi, and E. Roohi, Knudsen pump inspired by Crookes radiometer with a specular wall, Phys. Rev. Fluids 2(3), 033401 (2017)

[34] The simulation was carried out on the Tianhe-2 supercomputer system of the National Supercomputing Center in Guangzhou.

[35] M. Scandurra, F. Iacopetti, and P. Colona, Gas kinetic forces on thin plates in the presence of thermal gradients, Phys. Rev. E 75(2), 026308 (2007)

Declarations

The authors declare that they have no competing interests and there are no conflicts.

Electronic supplementary materials

The online version contains supplementary material available at https://doi.org/10.15302/frontphys.2025.012201.

Acknowledgements

This work was supported by the National Natural Science Foundation of China (Grant No. 61775036) and the high-level talents program of Dongguan University of Technology (Grant No. KCYCXPT2017003).

RIGHTS & PERMISSIONS

2024 Higher Education Press