Klein tunneling in phononic crystals: A step case

Abstract

In a recent paper [Jiang, et al., Science 370, 1447 (2020)], it was reported that zero reflection or Klein tunneling can be observed for normally incident quasiparticles upon a potential barrier constructed by two phononic crystals (PCs) with Dirac cone band structures. Here, we develop a first-principles approach for accurate computation of the reflection of quasiparticles by a potential step with two PCs at normal incidence. Strikingly, it is found that minimal reflection of quasiparticles ($R_m$ = 0.8%) occurs at an energy below the potential step, while moderate reflection ($R_d$ = 17%) remains at the center energy of the potential step, even with the PCs adopted by Jiang et al. A physical model is presented to understand such phenomena, where the zigzag interface in the step serves as a graded antireflection layer and reflection increases dramatically near the band gap of PCs. Two solutions are also shown for realizing lower $R_d$ or even ideal Klein tunneling in PCs, with reducing the difference between the two PCs or enhancing the antireflection effect of the interface. Our work reveals the effects of the zigzag interface and band gap on Klein tunneling in PCs, which will be inspiring for exploring more fascinating phenomena of quasiparticles in classical wave systems.

Graphical abstract

Keywords

Klein tunneling / phononic crystals

1 Introduction

One of the most fascinating phenomena in quantum mechanics is that under normal incidence, relativistic Dirac particles are not reflected by a potential step with any height, and they can thus completely pass through a potential barrier with any height and width. This effect was first predicted by Oscar Klein in 1929 and became known as Klein tunneling [1]. However, due to extreme difficulties in experiments, this intriguing phenomenon has not been verified for high-energy particles [2].

Recently, it was found that a graphene monolayer contains quasiparticles with massless relativistic dispersion known as Dirac cone band structures [3–7]. Measurement of electrical resistance showed some indirect features for Klein tunneling of quasiparticles in graphene systems [8–10], though its direct evidence remains absent due to the experimental difficulties in obtaining an ideal sample and highly-collimated electron beam [11]. To circumvent such problems, ones began to search for alternative systems with Dirac quasiparticles that were convenient for experimentation [12–24]. A major breakthrough was achieved by Jiang et al. [25], demonstrating that Klein tunneling of quasiparticles can be observed in phononic crystals (PCs) with Dirac cone band structures. However, since quasiparticles in PCs manifest as Bloch waves with complex mode profiles [13], the transmission data were acquired in an indirect way [25]. Moreover, the fundamental process in Klein tunneling, namely zero reflection of quasiparticles by a potential step with two PCs at normal incidence, has not been verified yet by either first-principles simulations or experimental measurements. Therefore, it remains unknown whether ideal Klein tunneling ($R$ = 0) can occur for quasiparticles in the PCs adopted by Jiang et al. [25].

In this paper, we develop a first-principles approach to accurately compute the reflection of quasiparticles by a potential step with two PCs at normal incidence. Strikingly, it is found that minimal reflection of quasiparticles ($R_m$ = 0.8%) occurs at an energy below the potential step, while moderate reflection ($R_d$ = 17%) remains at the center energy of the potential step even with the PCs adopted by Jiang et al. [25]. We will provide a physical model to understand such phenomena and show solutions to realize lower reflection R_d or even ideal Klein tunneling in PC steps.

2 Results

Our potential step is constructed by a triangular lattice of rigid cylinders in air, with a lattice constant of a, as shown in Fig.1(a). The cylinders have a height h and are placed between two parallel rigid boards with a distance of $h_b$. The cylinders are mounted on the lower board at $z$ = 0, and they have a radius of $r_1$ ($r_2$) on the left (right) side of the dashed line, constituting PC1 (PC2). For harmonic acoustic waves with angular frequency ω, the sound pressure in the system can be written as Re$\left[P(x,y,z){\mathrm{e}}^{-\mathrm{i}\omega t}\right]$ with P satisfying three-dimensional Helmholtz equation [25, 26]:

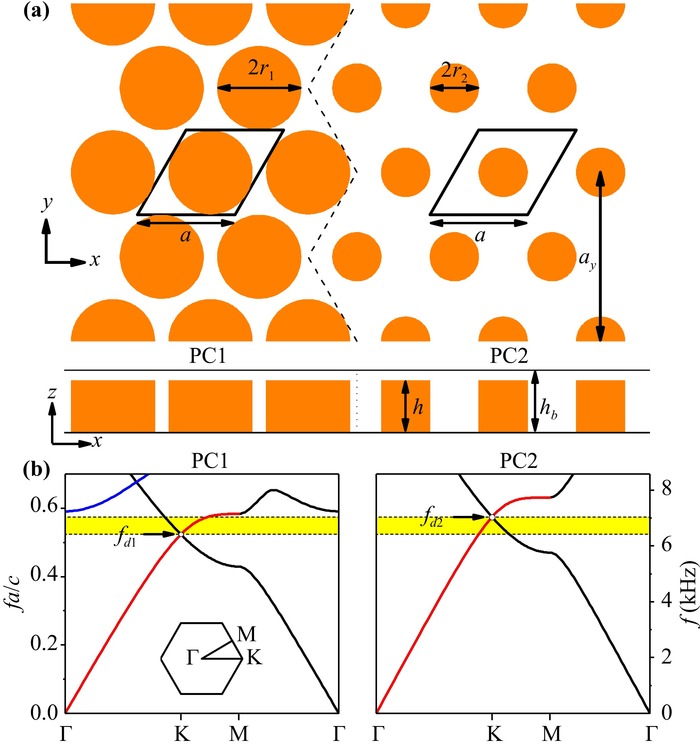
Fig.1 A potential step constructed by a triangular lattice of rigid cylinders in air. The cylinders have a height of h and are placed between two parallel rigid boards with a distance of $h_b$. The cylinders on the left (right) side of the dashed line have a radius of $r_1$ ($r_2$), constituting PC1 (PC2). The two PCs have a common lattice constant a. (a) Top (top panel) and side (bottom panel) views of the system, which has a period length of $a_y=\sqrt{3}a$ in the y direction. (b) Phononic band structures of PC1 and PC2, where $a$= 28 mm, $r_1$ = 12 mm, $r_2$ = 7 mm, $h$ = 15 mm, $h_b$ = 18 mm, and $f_{d1}$ ($f_{d2}$) is the Dirac point frequency of PC1 (PC2).

Full size|PPT slide

(1)${\mathrm{\nabla }}^{2}P+k^{2}P=0,$

subjected to boundary condition

(2)$\begin{array}{r}\frac{\mathrm{\partial }P}{\mathrm{\partial }\mathit{n}}=0\text{on the surfaces of cylinders and boards.}\end{array}$

Here, wavenumber $k=\omega /c$, c is the sound speed in air, and $\mathit{n}$ is the unit vector normal to the surfaces of cylinders and boards. In the following, we will adopt a commercial finite-element-method (FEM) software COMSOL MULTIPHYSICS to solve Eqs. (1) and (2).

We first consider a potential step constructed by PC1 and PC2 with parameters of $a=28$ mm, $r_1=12$ mm, $r_2=7$ mm, $h=15$ mm, and $h_b=18$ mm, which are the same as those in recent research [25]. By employing primitive unit cells [i.e., rhombuses in Fig.1(a)], phononic band structures are calculated for PC1 and PC2, as shown in Fig.1(b). Linearly dispersed Dirac cones occur near K and $K^{\mathrm{\prime }}$ points at the Brillouin corners. Since PC1 and PC2 possess the same lattice constant but different cylinder radii, they exhibit different Dirac point frequencies, with $f_{d1}=0.523c/a$ for PC1 and $f_{d2}=0.574c/a$ for PC2. Such results agree well with previous ones [25]. Moreover, we calculate the field distribution of $|P|$ for eigenmodes in band structures [see Fig. S1(b) in the Electronic Supplementary Materials (ESM)]. It is found that the field strongly (slightly) depends on z for modes in blue (red/black) curves in band structures. Hence, the blue (red/black) curves disappear (remain) in pure 2D PCs with $h_b=h\ll a$.

Reflection is then computed for the potential step impinged by normally incident quasiparticles. If ideal Klein tunneling effect occurs, the reflection of the step will be zero for frequencies between the Dirac point ones of PC1 and PC2 [25]. To verify the existence of the effect, we consider a structure with four regions, as shown in Fig.2(a). Here, region II (III) is PC1 (PC2) with a width of $20a$ in the x direction, and region I (IV) is absorptive PC1 (PC2) with a width of $80a$. For the air in region I (IV), the wavenumber $k=n\omega /c$, where $n=1+\mathrm{i}0.004|x-x_j|/a$ with $j=1$ (3) introduces a graded absorption in the x direction. We note that in experiments, such graded absorption could be realized by placing appropriate amounts of porous media (such as cotton, with a filling ratio increasing with increasing $|x-x_j|$) in the air of regions I and IV [27].

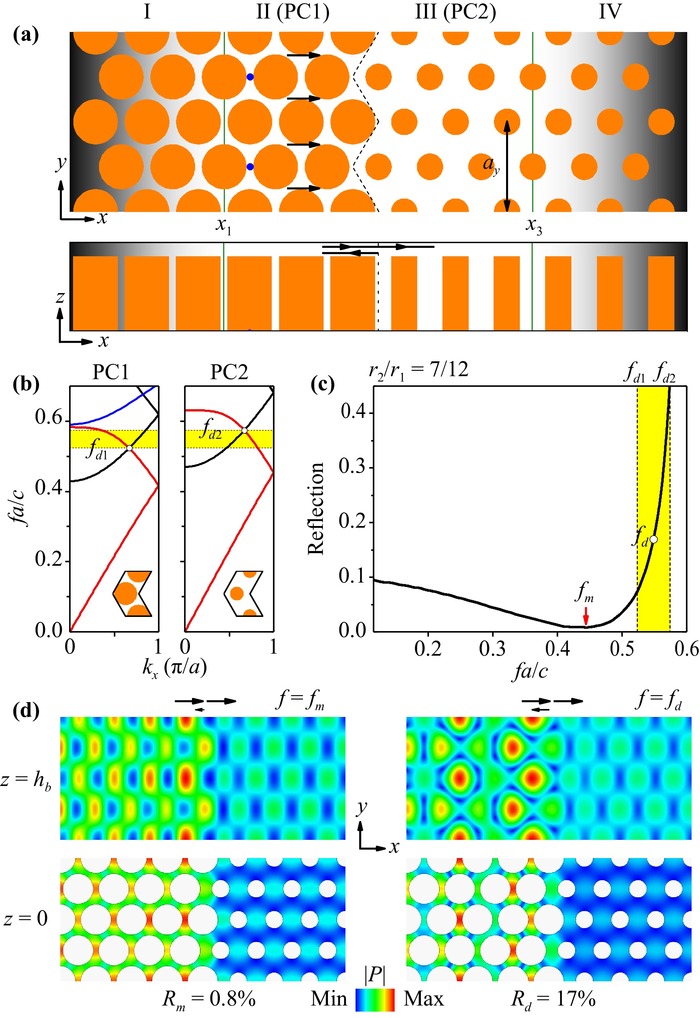
Fig.2 Normal incidence of quasiparticles upon a potential step with two PCs. The system has the same parameters as those in Fig.1(b). (a) Top (top panel) and side (bottom panel) views of the system, which has a period length of $a_y=\sqrt{3}a$ in the y direction, with quasiparticles normally entering PC2 from PC1. There exists a graded absorption in air in region I (IV) which does not reflect quasiparticles from PC1 (PC2). (b) Band structures in the x direction for PC1 and PC2. (c) Reflection spectrum of the quasiparticles. The reflection reaches minimum $R_m$ at $f_m$ and is $R_d$ at $f_d=(f_{d1}+f_{d2})/2$. (d) Distribution of $|P|$ at the upper (top panels) and lower (bottom panels) surfaces of the system. Left and right panels are at $f_m$ and $f_d$, respectively.

Full size|PPT slide

The whole structure in Fig.2(a) is periodic in the y direction, with a periodic length of $a_y=\sqrt{3}a$. A periodic array of harmonic point sources is placed at ($x_1+a/2,m\sqrt{3}a,0$) with m being integers. When the point sources have the same values in both amplitudes and phases, right-going Bloch waves (or quasiparticles) can be generated in PC1. The excited quasiparticles will be partially reflected by PC2 when $r_2\ne r_1$. However, when $r_2=r_1=12$ mm, such reflection disappears so that we can observe the field profile of $|P|$ for the excited quasiparticles in PC1.

To identify the type of excited quasiparticles, we calculate the phononic band structures in the x direction for PC1, as shown in the left panel of Fig.2(b). Here, the band structures are obtained by using the unit cell in the insets to Fig.2(b) under Bloch boundary conditions ($|k_x|<\pi /a$, $k_y=k_z=0$) [13], which can also be obtained by folding the Γ−K−M dispersion in the left panel of Fig.1(b). The corresponding field distributions of $|P|$ are also computed for the eigenmodes in band structures, as shown in Fig. S1 in the ESM. We can see that at particular points in unit cells, the field reaches maximum for the modes in red/blue curves in band structures, but it is zero for those in black curves. For the case in Fig.2(a), the point sources are placed at such field nodes ($|P|=0$) for black curves. Therefore, the modes in black curves cannot be excited, while the excited quasiparticles follow dispersion described by the red/blue curves. In the following, we will focus on Dirac quasiparticles with dispersion described by the red curves. Hence, such quasiparticles exhibit an upper bound of frequency ($f_b=0.583c/a$), above which exists a phononic band gap.

The generated quasiparticles are then normally incident from the left onto the above potential step, and they are partially reflected into PC1 and partially enter PC2. Such reflected (transmitted) quasiparticles are then gradually annihilated in region I (IV). By integrating the x-direction component of the energy flow density in the y−z plane in region III, we can obtain the transmitted energy flow $P_t$. The incident energy flow $P_i$ can also be computed by considering a control case with identical cylinders ($r_1=r_2=12$ mm). Consequently, the transmission $T=P_t/P_i$ and reflection $R=1-T$ can be obtained for the quasiparticles. The reflection can also be obtained by analyzing the field distribution in PC1. Fig.2(c) illustrates the calculated reflection for quasiparticles with different frequencies. Strikingly, the frequency ($f_m=0.44c/a$) where minimal reflection ($R_m$ = 0.8%) occurs is below the Dirac point frequencies of PC1 and PC2. The reflection is moderate ($R_d$ = 17%) at the center frequency [$f_d=(f_{d1}+f_{d2})/2$] of the potential step.

Fig.2(d) and Fig. S2 in the ESM illustrate the amplitude distribution of P-field at the top ($z=h_b$) and bottom ($z=0$) of the structure at $f_m$ and $f_d$. Periodic patterns can be seen in PC2, showing that only right-going quasiparticles (Bloch waves) occur in PC2 and region IV does not excite left-going (i.e., reflected) quasiparticles. In contrast, left-going quasiparticles exist in PC1 due to the reflection of the step. Such reflected quasiparticles interfere with incident ones, so that nonperiodic patterns are observed in PC1. Compared with the case at $f_m$, the interference is more obvious at $f_d$, confirming larger reflection at $f_d$. Since the reflection of the step is very low ($R_m$ = 0.8%) at $f_m$, the pattern in PC1 is approximately periodic.

3 Mechanism and discussion

The reflection property of the potential step can be understood by the physical model shown in Fig.3(a). A zigzag interface with thickness w exists between the two PC1 and PC2. For long wavelengths ($\lambda \gg w$), the interface can be considered flat and the reflection is nearly a constant dependent on the bulk geometries of the two PCs. For wavelengths comparable with w, the zigzag interface constitutes a graded layer that can reduce reflection. On the other hand, Bragg resonances in PC1 induce phononic band gaps, near which the reflection dramatically increases. As a result, the reflection of the potential step first decreases and then increases with increasing frequencies. The minimal reflection occurs at a frequency ($f_m$) lower than the band gap of PC1. Since the center frequency of the potential step is very close to the band gap, its reflection is moderate ($R_d$ = 17%).

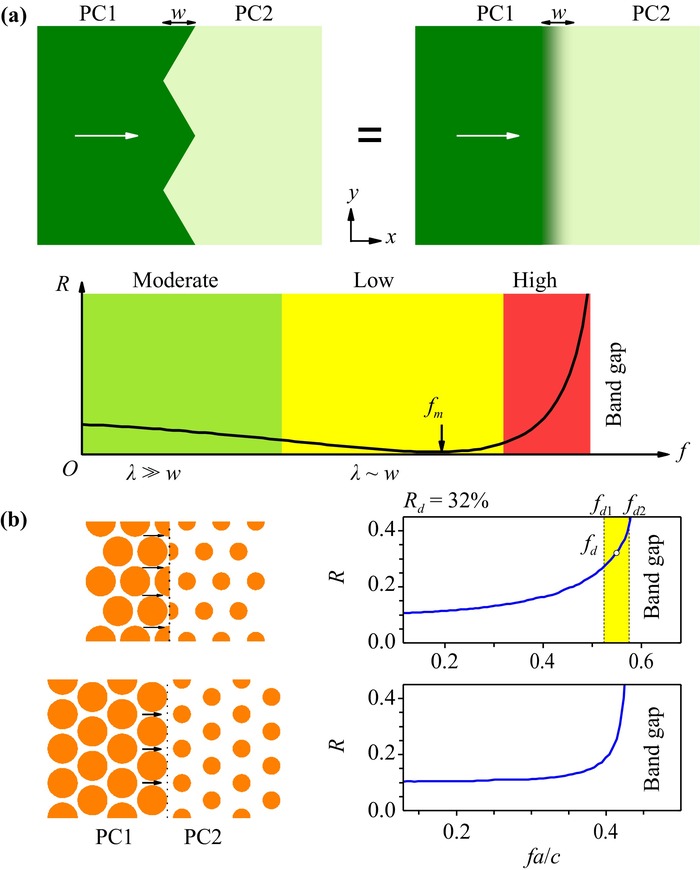
Fig.3 Mechanism for reflection of quasiparticles by a potential step with two PCs. (a) The zigzag interface between PC1 and PC2 constitutes a graded layer that can reduce reflection (top panel). Reflection increases dramatically near the phononic bandgap of PC1 (bottom panel). Hence, the reflection first decreases and then increases with increasing frequencies. The data in the bottom panel are the same as those in Fig.2(c). (b) When a straight interface exists between PC1 and PC2, the reflection increases with increasing frequencies. Parameters ($a,r_1,r_2,h,h_b$) are the same as those in Fig.1(b).

Full size|PPT slide

Our physical model suggests that if a straight interface exists between PC1 and PC2, the reflection dip at $f_d$ will disappear in Fig.2(c). To verify this viewpoint, we simulate two potential steps with straight interfaces between PC1 and PC2 [Fig.3(b)], where parameters ($a,r_1,r_2,h,h_b$) are the same as those in Fig.1(b). We can see that since straight interfaces cannot reduce reflection, the reflection increases with increasing frequencies. The lineshape of reflection spectrum is only influenced by the band gap of PC1. When a straight interface is adopted, the reflection $R_d$ will be 32% at the center frequency of the potential step, much higher than that (17%) in Fig.2(c). We note that antireflection effects have been discovered in other types of graded structures (with periodic cone arrays at surfaces) [28]. However, the thickness of the zigzag interface in Fig.2(a) is only $0.5a$, much lower than those of conventional structures [28]. Hence, its antireflection effect [see Fig.2(c) and Fig.3(a)] has not been discovered in previous studies on Klein tunneling in PCs [25].

The above studies manifest that the unique reflection spectrum in Fig.2(c) arises from the competition of the effects of the zigzag interface and band gap in the potential step. Quasiparticles can really pass through the potential step. However, since its center frequency $f_d$ is very close to the band gap, moderate reflection ($R_d$ = 17%) remains even for the case in recent research [25]. Here, we present two approaches to reduce the reflection $R_d$, as shown in Fig.4. First, we can reduce the difference between PC2 and PC1. When $r_2$ is closer to $r_1$, a smaller value of $R_d$ can be obtained. For instance, $R_d$ becomes 2% at $r_2/r_1=11/12$ [Fig.4(a)]. Secondly, we can enhance the antireflection effect of the zigzag interface by changing it into a zone with a width $w_g$, where the radii of the cylinders gradually change from $r_1$ to $r_2$ in the x direction [Fig.4(b)]. The reflection $R_d$ becomes 2% when $w_g=3.5a$. The energy width ($f_{d2}-f_{d1}$) of the potential step decreases in the first scheme, whereas it does not change in the second one. Using a larger width ($w_g$) of the zigzag zone, lower reflection $R_d$ and even ideal Klein tunneling can be realized. Such results can also be directly observed from the field distribution [Fig.4(c) and Fig. S4 in the ESM]. More results are illustrated in Fig. S5 and Fig. S6 in the ESM [29].

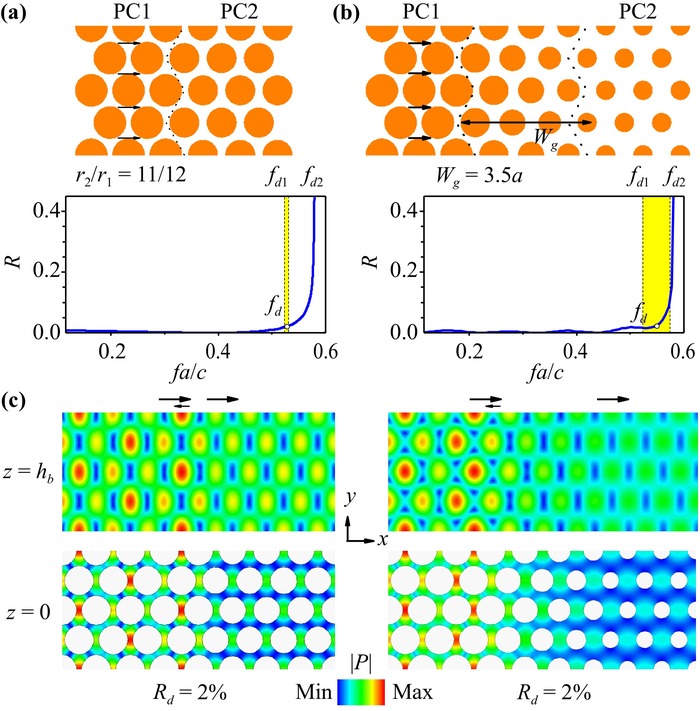
Fig.4 Achieving low reflection $R_d$ at the center frequency $f_d$ of a potential step with two PCs. Parameters ($a,r_1,h,h_b$) are the same as those in Fig.1(b). (a) Geometry and reflection spectrum for a potential step with $r_2/r_1=11/12$. (b) The same as (a) but for a potential step with $r_2/r_1=7/12$ and $w_g=3.5a$. The radii of the cylinders between the two dashed lines gradually decrease in the x direction. (c) Distribution of $|P|$ at the upper (top panels) and lower (bottom panels) surfaces of the system at $f_d$. Left and right panels are for (a) and (b), respectively.

Full size|PPT slide

4 Conclusion

In summary, we have developed a first-principles method to accurately calculate the reflection for quasiparticles normally incident upon a potential step constructed by two PCs with Dirac cone band structures. It is found that the zigzag interface in the potential step serves as a graded layer that can reduce reflection, whereas the reflection dramatically increases near the phononic band gap of PC1 due to Bragg resonance. As a result, minimal reflection of quasiparticles ($R_m$ = 0.8%) occurs at an energy below the potential step, while moderate reflection ($R_d$ = 17%) remains at the center energy of the potential step even for the case in recent research [25]. To achieve lower reflection $R_d$ or even ideal Klein tunneling in PCs, ones must either reduce the difference between the two PCs or enhance the antireflection effect of the interface (Fig.4). Our work has revealed the effects of the zigzag interface and Bragg band gap on Klein tunneling in PCs, which will be inspiring for exploring more fascinating phenomena of quasiparticles in classical wave systems.

References

[1] O. Klein, The reflection of electrons at a potential jump to the relative dynamics of Dirac, Z. Phys. 53(3−4), 157 (1929)

[2] A. Calogeracos and N. Dombey, History and physics of the Klein paradox, Contemp. Phys. 40(5), 313 (1999)

[3] K. S. Novoselov, A. K. Geim, S. V. Morozov, D. Jiang, Y. Zhang, S. V. Dubonos, I. V. Grigorieva, and A. A. Firsov, Electric field effect in atomically thin carbon films, Science 306(5696), 666 (2004)

[4] M. I. Katsnelson, K. S. Novoselov, and A. K. Geim, Chiral tunnelling and the Klein paradox in graphene, Nat. Phys. 2(9), 620 (2006)

[5] C. H. Park, L. Yang, Y. W. Son, M. L. Cohen, and S. G. Louie, Anisotropic behaviours of massless Dirac fermions in graphene under periodic potentials, Nat. Phys. 4(3), 213 (2008)

[6] C. H. Park and S. G. Louie, Energy gaps and Stark effect in boron nitride nanoribbons, Nano Lett. 8(8), 2200 (2008)

[7] A. H. Castro Neto, F. Guinea, N. M. R. Peres, K. S. Novoselov, and A. K. Geim, The electronic properties of graphene, Rev. Mod. Phys. 81(1), 109 (2009)

[8] B. Huard, J. A. Sulpizio, N. Stander, K. Todd, B. Yang, and D. Goldhaber-Gordon, Transport measurements across a tunable potential barrier in graphene, Phys. Rev. Lett. 98(23), 236803 (2007)

[9] R. V. Gorbachev, A. S. Mayorov, A. K. Savchenko, D. W. Horsell, and F. Guinea, Conductance of p-n-p structures with “Air-Bridge” top gates, Nano Lett. 8(7), 1995 (2008)

[10] A. F. Young and P. Kim, Quantum interference and Klein tunnelling in graphene heterojunctions, Nat. Phys. 5(3), 222 (2009)

[11] P. E. Allain and J. N. Fuchs, Klein tunneling in graphene: Optics with massless electrons, Eur. Phys. J. B 83(3), 301 (2011)

[12] X. Huang, Y. Lai, Z. H. Hang, H. Zheng, and C. T. Chan, Dirac cones induced by accidental degeneracy in photonic crystals and zero-refractive-index materials, Nat. Mater. 10(8), 582 (2011)

[13] J. Mei, Y. Wu, C. T. Chan, and Z. Q. Zhang, First-principles study of Dirac and Dirac-like cones in phononic and photonic crystals, Phys. Rev. B 86(3), 035141 (2012)

[14] Z. Yang, F. Gao, X. Shi, X. Lin, Z. Gao, Y. Chong, and B. Zhang, Topological acoustics, Phys. Rev. Lett. 114(11), 114301 (2015)

[15] A. B. Khanikaev, R. Fleury, S. H. Mousavi, and A. Alú, Topologically robust sound propagation in an angular-momentum-biased graphene-like resonator lattice, Nat. Commun. 6(1), 8260 (2015)

[16] M. Xiao, W. J. Chen, W. Y. He, and C. T. Chan, Synthetic gauge flux and Weyl points in acoustic systems, Nat. Phys. 11(11), 920 (2015)

[17] C. He, X. Ni, H. Ge, X. C. Sun, Y. B. Chen, M. H. Lu, X. P. Liu, and Y. F. Chen, Acoustic topological insulator and robust one-way sound transport, Nat. Phys. 12(12), 1124 (2016)

[18] J. Lu, C. Qiu, M. Ke, and Z. Liu, Valley vortex states in sonic crystals, Phys. Rev. Lett. 116(9), 093901 (2016)

[19] Y. Liu, C. S. Lian, Y. Li, Y. Xu, and W. Duan, Pseudospins and topological effects of phonons in a Kekule lattice, Phys. Rev. Lett. 119(25), 255901 (2017)

[20] Z. Zhang, Q. Wei, Y. Cheng, T. Zhang, D. Wu, and X. Liu, Topological creation of acoustic pseudospin multipoles in a flow-free symmetry-broken metamaterial lattice, Phys. Rev. Lett. 118(8), 084303 (2017)

[21] M. Dubois, C. Shi, X. Zhu, Y. Wang, and X. Zhang, Observation of acoustic Dirac-like cone and double zero refractive index, Nat. Commun. 8(1), 14871 (2017)

[22] F. Li, X. Huang, J. Lu, J. Ma, and Z. Liu, Weyl points and Fermi arcs in a chiral phononic crystal, Nat. Phys. 14(1), 30 (2018)

[23] X. J. Zhang, M. Xiao, Y. Cheng, M. H. Lu, and J. Christensen, Topological sound, Commun. Phys. 1(1), 97 (2018)

[24] C. Xu, J. Mei, G. Ma, and Y. Wu, Type-II Dirac phonons in a two-dimensional phononic crystal, APL Mater. 12(4), 041128 (2024)

[25] X. Jiang, C. Shi, Z. Li, S. Wang, Y. Wang, S. Yang, S. G. Louie, and X. Zhang, Direct observation of Klein tunneling in phononic crystals, Science 370(6523), 1447 (2020)

[26] J. Li and C. T. Chan, Double-negative acoustic metamaterial, Phys. Rev. E 70(5), 055602(R) (2004)

[27] L. Liu, H. Chang, C. Zhang, and X. Hu, Single-channel labyrinthine metasurfaces as perfect sound absorbers with tunable bandwidth, Appl. Phys. Lett. 111(8), 083503 (2017)

[28] Y. F. Huang, S. Chattopadhyay, Y. J. Jen, C. Y. Peng, T. A. Liu, Y. K. Hsu, C. L. Pan, H. C. Lo, C. H. Hsu, Y. H. Chang, C. S. Lee, K. H. Chen, and L. C. Chen, Improved broadband and quasi-omnidirectional anti-reflection properties with biomimetic silicon nanostructures, Nat. Nanotechnol. 2(12), 770 (2007)

[29] See supplemental material for more results on the topic.

Declarations

The authors declare that they have no competing interests and there are no conflicts.

Electronic supplementary materials

The online version contains supplementary material available at https://doi.org/10.15302/frontphys.2025.022204.

Acknowledgements

This work was supported by the National Key Research and Development Program of China (Nos. 2023YFA1406901 and 2018YFA0306201).

RIGHTS & PERMISSIONS

2024 Higher Education Press