Characterizing non-Hermitian topological monomodes via fractional mode charges in acoustic systems

Abstract

Non-Hermitian properties play an important role in topological acoustic systems, which can not only change the band topology but may also lead to novel applications such as non-Hermitian skin effect (NHSE). However, non-Hermitian systems, which are more closely related to real-world systems due to inevitable losses or gains, present challenges to topological classifications and boundary correspondence. Here, we demonstrate a topological monomodes based on one-dimensional (1D) Su−Schrieffer−Heeger (SSH) chains subject to non-Hermitian loss influences, which is achieved through tuning and introducing loss in the coupled acoustic cavity system. Moreover, we have extended this phenomenon from low-dimensional to high-dimensional systems. Theoretical and simulation results indicate that monomode can still be observed in non-Hermitian acoustic high-dimensional models, challenging the notion that topological states can only occur in pairs. More importantly, we have simulated the acoustic topological monomodes under non-Hermitian high-dimensional systems using acoustic local density of states (LDOS). Theoretical and simulation results demonstrate that local density of states can be used to calculate fractional charge modes and characterize topological monomodes in non-Hermitian acoustic systems. Our findings may have significant implications for the characterization of topology in non-Hermitian acoustic systems. This discovery offers a new perspective and approach to the study of non-Hermitian acoustic topology.

Graphical abstract

Keywords

topological monomodes / non-Hermitian system / local density of states

1 Introduction

In recent years, the study of Hermitian systems has gained increasing attention, as it holds significant implications for topology research. This includes categorizing and defining topological phases [1−5], as well as its application in various fields such as superconductors [6−8], cold atoms [9−14], and solid states [15, 16]. However, the non-Hermitian system is more closely related to real systems and widely exists because losses or gains may be inevitable, which brings challenges to basic topological classifications [16−18] and corresponding boundary correspondence [20−29]. Nevertheless, interesting non-Hermitian topological phenomena have also been observed, such as the occurrence of amplified or attenuated modes in the valley states [30], the degenerated Weyl evolves into the Weyl exceptional ring [31], topological interface generated by gain and loss [32, 33], or non-Hermitian skin effect protected by spectrum winding numbers [34−38]. By allowing the Hamiltonian to be non-Hermitian, we have expanded the different symmetries protected phases [17, 18]. It is commonly believed that dissipation in topological systems is detrimental, but in reality, non-Hermitianity can play a crucial role, not just as a source of disturbance, such as for robust target boundary modes [39].

Topological modes are usually measured by spectral measurements, but topological phases may be embedded in boundary states in the bulk spectrum, which makes it impossible to accurately distinguish between topological phases, especially in higher-order of topology [40, 41]. Recently, research on topological insulator crystals has revealed that crystal defects can serve as effective probes for exploring the topology of crystals beyond the edge, a finding that has been experimentally confirmed by [42−48]. The local density of states (LDOSs) of acoustic systems can be obtained through direct measurement of acoustic metamaterials [47, 48]. This is advantageous for the classification of topological acoustic crystals, but the exploration of the possibility of non-Hermitian LDOSs remains an open question.

In this work, we present a simulation demonstration of the topological monomodes corresponding to non-Hermitian acoustic system, which is determined by observing the fractional mode charge of the system. We propose a novel coupled acoustic cavity structure that introduces non-Hermitian losses in the coupled cavity, thereby enabling the realization of topological monomode, even in high-dimensional structures. We have also observed that the topological properties of this structure can be analyzed through the fractional mode charge. We have verified the existence of this topological effect both theoretically and through simulations, and we have been able to observe the topological bandgap and its associated edge and corner states. Moreover, we compared the bandgap characteristics under different loss configurations and found that even disturbance could lead to the appearance of topological states under specific conditions. This work has significant implications for understanding the topological properties of non-Hermitian acoustic crystals and exploring applications of high-order non-Hermitian topological states.

2 Model and simulation

2.1 1D SSH model

We first consider a typical example is the 1D SSH model, where units are composed of two closely coupled cavities and waveguides. As illustrated in Fig.1(a), the system can be tuned to modify its energy band structure and band gaps by controlling the sizes of internal coupling t₁ and external coupling t₂, which is accomplished through dimerization coupling [49, 50]. Then we add an additional loss is artificially introduced in one of the resonators, topological monomode emerges in Fig.1(b), which depends on the configuration of the losses. In tight-binding model, the Hamiltonian of one-dimensional finite chain is written as:

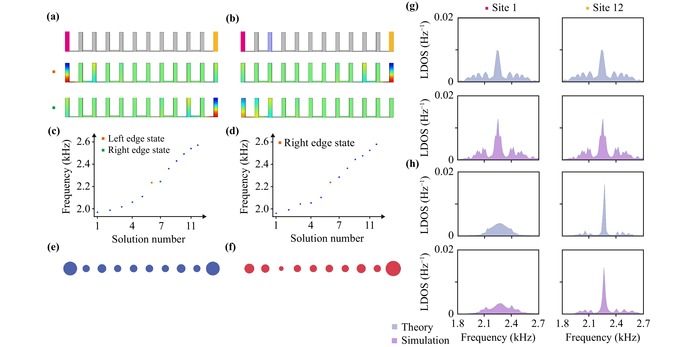
Fig.1 (a) Schematic of a 1D SSH chain of coupled acoustic system with t2/t1 = 1.33. The localization of edge modes is clearly evident, indicating the presence of well-defined eigenstates in the system. (b) Same as (a) but with an additional loss at the blue dot positioned near the left edge, one of the edge modes vanishes, revealing a monomode pattern. (c) The edge state emerges in the bandgap. (d) Same as (c) but with an additional loss. (e) The simulated mode charge of each cavity in the 1D acoustic structure is represented by filled circles in the frequency domain ranging from 1800 to 2700 Hz. The size of each circle corresponds to the simulated mode density of the resonator in that frequency band. (f) Same as (e) but with an additional loss. (g) The LDOS spectrum of site 1 and site 12 shows distinct features for different types of states in Fig.1(a). (h) Same as (g) but in Fig.1(b). The edge state appears as a single peak, the bulk states exhibits outward diffusion.

Full size|PPT slide

$H_x=\left(\begin{array}{ccccc}{E}_0& t_1& 0& \cdots & 0\\ t_1& E_0& t_2& \cdots & ⋮\\ 0& t_2& E_0& \cdots & 0\\ ⋮& ⋮& ⋮& \ddots & t_1\\ 0& \cdots & 0& t_1& E_0\end{array}\right).$

Here, $E_0$ represents the on-site potential, the interunit-cell coupling strength is stronger than the intraunit-cell coupling, which can be represented by t₂ = 1.33∗t₁. The loss can be simulated by the imaginary part of the dispersion.

2.2 Simulation calculation of local density of states

In simulations, we employed the Pressure-Sound module of COMSOL Multiphysics. For all the simulations, the density of the background medium air was set to 1.22 kg/m³, and the real part of the sound speed in air was set to 340 m/s. By adjusting the sound speed, we fitted the simulated spectrum of the resonator with only background loss (c = 340 + 2.2i m/s) and the simulated spectrum of the resonator with additional loss (c = 340 + 85.0i m/s). For the calculation of the band structure, we adopted periodic boundary conditions at the outer boundary, while the other boundaries were considered as hard boundaries. In the calculation of the finite-element eigenfrequency [Fig.1(a) and (b)], all boundaries were assumed to be hard boundaries.

We calculated the eigenstates of the 1D chain, and the 12 eigenstates correspond to the number of cavities. The edge states are located in the bandgap, and the frequencies of the edge states on the left and right boundaries are approximately the same in Fig.1(c). After adding loss, the frequency of the right edge state is shifted in Fig.1(d), which is because the loss breaks the symmetry of the one-dimensional chain. When the loss tends to be finite, one of these two zero modes acquires an energy with a negative imaginary part, while the other remains at zero energy. This phenomenon arises from the fact that we have introduced loss to only one of the two sublattices. Each edge state exists on only one sublattice, so they exhibit different energy properties. In previous studies, we have known that local state density can characterize topological states in Hermitian systems, especially in defect structures [42−44]. Then, we calculate the local state density, with calculation details referring to Ref. [47]. The acoustic LDOS is obtained from

$\rho \left(\omega ,r\right)={\displaystyle \frac{2}{\pi }}{\displaystyle \frac{1}{{\rho }_{\mathrm{a}\mathrm{i}\mathrm{r}}{c}_{\mathrm{a}\mathrm{i}\mathrm{r}}^2}}\mathrm{R}\mathrm{e}\left({\displaystyle \frac{p_{\mathrm{s}}}{u_{\mathrm{s}}{S}_{\mathrm{s}}}}\right),$

where ${\rho }_{\mathrm{a}\mathrm{i}\mathrm{r}}$ is the mass density of air, $u_{\mathrm{s}}$ is the acoustic particle velocity on the surface of monopole source, and $S_{\mathrm{s}}$ is the surface area of the source. The velocity $u_{\mathrm{s}}$ stands for the oscillating velocity of the air flows. The fractional mode charge can be obtained by integrating the LDOS above the edge state frequency in the band gap.

3 Results and discussion

3.1 The fractional mode charge of 1D topological monomode

Theoretical and simulation results show that although the bandgap is small, and the boundary states and body modes have hybridization, the fractional charge modes on the left and right edges are approximately 1, while the fractional charge modes in the middle are fractional in Fig.1(e). This feature allows us to distinguish boundary states through local state density in Fig.1(g). We also simulated topological monomode in non-Hermitian systems in Fig.1(f). By adding loss in the cavity, a monomode can be formed, and this phenomenon can be observed through local state density as well, which is similar to Hermitian systems. Specifically, the local state density of the left boundary state changes from outward diffusion in Fig.1(h), which is due to the damage of the edge modes by loss. We can also find that the fractional charge mode on the right side is significantly greater than the left side, indicating that we detect the topological monomodes. This may bring new applications to robust boundary states.

3.2 The fractional mode charge of 2D topological monomode

To further investigate the impact of loss on topology, we extend the model to two dimensions. We first solve the conventional two-dimensional (2D) SSH model and find that the eigenstate is localized at the four corners in Fig.2(a). Then, we try different operations, introducing loss along the x and y directions of each cavity, to reduce the symmetry and form diagonal eigenmodes in Fig.2(b). By further increasing the amount of loss, we break the symmetry even more, resulting in a eigenmode in Fig.2(c), which is referred to as a monomode. The existence of monomode in non-Hermitian systems is theoretically and simulatively proven through the introduction of loss in the selected crystal structure and near the corresponding edges, where one topological edge mode will decay over time. We have already simulatively realized these monomode in acoustic coupled systems. This result shows that under appropriate conditions, we can effectively control and adjust the symmetry and the number of corner modes of the acoustic coupled systems.

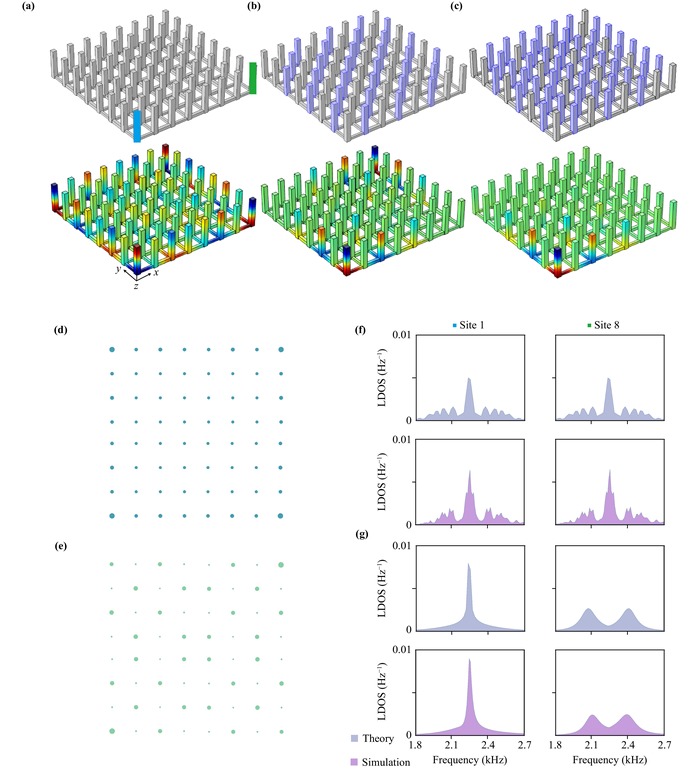
Fig.2 (a) The 2D SSH chain of coupled acoustic system with t2/t1 = 1.33. The localization of corner modes is clearly evident, indicating the presence of well-defined eigenstates in the system. (b) Same as (a) but breaking the C4 symmetry of the system to C2 symmetry, additional losses are shown by the blue dots. (c) Similar to (a), but breaking the C2 symmetry of the system to monomode pattern, further losses are demonstrated by the blue dots. (d) The simulated mode charge of each cavity in Fig.2(a) is represented by filled circles in the frequency domain ranging from 1800 to 2700 Hz. The size of each circle corresponds to the simulated mode density of the resonator in that frequency band. (e) Same as (d) but in Fig.2(b). (f) The LDOS spectrum of site 1 and site 8 shows distinct features for different types of states in Fig.2(a). (g) Same as (f) but in Fig.2(b). The corner state appears as a single peak, and the bulk states manifest as two peaks within the bandgap.

Full size|PPT slide

We are more interested in characterizing the local state density as a means of addressing this issue. When the corner and body modes are hybridized, the boundary state can be entirely located within the bandgap, making it difficult to distinguish it from the bulk state using the bulk-boundary correspondence principle. Therefore, we also calculate the local state density of each cavity in Fig.2(d). The local density of state in the corner state is significantly distinct from that in the bulk state. As shown in Fig.2(e), when loss is introduced into the system, the local state density of the double-mode corner state changes, with the peak transforming from a single to a double-peaked state. This result is attributed to the disappearance of symmetry, as evidenced by both theoretical and simulation findings in Fig.2(g) and (f). The topological monomodes induced by the introduced losses through acoustic local density of states simulated are directly observed, leading to the appearance of edge states and intermediate gap corner states, which are typical features of higher-order topology [33].

3.3 The fractional mode charge of 2D robust topological monomode

Previous research indicates that disorder typically has a significant impact on altering the energy band structure within quantum systems [51, 52]. We hope that topological monomode can also maintain their integrity without being perturbed. As a comparison, we conduct simulations to investigate the effects of random perturbations on loss configurations. To evaluate the robustness of this structure, we have introduced random losses to each cavity, which is consistent with the real-life situation, as the experimental process inevitably introduces losses. The disturbance intensity is E₀$\ast$0.25$\ast$rand(−1,1)$\ast$i, and the simulation results demonstrate that topological monomode exhibit strong robustness to perturbations in Fig.3(a) and (b). Subsequently, fractional mode charge calculations were carried out at all positions on the topological crystal, and it was found that while the fractional mode charge changed in Fig.3(c), the corner state characteristics remained evident, further demonstrating the robustness of the structure to perturbations. The simulated fractional mode charge is presented in Fig.3(d) and (e). Since each disturbance strength is random, the simulation and simulation may not be consistent. We found that although there are some fluctuations in the peaks, the overall intensity remains basically unchanged. This indicates that although there are disturbances, the overall system remains stable, which is consistent with the inevitable introduction of losses or gains in real life.

Fig.3 (a) Robust topological monomodes in Fig.2(b) with disorder introduced into structure. (b) Same as (a). (c) The simulated mode charge of each cavity in (b) is represented by filled circles in the frequency domain ranging from 1800 to 2700 Hz. The size of each circle corresponds to the simulated mode density of the resonator in that frequency band. (d) The LDOS spectrum of site 1 and site 8 shows distinct features for different types of states in the system, accompanied by fluctuations induced by added disturbances. (e) Same as (d).

Full size|PPT slide

3.4 The fractional mode charge of 3D topological monomode

Most crystals have a natural three-dimensional structure. To further demonstrate the practicality of monomode, we have extended it to a three-dimensional structure, as shown in Fig.4(a). Similar to the previous two-dimensional structure, we display the topological state of the loss structure within the three-dimensional structure. The three-dimensional structure exhibits a surface state that complicates the band structure compared to the two-dimensional structure. In addition to incorporating loss into the cavity, we have observed diagonal-symmetric corner modes in Fig.4(a) and (b), making it easier to design practical acoustic structures. We have also simulated the local density of states in the three-dimensional structure in Fig.4(c), similar to the two-dimensional structure, where the corner state displays a single peak; however, due to a symmetry break, the corner state becomes a double peak in Fig.4(d) and (e). By integrating the local density of states in the corresponding frequency domain, we obtained fractional mode charge, which indicates that the corner charge is much higher than that of the boundary state and bulk state. Therefore, we can verify the topological monomode under non-Hermitian systems through the local density of state.

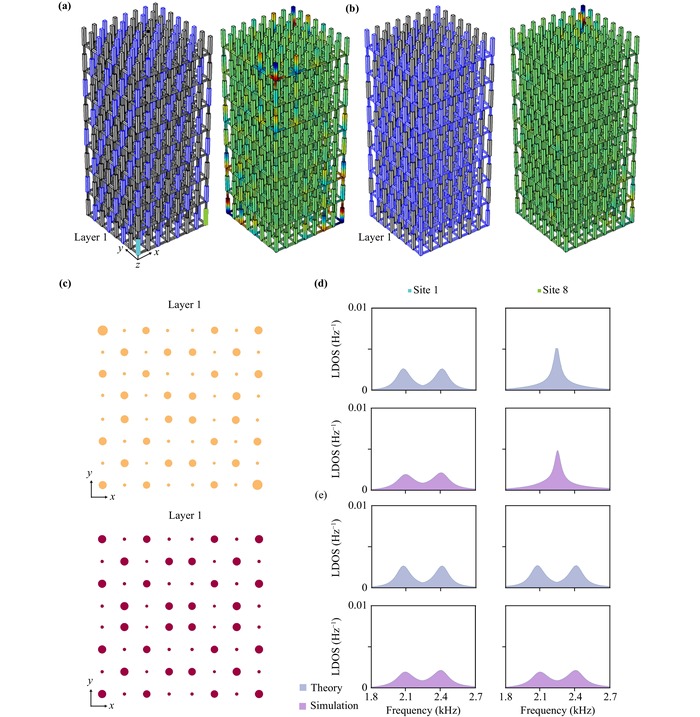
Fig.4 (a) The 3D acoustic structure of coupled acoustic system. The localization of corner modes is clearly evident, indicating the presence of well-defined eigenstates in the system. (b) Same as (a) but additional losses are shown by the blue dots. (c) The simulated mode charge of each cavity in layer 1 is represented by filled circles in the frequency domain ranging from 1800 to 2700 Hz. The size of each circle corresponds to the simulated mode density of the resonator in that frequency band. (d) The LDOS spectrum of site 1 and site 8 shows distinct features for different types of states in Fig.4(a). (e) Same as (d) in Fig.4(b).

Full size|PPT slide

4 Conclusions

In summary, we have observed topological monomodes arising from the addition of losses in non-Hermitian acoustic coupled systems. We have verified the acoustic topological insulators under different loss configurations, starting from low-dimensional structures by introducing losses in selected cavities with adequate proximity to the corresponding edges. We first achieved topological monomode in one-dimensional structures and then extended it to high-dimensional structures. More importantly, we found that topological monomodes of non-Hermitian acoustic coupled systems can be characterized by fractional mode charges, even in higher-order structures. Lastly, we evaluated the robustness of topological monomodes to disturbances, and the experimental results showed that these topological monomodes exhibited robustness and did not hybridize, pointing to huge potential applications, as non-Hermiticity can be externally modulated, reconfigurable, and active. Additionally, the tunability of losses opens up great potential for future reconfigurable devices [53, 54]. These results may be applied to explore higher-order non-Hermitian models and provide a flexible and useful scheme for characterizing topological states under non-Hermiticity.

References

[1] M. Z. Hasan and C. L. Kane, Colloquium: Topological insulators, Rev. Mod. Phys. 82(4), 3045 (2010)

[2] X. L. Qi and S. C. Zhang, Topological insulators and superconductors, Rev. Mod. Phys. 83(4), 1057 (2011)

[3] A. Bansil, H. Lin, and T. Das, Colloquium: Topological band theory, Rev. Mod. Phys. 88(2), 021004 (2016)

[4] X. G. Wen, Colloquium: Zoo of quantum-topological phases of matter, Rev. Mod. Phys. 89(4), 041004 (2017)

[5] Z. Guo,Y. Wang,S. Ke,X. Su,J. Ren, H. Chen, 1D photonic topological insulators composed of split ring resonators: A mini review, Adv. Phys. Res. 3(6), 2300125 (2024)

[6] J. Alicea, New directions in the pursuit of Majorana fermions in solid state systems, Rep. Prog. Phys. 75(7), 076501 (2012)

[7] C. W. J. Beenakker, Search for Majorana fermions in superconductors, Annu. Rev. Condens. Matter Phys. 4(1), 113 (2013)

[8] M. Sato and Y. Ando, Topological superconductors: A review, Rep. Prog. Phys. 80(7), 076501 (2017)

[9] M. Aidelsburger, M. Atala, M. Lohse, J. T. Barreiro, B. Paredes, and I. Bloch, Realization of the Hofstadter Hamiltonian with ultracold atoms in optical lattices, Phys. Rev. Lett. 111(18), 185301 (2013)

[10] M. Aidelsburger, M. Lohse, C. Schweizer, M. Atala, J. T. Barreiro, S. Nascimbène, N. R. Cooper, I. Bloch, and N. Goldman, Measuring the Chern number of Hofstadter bands with ultracold bosonic atoms, Nat. Phys. 11(2), 162 (2015)

[11] G. Jotzu, M. Messer, R. Desbuquois, M. Lebrat, T. Uehlinger, D. Greif, and T. Esslinger, Experimental realization of the topological Haldane model with ultracold fermions, Nature 515(7526), 237 (2014)

[12] N. Goldman, G. Juzeliūnas, P. Öhberg, and I. B. Spielman, Light-induced gauge fields for ultracold atoms, Rep. Prog. Phys. 77(12), 126401 (2014)

[13] N. Goldman, J. C. Budich, and P. Zoller, Topological quantum matter with ultracold gases in optical lattices, Nat. Phys. 12(7), 639 (2016)

[14] N. R. Cooper, J. Dalibard, and I. B. Spielman, Topological bands for ultracold atoms, Rev. Mod. Phys. 91(1), 015005 (2019)

[15] B. A. Bernevig, T. L. Hughes, and S. C. Zhang, Quantum spin Hall effect and topological phase transition in HgTe quantum wells, Science 314(5806), 1757 (2006)

[16] M. König, S. Wiedmann, C. Brüne, A. Roth, H. Buhmann, L. W. Molenkamp, X. L. Qi, and S. C. Zhang, Quantum spin Hall insulator state in HgTe quantum wells, Science 318, 766 (2007)

[17] Z. Gong, Y. Ashida, K. Kawabata, K. Takasan, S. Higashikawa, and M. Ueda, Topological phases of non-Hermitian systems, Phys. Rev. X 8(3), 031079 (2018)

[18] K. Kawabata, K. Shiozaki, M. Ueda, and M. Sato, Symmetry and topology in non-Hermitian physics, Phys. Rev. X 9(4), 041015 (2019)

[19] H. Zhou and J. Y. Lee, Periodic table for topological bands with non-Hermitian symmetries, Phys. Rev. B 99(23), 235112 (2019)

[20] T. E. Lee, Anomalous edge state in a non-Hermitian lattice, Phys. Rev. Lett. 116(13), 133903 (2016)

[21] D. Leykam, K. Y. Bliokh, C. Huang, Y. D. Chong, and F. Nori, Edge modes, degeneracies, and topological numbers in non-Hermitian systems, Phys. Rev. Lett. 118(4), 040401 (2017)

[22] V. M. Martinez Alvarez, J. E. Barrios Vargas, and L. E. F. Foa Torres, Non-Hermitian robust edge states in one dimension: Anomalous localization and eigenspace condensation at exceptional points, Phys. Rev. B 97(12), 121401 (2018)

[23] Y. Xiong, Why does bulk boundary correspondence fail in some non-Hermitian topological models, J. Phys. Commun. 2(3), 035043 (2018)

[24] S. Yao and Z. Wang, Edge states and topological invariants of non-Hermitian systems, Phys. Rev. Lett. 121(8), 086803 (2018)

[25] F. K. Kunst, E. Edvardsson, J. C. Budich, and E. J. Bergholtz, Biorthogonal bulk-boundary correspondence in non-Hermitian systems, Phys. Rev. Lett. 121(2), 026808 (2018)

[26] C. H. Lee and R. Thomale, Anatomy of skin modes and topology in non-Hermitian systems, Phys. Rev. B 99(20), 201103 (2019)

[27] K. Y. Bliokh, D. Leykam, M. Lein, and F. Nori, Topological non-Hermitian origin of surface Maxwell waves, Nat. Commun. 10(1), 580 (2019)

[28] K. Y. Bliokh and F. Nori, Klein-Gordon representation of acoustic waves and topological origin of surface acoustic modes, Phys. Rev. Lett. 123(5), 054301 (2019)

[29] A. Ghatak and T. Das, New topological invariants in non-Hermitian systems, J. Phys.: Condens. Matter 31(26), 263001 (2019)

[30] M. Wang, L. Ye, J. Christensen, and Z. Liu, Valley physics in non-Hermitian artificial acoustic boron nitride, Phys. Rev. Lett. 120(24), 246601 (2018)

[31] J. Liu, Z. Li, Z. G. Chen, W. Tang, A. Chen, B. Liang, G. Ma, and J. C. Cheng, Experimental realization of Weyl exceptional rings in a synthetic three-dimensional non-Hermitian phononic crystal, Phys. Rev. Lett. 129(8), 084301 (2022)

[32] H. Gao, H. Xue, Q. Wang, Z. Gu, T. Liu, J. Zhu, and B. Zhang, Observation of topological edge states induced solely by non-Hermiticity in an acoustic crystal, Phys. Rev. B 101(18), 180303 (2020)

[33] H. Gao, H. Xue, Z. Gu, T. Liu, J. Zhu, and B. Zhang, Non-Hermitian route to higher-order topology in an acoustic crystal, Nat. Commun. 12(1), 1888 (2021)

[34] K. Esaki, M. Sato, K. Hasebe, and M. Kohmoto, Edge states and topological phases in non-Hermitian systems, Phys. Rev. B 84(20), 205128 (2011)

[35] L. Herviou, J. H. Bardarson, and N. Regnault, Defining a bulk-edge correspondence for non-Hermitian Hamiltonians via singular-value decomposition, Phys. Rev. A 99(5), 052118 (2019)

[36] N. Okuma and M. Sato, Non-Hermitian topological phenomena: A review, Annu. Rev. Condens. Matter Phys. 14(1), 83 (2023)

[37] R. Lin, T. Tai, L. Li, and C. H. Lee, Topological non-Hermitian skin effect, Front. Phys. 18(5), 53605 (2023)

[38] M. J. Liao, M. S. Wei, Z. Lin, J. Xu, and Y. Yang, Non-Hermitian skin effect induced by on-site gain and loss in the optically coupled cavity array, Results Phys. 57, 107372 (2024)

[39] W. Cherifi,J. Carlström,M. Bourennane,E. J. Bergholtz, Non-Hermitian boundary state distillation with lossy waveguides, 2023)

[40] B. Xie, H. X. Wang, X. Zhang, P. Zhan, J. H. Jiang, M. Lu, and Y. Chen, Higher-order band topology, Nat. Rev. Phys. 3(7), 520 (2021)

[41] X. Zhang, H. X. Wang, Z. K. Lin, Y. Tian, B. Xie, M. H. Lu, Y. F. Chen, and J. H. Jiang, Second-order topology and multidimensional topological transitions in sonic crystals, Nat. Phys. 15(6), 582 (2019)

[42] T. Li, P. Zhu, W. A. Benalcazar, and T. L. Hughes, Fractional disclination charge in two-dimensional C_n-symmetric topological crystalline insulators, Phys. Rev. B 101(11), 115115 (2020)

[43] C. W. Peterson, T. Li, W. Jiang, T. L. Hughes, and G. Bahl, Trapped fractional charges at bulk defects in topological insulators, Nature 589(7842), 376 (2021)

[44] Y. Liu, S. Leung, F. F. Li, Z. K. Lin, X. Tao, Y. Poo, and J. H. Jiang, Bulk-disclination correspondence in topological crystalline insulators, Nature 589(7842), 381 (2021)

[45] H. X. Wang, L. Liang, B. Jiang, J. Hu, X. Lu, and J. H. Jiang, Higher-order topological phases in tunable C₃ symmetric photonic crystals, Photon. Res. 9(9), 1854 (2021)

[46] S. Wu, B. Jiang, Y. Liu, and J. H. Jiang, All-dielectric photonic crystal with unconventional higher-order topology, Photon. Res. 9(5), 668 (2021)

[47] T. Zheng, H. Ge, Z. Long, C. Xu, and M. H. Lu, Fractional mode charge of higher-order topological acoustic transport, Appl. Phys. Lett. 122(18), 183101 (2023)

[48] H. Ge, Z. W. Long, X. Y. Xu, J. G. Hua, Y. Liu, B. Y. Xie, J. H. Jiang, M. H. Lu, and Y. F. Chen, Direct measurement of acoustic spectral density and fractional topological charge, Phys. Rev. Appl. 19(3), 034073 (2023)

[49] W. A. Benalcazar, B. A. Bernevig, and T. L. Hughes, Quantized electric multipole insulators, Science 357(6346), 61 (2017)

[50] W. A. Benalcazar, B. A. Bernevig, and T. L. Hughes, Electric multipole moments, topological multipole moment pumping, and chiral hinge states in crystalline insulators, Phys. Rev. B 96(24), 245115 (2017)

[51] V. M. Martinez Alvarez and M. D. Coutinho-Filho, Edge states in trimer lattices, Phys. Rev. A 99(1), 013833 (2019)

[52] J. R. Li, C. Jiang, H. Su, D. Qi, L. L. Zhang, and W. J. Gong, Parity-dependent skin effects and topological properties in the multilayer nonreciprocal Su−Schrieffer−Heeger structures, Front. Phys. 19(3), 33204 (2024)

[53] T. Hofmann, T. Helbig, F. Schindler, N. Salgo, M. Brzezińska, M. Greiter, T. Kiessling, D. Wolf, A. Vollhardt, A. Kabaši, C. H. Lee, A. Bilušić, R. Thomale, and T. Neupert, Reciprocal skin effect and its realization in a topolectrical circuit, Phys. Rev. Res. 2(2), 023265 (2020)

[54] E. Slootman,W. Cherifi,L. Eek, R. Arouca,E. J. Bergholtz,M. Bourennane,C. M. Smith, Topological monomodes in non-Hermitian systems, 2023)

Declarations

The authors declare that they have no competing interests and there are no conflicts.

Data availability

The data that support the findings of this study are available from the corresponding author upon reasonable request.

Acknowledgements

The authors would like to acknowledge the support of the Natural Science Foundation of Guangdong, China (No. 2020A1515010634).

RIGHTS & PERMISSIONS

2024 Higher Education Press