

New nonlinear stiffness actuator with predefined torque‒deflection profile
Received date: 20 Jan 2022
Accepted date: 16 Jun 2022
Copyright
A nonlinear stiffness actuator (NSA) could achieve high torque/force resolution in low stiffness range and high bandwidth in high stiffness range, both of which are beneficial for physical interaction between a robot and the environment. Currently, most of NSAs are complex and hardly used for engineering. In this paper, oriented to engineering applications, a new simple NSA was proposed, mainly including leaf springs and especially designed cams, which could perform a predefined relationship between torque and deflection. The new NSA has a compact structure, and it is lightweight, both of which are also beneficial for its practical application. An analytical methodology that maps the predefined relationship between torque and deflection to the profile of the cam was developed. The optimal parameters of the structure were given by analyzing the weight of the NSA and the mechanic characteristic of the leaf spring. Though sliding friction force is inevitable because no rollers were used in the cam-based mechanism, the sliding displacement between the cam and the leaf spring is very small, and consumption of sliding friction force is very low. Simulations of different torque‒deflection profiles were carried out to verify the accuracy and applicability of performing predefined torque‒deflection profiles. Three kinds of prototype experiments, including verification experiment of the predefined torque‒deflection profile, torque tracking experiment, and position tracking experiment under different loads, were conducted. The results prove the accuracy of performing the predefined torque‒deflection profile, the tracking performance, and the interactive performance of the new NSA.
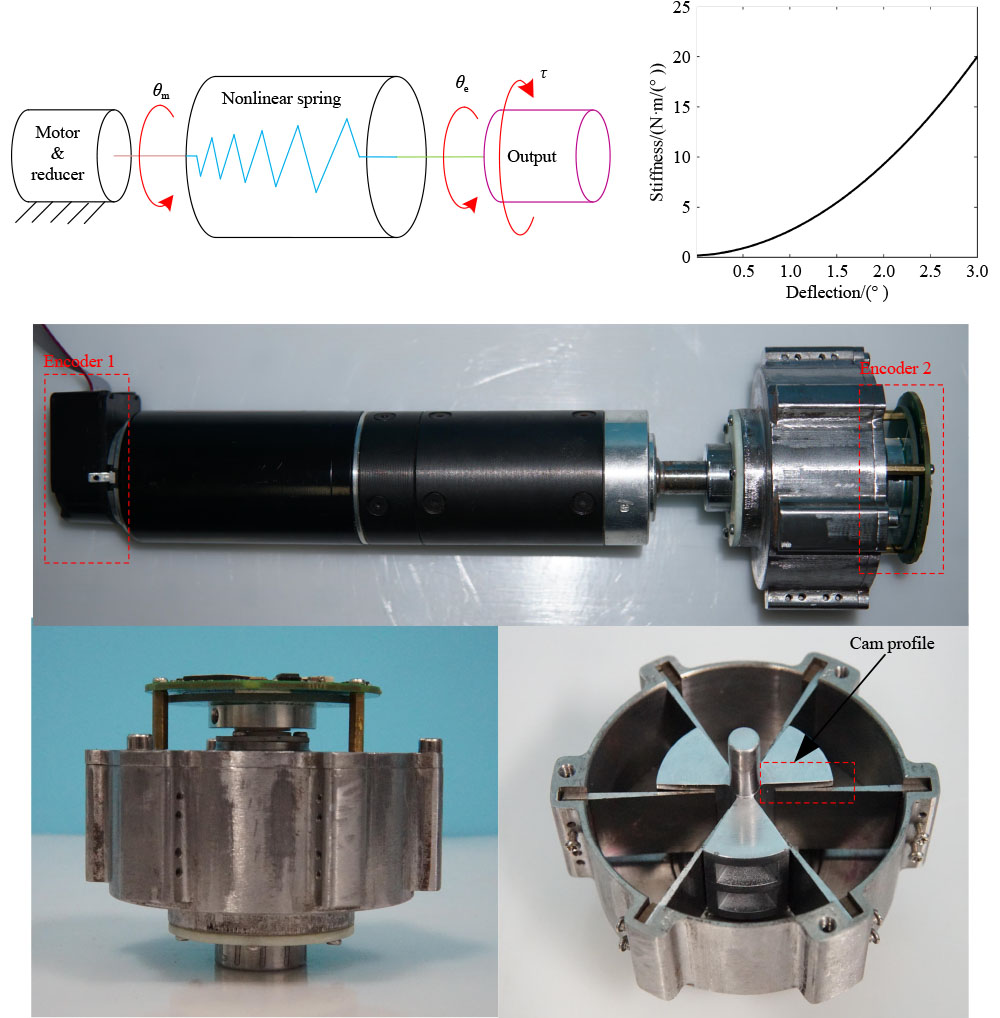
Wenjie JU, Hexi GONG, Keke QI, Rongjie KANG, Jian S. DAI, Zhibin SONG. New nonlinear stiffness actuator with predefined torque‒deflection profile[J]. Frontiers of Mechanical Engineering, 2023, 18(1): 5. DOI: 10.1007/s11465-022-0721-3
| 1 |
Pratt G A , Williamson M M . Series elastic actuators. In: Proceedings of 1995 IEEE/RSJ International Conference on Intelligent Robots and Systems. Human Robot Interaction and Cooperative Robots. Pittsburgh: IEEE, 1995,
|
| 2 |
Van Ham R , Vanderborght B , Van Damme M , Verrelst B , Lefeber D . MACCEPA, the mechanically adjustable compliance and controllable equilibrium position actuator: design and implementation in a biped robot. Robotics and Autonomous Systems, 2007, 55(10): 761–768
|
| 3 |
Deboon B , Nokleby S , Delfa N L , Rossa C . Differentially-clutched series elastic actuator for robot-aided musculoskeletal rehabilitation. In: Proceedings of 2019 International Conference on Robotics and Automation (ICRA). Montreal: IEEE, 2019,
|
| 4 |
Pfeifer S , Pagel A , Riener R , Vallery H . Actuator with angle-dependent elasticity for biomimetic transfemoral prostheses. IEEE/ASME Transactions on Mechatronics, 2015, 20(3): 1384–1394
|
| 5 |
Aguirre-Ollinger G , Yu H Y . Lower-limb exoskeleton with variable-structure series elastic actuators: phase-synchronized force control for gait asymmetry correction. IEEE Transactions on Robotics, 2021, 37(3): 763–779
|
| 6 |
Van Ham R , Sugar T G , Vanderborght B , Hollander K W , Lefeber D . Compliant actuator designs. IEEE Robotics & Automation Magazine, 2009, 16(3): 81–94
|
| 7 |
Kong K , Bae J , Tomizuka M . A compact rotary series elastic actuator for human assistive systems. IEEE/ASME Transactions on Mechatronics, 2012, 17(2): 288–297
|
| 8 |
Paine N , Mehling J S , Holley J , Radford N A , Johnson J , Fok C L , Sentis L . Actuator control for the NASA-JSC Valkyrie humanoid robot: a decoupled dynamics approach for torque control of series elastic robots. Journal of Field Robotics, 2015, 32(3): 378–396
|
| 9 |
Zhang T , Huang H . Design and control of a series elastic actuator with clutch for hip exoskeleton for precise assistive magnitude and timing control and improved mechanical safety. IEEE/ASME Transactions on Mechatronics, 2019, 24(5): 2215–2226
|
| 10 |
Jung Y , Bae J . Torque control of a series elastic tendon-sheath actuation mechanism. IEEE/ASME Transactions on Mechatronics, 2020, 25(6): 2915–2926
|
| 11 |
Kwak J , Choi W , Lee C , Oh S . Gravity and impedance compensation of body weight support system driven by two series elastic actuators. IEEE/ASME Transactions on Mechatronics, 2021, 27(1): 190–201
|
| 12 |
Liu Y Y , Li Z J , Su H , Su C Y . Whole body control of an autonomous mobile manipulator using series elastic actuators. IEEE/ASME Transactions on Mechatronics, 2021, 26(2): 657–667
|
| 13 |
Pei Y N , Han T Y , Zallek C M , Liu T , Yang L J , Hsiao-Wecksler E T . Design and clinical validation of a robotic ankle-foot simulator with series elastic actuator for ankle clonus assessment training. IEEE Robotics and Automation Letters, 2021, 6(2): 3793–3800
|
| 14 |
Robinson D W . Design and analysis of series elasticity in closed-loop actuator force control. Dissertation for the Doctoral Degree. Cambridge: Massachusetts Institute of Technology, 2000,
|
| 15 |
Carpino G , Accoto D , Sergi F , Luigi Tagliamonte N L , Guglielmelli E . A novel compact torsional spring for series elastic actuators for assistive wearable robots. Journal of Mechanical Design, 2012, 134(12): 121002
|
| 16 |
Paine N , Oh S , Sentis L . Design and control considerations for high-performance series elastic actuators. IEEE/ASME Transactions on Mechatronics, 2014, 19(3): 1080–1091
|
| 17 |
Tsagarakis N G , Sardellitti I , Caldwell D G . A new variable stiffness actuator (CompAct-VSA): design and modelling. In: Proceedings of 2011 IEEE/RSJ International Conference on Intelligent Robots and Systems. San Francisco: IEEE, 2011,
|
| 18 |
Wolf S , Eiberger O , Hirzinger G . The DLR FSJ: energy based design of a variable stiffness joint. In: Proceedings of 2011 IEEE International Conference on Robotics and Automation. Shanghai: IEEE, 2011,
|
| 19 |
Choi J , Hong S , Lee W , Kang S , Kim M . A robot joint with variable stiffness using leaf springs. IEEE Transactions on Robotics, 2011, 27(2): 229–238
|
| 20 |
Jafari A , Tsagarakis N G , Caldwell D G . A novel intrinsically energy efficient actuator with adjustable stiffness (AwAS). IEEE/ASME Transactions on Mechatronics, 2013, 18(1): 355–365
|
| 21 |
Liu Y W , Cui S P , Sun Y J . Mechanical design and analysis of a novel variable stiffness actuator with symmetrical pivot adjustment. Frontiers of Mechanical Engineering, 2021, 16(4): 711–725
|
| 22 |
Sun J T , Guo Z , Sun D Y , He S Y , Xiao X H . Design, modeling and control of a novel compact, energy-efficient, and rotational serial variable stiffness actuator (SVSA-II). Mechanism and Machine Theory, 2018, 130: 123–136
|
| 23 |
Wu J H , Wang Z R , Chen W , Wang Y Q , Liu Y H . Design and validation of a novel leaf spring-based variable stiffness joint with reconfigurability. IEEE/ASME Transactions on Mechatronics, 2020, 25(4): 2045–2053
|
| 24 |
Zhu Y H , Wu Q C , Chen B , Xu D W , Shao Z Y . Design and evaluation of a novel torque-controllable variable stiffness actuator with reconfigurability. IEEE/ASME Transactions on Mechatronics, 2021, 27(1): 292–303
|
| 25 |
Xu Y P , Guo K , Sun J , Li J F . Design, modeling and control of a reconfigurable variable stiffness actuator. Mechanical Systems and Signal Processing, 2021, 160: 107883
|
| 26 |
Sun Y X , Tang P , Dong D B , Zheng J , Chen X H , Bai L . Modeling and experimental evaluation of a pneumatic variable stiffness actuator. IEEE/ASME Transactions on Mechatronics, 2022, 27(5): 2462–2473
|
| 27 |
Mengacci R , Garabini M , Grioli G , Catalano M G , Bicchi A . Overcoming the torque/stiffness range tradeoff in antagonistic variable stiffness actuators. IEEE/ASME Transactions on Mechatronics, 2021, 26(6): 3186–3197
|
| 28 |
Li X , Zhu H Y , Lin W , Chen W J , Low K H . Structure-controlled variable stiffness robotic joint based on multiple rotary flexure hinges. IEEE Transactions on Industrial Electronics, 2021, 68(12): 12452–12461
|
| 29 |
Yang S K , Chen P , Wang D Q , Yu Y , Liu Y W . Design and analysis of a 2-DOF actuator with variable stiffness based on leaf springs. Journal of Bionic Engineering, 2022, 19: 1392–1404
|
| 30 |
Liu L , Misgeld B J E , Pomprapa A , Leonhardt S . A testable robust stability framework for the variable impedance control of 1-DOF exoskeleton with variable stiffness actuator. IEEE Transactions on Control Systems Technology, 2021, 29(6): 2728–2737
|
| 31 |
Koganezawa K, Watanabe Y, Shimizu N. Antagonistic muscle-like actuator and its application to multi-d.o.f. forearm prosthesis. Advanced Robotics, 1997, 12(7–8): 771–789
|
| 32 |
Hollander K W , Sugar T G , Herring D E . Adjustable robotic tendon using a ‘Jack Spring’/spl trade/. In: Proceedings of the 9th International Conference on Rehabilitation Robotics. Chicago: IEEE, 2005,
|
| 33 |
Hutter M , David C D , Siegwart R . Design of an articulated robotic leg with nonlinear series elastic actuation. In: Tosun O, Akin H L, Tokhi M O, Virk G S, eds. Mobile Robotics: Solutions and Challenges. New Jersey: World Scientific, 2009,
|
| 34 |
Thorson I , Caldwell D . A nonlinear series elastic actuator for highly dynamic motions. In: Proceedings of 2011 IEEE/RSJ International Conference on Intelligent Robots and Systems. San Francisco: IEEE, 2011,
|
| 35 |
Chen W H , Zhou L B , Wang J H , Zhao Z , Chen W J , Bai S P . A Maxwell-slip based hysteresis model for nonlinear stiffness compliant actuators. IEEE Transactions on Industrial Electronics, 2021, 69(11): 11510–11520
|
| 36 |
Rodríguez A G , Chacón J M , Donoso A , González Rodríguez A G . Design of an adjustable-stiffness spring: mathematical modeling and simulation, fabrication and experimental validation. Mechanism and Machine Theory, 2011, 46(12): 1970–1979
|
| 37 |
Li B K , Hao G B . Nonlinear behaviour design using the kinematic singularity of a general type of double-slider four-bar linkage. Mechanism and Machine Theory, 2018, 129: 106–130
|
| 38 |
Liu Y W , Wang D Q , Yang S K , Liu J G , Hao G B . Design and experimental study of a passive power-source-free stiffness-self-adjustable mechanism. Frontiers of Mechanical Engineering, 2021, 16(1): 32–45
|
| 39 |
Li Z Y , Bai S P , Chen W H , Zhang J B . Nonlinear stiffness analysis of spring-loaded inverted slider crank mechanisms with a unified model. Journal of Mechanisms and Robotics, 2020, 12(3): 031011
|
| 40 |
Migliore S A , Brown E A , DeWeerth S P . Biologically inspired joint stiffness control. In: Proceedings of the 2005 IEEE International Conference on Robotics and Automation. Barcelona: IEEE, 2005,
|
| 41 |
Shao Y X , Zhang W X , Su Y J , Ding X L . Design and optimisation of load-adaptive actuator with variable stiffness for compact ankle exoskeleton. Mechanism and Machine Theory, 2021, 161: 104323
|
| 42 |
Hao G B . A framework of designing compliant mechanisms with nonlinear stiffness characteristics. Microsystem Technologies, 2018, 24(4): 1795–1802
|
| 43 |
Palli G , Berselli G , Melchiorri C , Vassura G . Design of a variable stiffness actuator based on flexures. Journal of Mechanisms and Robotics, 2011, 3(3): 034501
|
| 44 |
Radaelli G , Herder J L . Shape optimization and sensitivity of compliant beams for prescribed load-displacement response. Mechanical Sciences, 2016, 7(2): 219–232
|
| 45 |
Schepelmann A , Geberth K A , Geyer H . Compact nonlinear springs with user defined torque-deflection profiles for series elastic actuators. In: Proceedings of 2014 IEEE International Conference on Robotics and Automation (ICRA). Hong Kong: IEEE, 2014,
|
| 46 |
Kuo P H , Deshpande A D . A novel joint design for robotic hands with humanlike nonlinear compliance. Journal of Mechanisms and Robotics, 2016, 8(2): 021004
|
| 47 |
Bi S S , Qiao T , Zhao H Z , Yu J J . Stiffness analysis of two compliant pivots used in series elastic actuators. Transactions of the Canadian Society for Mechanical Engineering, 2012, 36(3): 315–328
|
| 48 |
Kuo P H , Deshpande A D . Muscle-tendon units provide limited contributions to the passive stiffness of the index finger metacarpophalangeal joint. Journal of Biomechanics, 2012, 45(15): 2531–2538
|
| 49 |
Lan S B , Song Z B . Design of a new nonlinear stiffness compliant actuator and its error compensation method. Journal of Robotics, 2016, 2016: 7326905
|
| 50 |
Zhao Y R , Song Z B , Ma T Y , Dai J S . Optimisation of stiffness to achieve increased bandwidth and torque resolution in nonlinear stiffness actuators. IEEE Transactions on Industrial Electronics, 2020, 67(4): 2925–2935
|
| 51 |
Song Z B , Lan S B , Dai J S . A new mechanical design method of compliant actuators with non-linear stiffness with predefined deflection-torque profiles. Mechanism and Machine Theory, 2019, 133: 164–178
|
| 52 |
Hu X Q , Song Z B , Ma T Y . Novel design method for nonlinear stiffness actuator with user-defined deflection-torque profiles. Mechanism and Machine Theory, 2020, 146: 103712
|
| 53 |
Qi K K , Song Z B , Dai J S . Safe physical human-robot interaction: a quasi whole-body sensing method based on novel laser-ranging sensor ring pairs. Robotics and Computer-Integrated Manufacturing, 2022, 75: 102280
|
/
| 〈 |
|
〉 |