Deviation correction strategy for the earth pressure balance shield based on shield–soil interactions

Abstract

The control system presently used in shield posture rectification is based on driver experience, which is marginally reliable. The study of the related theory is flawed. Therefore, a decision-making approach for the deviation correction trajectory and posture rectification load for an earth pressure balance (EPB) shield is proposed. A calculation model of posture rectification load of an EPB shield is developed by considering the interactions among the cutter head, shield shell, and ground. The additional position change during the shield attitude correction is highlighted. The posture rectification loads and shield behaviors results can be solved by the proposed method. The influences of the stratum distribution (i.e., bedrock height in the upper-soft and lower-hard strata) on shield behaviors and posture rectification loads are analyzed. Results indicated that the increase of pitch angle in the upper-soft and lower-hard strata causes a sharp rise in vertical displacement. The bedrock height increases the magnitudes of the required posture rectification moments when h_r/D > 0.5. For a tunnel with h_r/D ≤ 0.5, the variation of h_r/D has little effect on the posture rectification moments. Finally, the posture rectifying curves based on the theoretical model are compared with the target ones based on the double circular arc interpolation method. The required results can be obtained regardless of the soil–rock compound stratum distribution. The maximum rectification moment in the rock layer is almost 12.6 times that in the soil layer. Overall, this study provides a valuable reference for moment determination and the trajectory prediction of posture rectification in compound strata.

Key words： additional position change; deviation correction trajectory; earth pressure balance shield; mechanical model; posture rectification

Nomenclature

ae	Gradient of the ground reaction curve
c	Soil cohesive force
C	A dimensionless parameter of CSM model
d	Wedge amount of the segment
D	Shield diameter
F1	Shield gravity
F2	Reaction force of the tail brush on the inner shield surface
F3	Thrust from jacks
F4	Force acting on the cutter head
F5	Force acting on the shield shell
Fn	Normal resistance forces of a single disc cutter
FM1,FM2	Moments induced by the penetration resistance and earth pressure, respectively
FM21, FM22	Moments caused by the later pressure of soil on the cutter head and rock on the cutter head, respectively
G	Gravity of a shield machine
h	Buried depth of the rock–soil interface
hr	Height of the bedrock in the tunnel face
H	Buried depth of the tunnel center
k	Number of segments
ke	Coefficient of the subgrade reaction
Ke,ij	Coefficient of earth pressure for an element
Ke0, Ke,max,Ke,min	Initial, passive, and active earth pressure coefficients, respectively
l	Segment length
ls	Gravity eccentric distance
L	Shield length
Ldev	Deviation correction mileage
m, n	Numbers of elements along the length and the ring direction of shield, respectively
M1v	Moment with respect to the shield center induced by the eccentric gravity
M4v	Moment with respect to the shield center on the vertical plane caused by the force acting on the cutter head
M5h	Moment with respect to the shield center on the horizontal plane induced by pressure on the shield shell
M5v	Moment with respect to the shield center on the vertical plane induced by pressure on the shield shell
M_{\mathrm{h}}^{\alpha }, M_{\mathrm{v}}^{\beta }	Moments for posture rectification in the horizontal and vertical plane generated by the thrust, respectively
nr	Number of disc cutters
p	Penetration per revolution
P0	Pressure of the broken zone
R	Disc cutter radius
Rc	Radius of the shield shell
Rrec	Radius of deviation correction curve
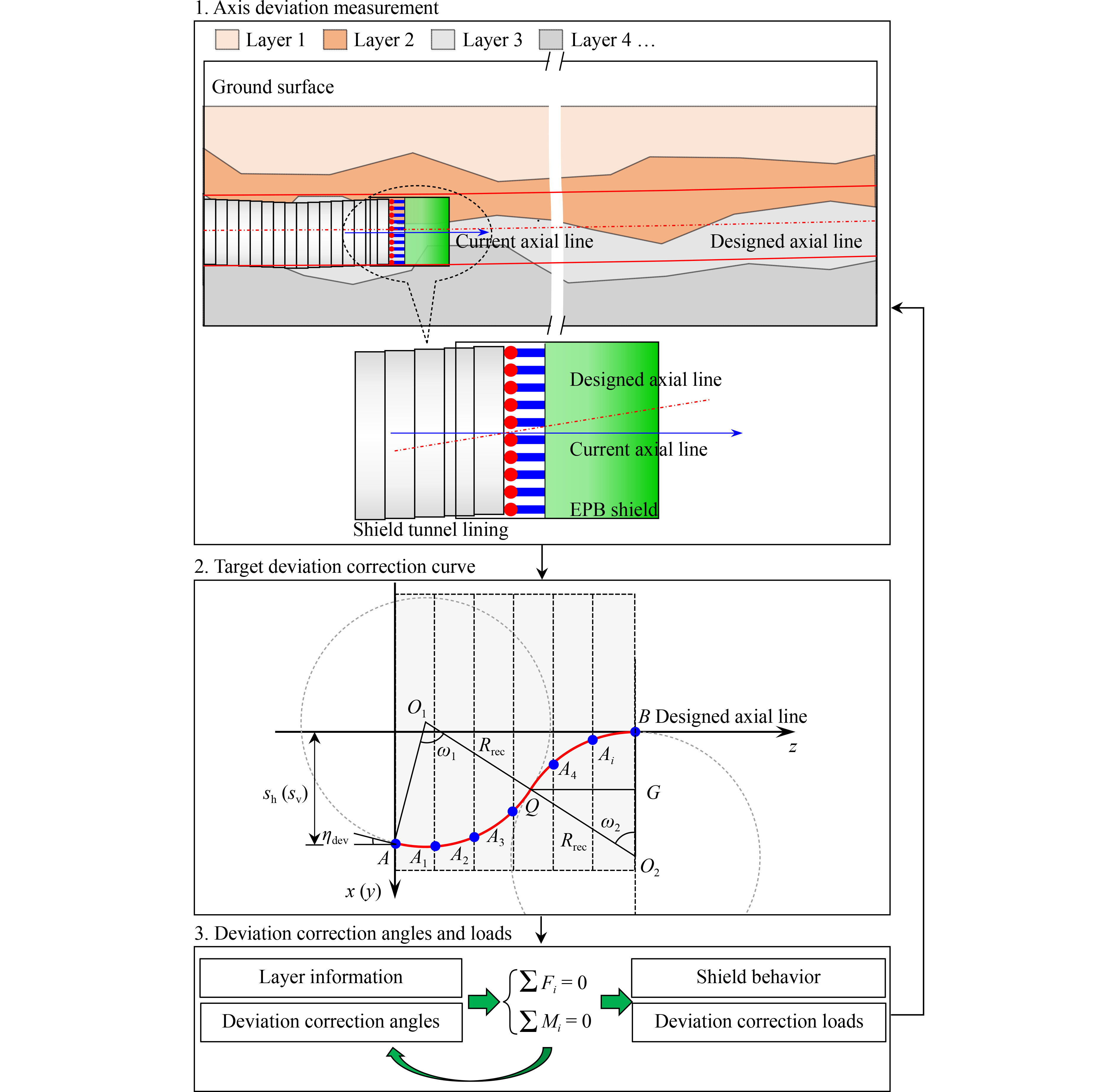
se	Axis deviation in the horizontal (e = h) or the vertical (e = v) direction
Δs	Vertical subsidence of the circle center induced by the shield gravity
Δsv, Δsh	Vertical and horizontal displacements of the circle center, respectively
S	Spacing between disc cutters
t	Tip width of a disc cutter
Ue,ij	Ground displacement of each element
Δyi	Target deviation correction amount at the ring (i‒1)
zij	Position on the shell of each element
α	Yawing angle
β	Pitch angle
γ1, γ2	Unit weights of soil and rock, respectively
δh, δv	Horizontal and vertical displacements of the circle center caused by posture rectification, respectivley
η	Opening ratio of cutterhend
ηdev	The angle of the shield and positive z-axis
ηi‒1	Angle of the shield down from the z-axis at the deviation correction segment (i‒1)
λ1, λ2	Later pressure coefficients of soil and rock, respectively
σc	Uniaxial compressive strength
σe0,ij	Initial pressure of each element
σh,ij, σv,ij	Horizontal and vertical pressures of each element, respectively
σn	Normal pressure on the shield shell
σt	Tensile strength
φ	Internal friction angle of soil
Φ	Contact angle between the rock and disc cutter
ψ	A model parameter of CSM model
{\omega }_1	Central angle of the first circular arc with a center of O1
{\omega }_2	Central angle of the second circular arc with a center of O2

Acknowledgements

This work was supported by the National Key R&D Program of China (Grant No. 2018YFC1505304), the National Major Scientific Instruments Development Project of China (Grant No. 41627801), the State Key Program of National Natural Science Foundation of China (Grant No. 41731288), and the Open Research Fund Program of State Key Laboratory of Permafrost Engineering of China (Grant No. SKLFSE202015).

References

1 Liu X Y , Shao C , Ma H F , Liu R X . Optimal earth pressure balance control for shield tunneling based on LS-SVM and PSO. Automation in Construction, 2011, 20( 4): 321– 327

2 Tang X Q , Deng K S , Wang L P , Chen X . Research on natural frequency characteristics of thrust system for EPB machines. Automation in Construction, 2012, 22 : 491– 497

3 Shao C , Lan D S . Optimal control of an earth pressure balance shield with tunnel face stability. Automation in Construction, 2014, 46 : 22– 29

4 Li X G , Yuan D J . Development of the safety control framework for shield tunneling in close proximity to the operational subway tunnels. SpringerPlus, 2016, 5( 1): 527

5 Lin S S , Shen S L , Zhang N , Zhou A . Modelling the performance of EPB shield tunnelling using machine and deep learning algorithms. Geoscience Frontiers, 2021, 12( 5): 101177

6 Zhang P , Chen R P , Wu H N . Real-time analysis and regulation of EPB shield steering using random forest. Automation in Construction, 2019, 106 : 102860

7 Koopialipoor M , Nikouei S S , Marto A , Fahimifar A , Jahed Armaghani D , Mohamad E T . Predicting tunnel boring machine performance through a new model based on the group method of data handling. Bulletin of Engineering Geology and the Environment, 2019, 78( 5): 3799– 3813

8 Elbaz K , Shen S L , Sun W J , Yin Z Y , Zhou A . Prediction model of shield performance during tunnelling via incorporating improved particle swarm optimization into ANFIS. IEEE Access: Practical Innovations, Open Solutions, 2020, 8 : 39659– 39671

9 Elbaz K , Shen S L , Zhou A , Yin Z Y , Lyu H M . Prediction of disc cutter life during shield tunneling with AI via the incorporation of a genetic algorithm into a GMDH-type neural network. Engineering, 2021, 7( 2): 238– 251

10 Yan T , Shen S L , Zhou A , Lyu H M . Construction efficiency of shield tunnelling through soft deposit in Tianjin, China. Tunnelling and Underground Space Technology, 2021, 112 : 103917

11 Yang J P , Chen W Z , Zhao W S , Tan X J , Tian H M , Yang D S , Ma C S . Geohazards of tunnel excavation in interbedded layers under high in situ stress. Engineering Geology, 2017, 230 : 11– 22

12 Berthoz N , Branque D , Wong H , Subrin D . TBM soft ground interaction: experimental study on a 1 g reduced-scale EPBS model. Tunnelling and Underground Space Technology, 2018, 72 : 189– 209

13 Baghban Golpasand M R , Do N A , Dias D . Impact of pre-existent Qanats on ground settlements due to mechanized tunneling. Transportation Geotechnics, 2019, 21 : 100262

14 Moeinossadat S R , Ahangari K . Estimating maximum surface settlement due to EPBM tunneling by numerical-intelligent approach―a case study: Tehran subway line 7. Transportation Geotechnics, 2019, 18 : 92– 102

15 Zheng G , Tong J B , Zhang T Q , Wang R K , Fan Q , Sun J B , Diao Y . Experimental study on surface settlements induced by sequential excavation of two parallel tunnels in drained granular soil. Tunnelling and Underground Space Technology, 2020, 98 : 103347

16 Liu Z Y , Xue J F , Ye J Z , Qian J G . A simplified two-stage method to estimate the settlement and bending moment of upper tunnel considering the interaction of undercrossing twin tunnels. Transportation Geotechnics, 2021, 29 : 100558

17 Shirlaw J N . Pressurised TBM tunnelling in mixed face conditions resulting from tropical weathering of igneous rock. Tunnelling and Underground Space Technology, 2016, 57 : 225– 240

18 Labra C , Rojek J , Oñate E . Discrete/finite element modelling of rock cutting with a TBM disc cutter. Rock Mechanics and Rock Engineering, 2017, 50( 3): 621– 638

19 Smolnicki T , Pękalski G , Jakubik J , Harnatkiewicz P . Investigation into wear mechanisms of the bearing raceway used in bucket wheel excavators. Archives of Civil and Mechanical Engineering, 2017, 17( 1): 1– 8

20 Ghorbani S , Kopilov V V , Polushin N I , Rogov V A . Experimental and analytical research on relationship between tool life and vibration in cutting process. Archives of Civil and Mechanical Engineering, 2018, 18( 3): 844– 862

21 Macias F J , Nærland J , Espallargas N . Cutter wear mechanisms in hard rock tunnel boring. Geomechanics and Tunnelling, 2018, 11( 2): 123– 130

22 Tóth Á , Gong Q M , Zhao J . Case studies of TBM tunneling performance in rock–soil interface mixed ground. Tunnelling and Underground Space Technology, 2013, 38 : 140– 150

23 Ma H , Yin L , Gong Q , Wang J . TBM tunneling in mixed-face ground: problems and solutions. International Journal of Mining Science and Technology, 2015, 25( 4): 641– 647

24 Wang L T , Yang X , Gong G F , Du J . Pose and trajectory control of shield tunneling machine in complicated stratum. Automation in Construction, 2018, 93 : 192– 199

25 Zhou C , Xu H C , Ding L Y , Wei L C , Zhou Y . Dynamic prediction for attitude and position in shield tunneling: a deep learning method. Automation in Construction, 2019, 105 : 102840

26 Mo H H , Chen J S . Study on inner force and dislocation of segments caused by shield machine attitude. Tunnelling and Underground Space Technology, 2008, 23( 3): 281– 291

27 Liu W , Zhao T S , Zhou W , Tang J J . Safety risk factors of metro tunnel construction in China: an integrated study with EFA and SEM. Safety Science, 2018, 105 : 98– 113

28 Zhang N Zhang N Zheng Q Xu Y S. Real-time prediction of shield moving trajectory during tunnelling using GRU deep neural network. Acta Geotechnica, 2021

29 Zhu B D , Gong G F , Shi H . Electrohydraulic control of thrust hydraulic system for shield tunneling machine. In: Xie M, Xiong Y, Xiong C, Liu H, Hu Z, eds. Intelligent Robotics and Applications. ICIRA 2009. Lecture Notes in Computer Science, vol 5928. Berlin: Springer, 2009, 482– 492

30 Luo L W Wang S C Wang X. Fuzzy control technique in tunneling process of shield machine. Machinery Design & Manufacture, 2010, 3: 125− 126 (in Chinese)

31 Feng H H Chen K Zhang B. Application and forecasting of fuzzy control technology in shield tunneling. Tunnel Construction, 2012, 32(S2): 51− 55 (in Chinese)

32 Hu X T , Huang Y A , Yin Z P , Xiong Y L . Driving force planning in shield tunneling based on Markov decision processes. Science China Technological Sciences, 2012, 55( 4): 1022– 1030

33 Yue M , Guo L . Double closed-loop adaptive rectification control of a shield tunneling machine with hydraulic actuator dynamics subject to saturation constraint. Journal of Vibration and Control, 2016, 22( 2): 309– 319

34 Wang P , Kong X G , Guo Z K , Hu L . Prediction of axis attitude deviation and deviation correction method based on data driven during shield tunneling. IEEE Access: Practical Innovations, Open Solutions, 2019, 7 : 163487– 163501

35 Sugimoto M , Sramoon A . Theoretical model of shield behavior during excavation. I: theory. Journal of Geotechnical and Geoenvironmental Engineering, 2002, 128( 2): 138– 155

36 Sugimoto M , Sramoon A , Konishi S , Sato Y . Simulation of shield tunneling behavior along a curved alignment in a multilayered ground. Journal of Geotechnical and Geoenvironmental Engineering, 2007, 133( 6): 684– 694

37 Shen S L , Du Y J , Luo C Y . Evaluation of the effect of rolling correction of double-o-tunnel shields via one-side loading. Canadian Geotechnical Journal, 2010, 47( 10): 1060– 1070

38 Hu X T , Huang Y A , Yin Z P , Xiong Y L . Optimization-based model of tunneling-induced distributed loads acting on the shield periphery. Automation in Construction, 2012, 24 : 138– 148

39 Festa D , Broere W , Bosch J W . Kinematic behaviour of a tunnel boring machine in soft soil: theory and observations. Tunnelling and Underground Space Technology, 2015, 49 : 208– 217

40 Festa D , Broere W , Bosch J W . An investigation into the forces acting on a TBM during driving–mining the TBM logged data. Tunnelling and Underground Space Technology, 2012, 32 : 143– 157

41 Festa D , Broere W , Bosch J . Tunnelling in soft soil: tunnel boring machine operation and soil response. In: Proceedings of International Symposium on Tunnelling and Underground Space Construction for Sustainable Development. Seoul, 2013, 117– 120

42 Shen X , Yuan D J , Jin D L . Influence of shield attitude change on shield–soil interaction. Applied Sciences, 2019, 9( 9): 1812

43 Zhang Z Q Yan Q S Zheng Z D Chen S B. Error estimate of approximate double circular arc interpolated method for curve smoothing. Materials Science Forum, 2004, 471–472: 92– 95

44 Rostami J. Development of a force estimation model for rock fragmentation with disc cutters through theoretical modeling and physical measurement of crushed zone pressure. Dissertation for the Doctoral Degree. Golden: Colorado School of Mines, 1997

45 Sramoon A , Sugimoto M . Development of a ground reaction curve for shield tunneling. In: Proceedings of International Symposium Geotechnical Aspects of Underground Construction in Soft Ground. Rotterdam, 1999, 437– 442

46 Terzaghi K . Stress distribution in dry and in saturated sand above a yielding trap-door. In: Proceedings of the 1st International Conference on Soil Mechanics and Foundation Engineering. Cambridge, 1936, 1 : 307– 311