

Frontiers of Mechanical Engineering >
High-precision gyro-stabilized control of a gear-driven platform with a floating gear tension device
Received date: 19 Oct 2020
Accepted date: 20 Feb 2021
Published date: 15 Sep 2021
Copyright
This study presents an improved compound control algorithm that substantially enhances the anti-disturbance performance of a gear-drive gyro-stabilized platform with a floating gear tension device. The tension device can provide a self-adjustable preload to eliminate the gap in the meshing process. However, the weaker gear support stiffness and more complex meshing friction are also induced by the tension device, which deteriorates the control accuracy and the ability to keep the aim point of the optical sensors isolated from the platform motion. The modeling and compensation of the induced complex nonlinearities are technically challenging, especially when base motion exists. The aim of this research is to cope with the unmeasured disturbances as well as the uncertainties caused by the base lateral motion. First, the structural properties of the gear transmission and the friction-generating mechanism are analyzed, which classify the disturbances into two categories: Time-invariant and time-varying parts. Then, a proportional-integral controller is designed to eliminate the steady-state error caused by the time-invariant disturbance. A proportional multiple-integral-based state augmented Kalman filter is proposed to estimate and compensate for the time-varying disturbance that can be approximated as a polynomial function. The effectiveness of the proposed compound algorithm is demonstrated by comparative experiments on a gear-drive pointing system with a floating gear tension device, which shows a maximum 76% improvement in stabilization precision.
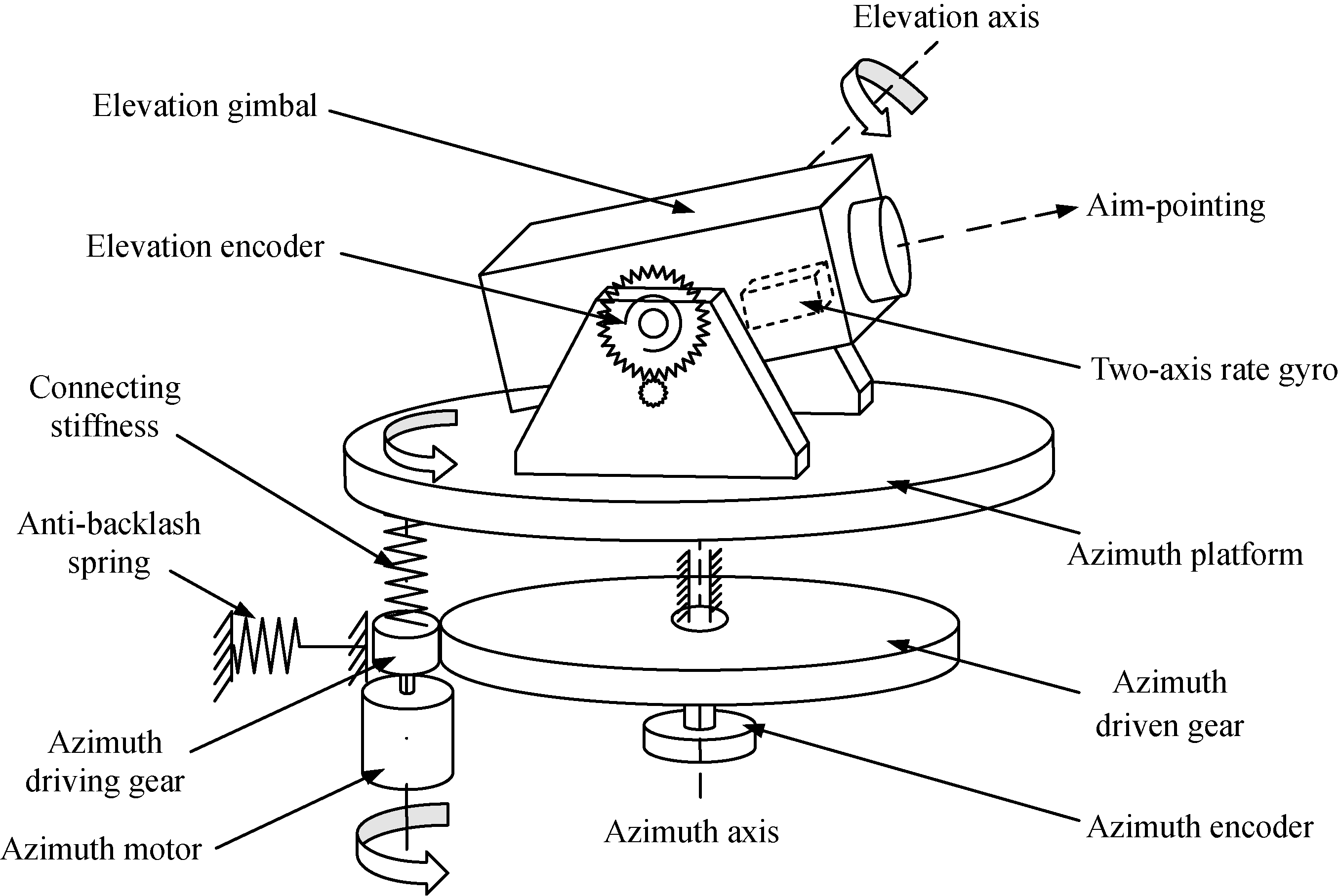
Xianliang JIANG , Dapeng FAN , Shixun FAN , Xin XIE , Ning CHEN . High-precision gyro-stabilized control of a gear-driven platform with a floating gear tension device[J]. Frontiers of Mechanical Engineering, 2021 , 16(3) : 487 -503 . DOI: 10.1007/s11465-021-0635-5
| 1 |
Yao J. Model-based nonlinear control of hydraulic servo systems: Challenges, developments and perspectives. Frontiers of Mechanical Engineering, 2018, 13(2): 179–210
|
| 2 |
Profeta J A, Vogt W G, Mickle M H. Torque disturbance rejection in high accuracy tracking systems. IEEE Transactions on Aerospace and Electronic Systems, 1990, 26(2): 232–287
|
| 3 |
Boscariol P, Gasparetto A. Vibration suppression of speed-controlled robots with nonlinear control. Frontiers of Mechanical Engineering, 2016, 11(2): 204–212
|
| 4 |
Xiao B, Yin S, Kaynak O. Attitude stabilization control of flexible satellites with high accuracy: An estimator-based approach. IEEE/ASME Transactions on Mechatronics, 2017, 22(1): 349–358
|
| 5 |
Kim K H, Lee J K, Park B S,
|
| 6 |
Königseder F, Kemmetmüller W, Kugi A. Attitude control strategy for a camera stabilization platform. Mechatronics, 2017, 46: 60–69
|
| 7 |
Kennedy P J, Kennedy R L. Direct versus indirect line of sight (LOS) stabilization. IEEE Transactions on Control Systems Technology, 2003, 11(1): 3–15
|
| 8 |
Jiang L, Deng Z, Gu F,
|
| 9 |
Lin J, Parker R G. Mesh stiffness variation instabilities in two-stage gear systems. Journal of Vibration and Acoustics, 2002, 124(1): 68–76
|
| 10 |
Cai Y, Ren G, Song J,
|
| 11 |
Guo X, Zeng J, Ma H,
|
| 12 |
Li B, Hullender D, DiRenzo M. Nonlinear induced disturbance rejection in inertial stabilization systems. IEEE Transactions on Control Systems Technology, 1998, 6(3): 421–427
|
| 13 |
Zhou X, Shi Y, Li L,
|
| 14 |
Tsai S J, Huang G L, Ye S Y. Gear meshing analysis of planetary gear sets with a floating sun gear. Mechanism and Machine Theory, 2015, 84: 145–163
|
| 15 |
Magnani G, Rocco P. Mechatronic analysis of a complex transmission chain for performance optimization in a machine tool. Mechatronics, 2010, 20(1): 85–101
|
| 16 |
Li S, Zhong M. High-precision disturbance compensation for a three-axis gyro-stabilized camera mount. IEEE/ASME Transactions on Mechatronics, 2015, 20(6): 3135–3147
|
| 17 |
Li B, Hullender D A. Self-tuning controller for nonlinear inertial stabilization systems. Proceedings of the Society for Photo-Instrumentation Engineers, 1996, 2739(1): 229–241
|
| 18 |
Pisu P, Serrani A. Attitude tracking with adaptive rejection of rate gyro disturbances. IEEE Transactions on Automatic Control, 2007, 52(12): 2374–2379
|
| 19 |
Hong S, Cho K D. Kinematic algorithms and robust controller design for inertially stabilized system. IEEE/ASME Transactions on Mechatronics, 2014, 19(1): 76–87
|
| 20 |
Ahi B, Nobakhti A. Hardware implementation of an ADRC controller on a gimbal mechanism. IEEE Transactions on Control Systems Technology, 2018, 26(6): 2268–2275
|
| 21 |
Mao J, Yang J, Liu X,
|
| 22 |
Safa A, Yazdanpanah Abdolmalaki R. Robust output feedback tracking control for inertially stabilized platforms with matched and unmatched uncertainties. IEEE Transactions on Control Systems Technology, 2019, 27(1): 118–131
|
| 23 |
Ferguson J, Donaire A, Ortega R,
|
| 24 |
Koenig D, Mammar S. Design of proportional-integral observer for unknown input descriptor systems. IEEE Transactions on Automatic Control, 2002, 47(12): 2057–2062
|
| 25 |
Ren C, Ma S. Generalized proportional integral observer based control of an omnidirectional mobile robot. Mechatronics, 2015, 26: 36–44
|
| 26 |
Zhang S Q, Li H N, Schmidt R,
|
| 27 |
Lu J, Savaghebi M, Ghias A M Y M,
|
| 28 |
Boichuk O F. Vibrations of a stabilized platform in an inertial navigation system. Soviet Applied Mechanics, 1968, 4(9): 65–68
|
| 29 |
Wu M, Gao F, Yu P,
|
| 30 |
Gutman P, Velger M. Tracking targets using adaptive Kalman filtering. IEEE Transactions on Aerospace and Electronic Systems, 1990, 26(5): 691–699
|
| 31 |
Erkorkmaz K, Altintas Y. High speed CNC system design. Part III: High speed tracking and contouring control of feed drives. International Journal of Machine Tools and Manufacture, 2001, 41(11): 1637–1658
|
| 32 |
Sheikh Sofla M, Zareinejad M, Parsa M,
|
| 33 |
Zhou S, Song G, Sun M,
|
/
| 〈 |
|
〉 |