

Spin-controlled topological phase transition in non-Euclidean space
Received date: 04 Jan 2024
Accepted date: 27 Feb 2024
Published date: 15 Mar 2024
Copyright
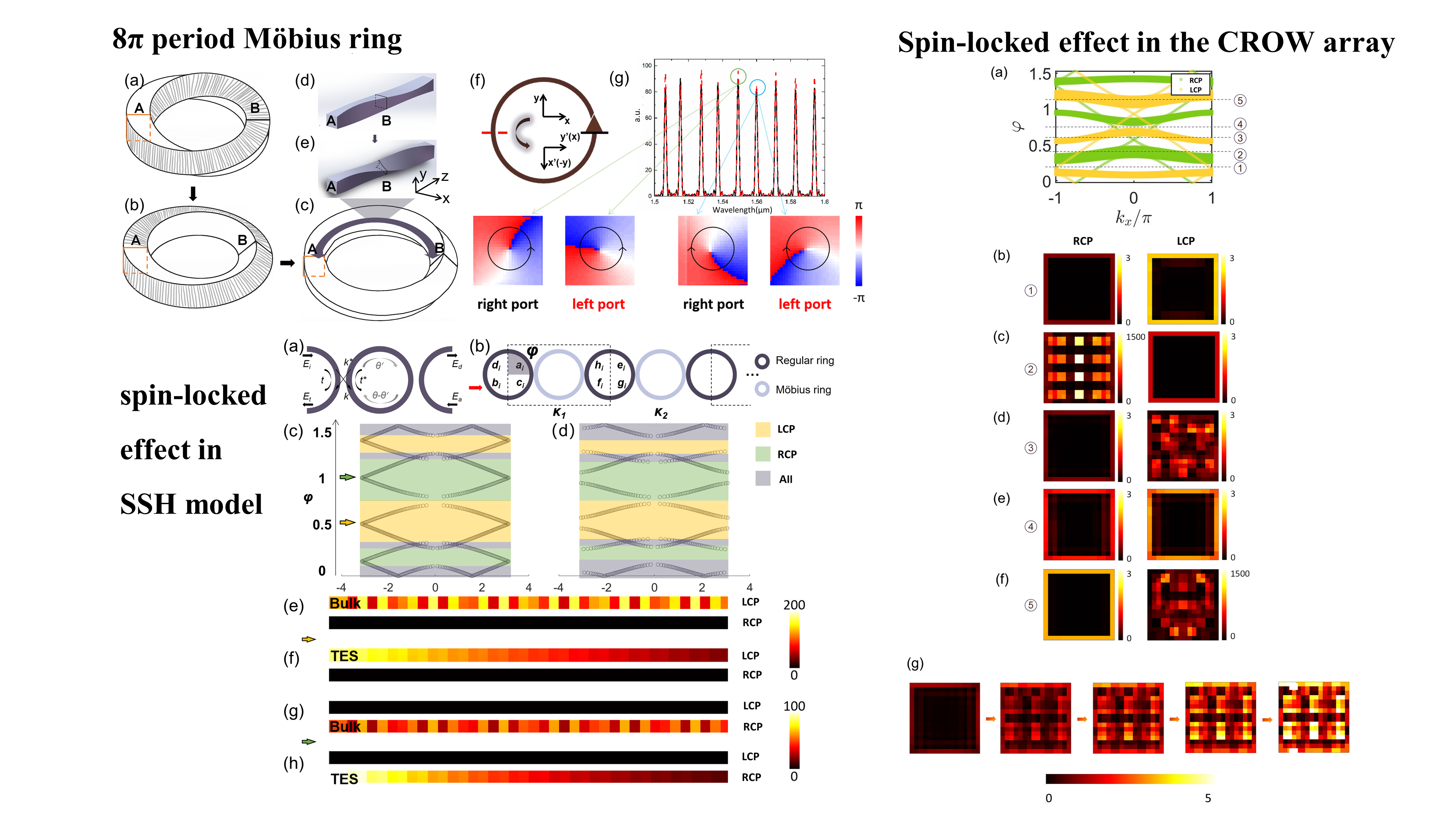
Modulation of topological phase transition has been pursued by researchers in both condensed matter and optics research fields, and has been realized in Euclidean systems, such as topological photonic crystals, topological metamaterials, and coupled resonator arrays. However, the spin-controlled topological phase transition in non-Euclidean space has not yet been explored. Here, we propose a non-Euclidean configuration based on Möbius rings, and we demonstrate the spin-controlled transition between the topological edge state and the bulk state. The Möbius ring, which is designed to have an 8π period, has a square cross section at the twist beginning and the length/width evolves adiabatically along the loop, accompanied by conversion from transverse electric to transverse magnetic modes resulting from the spin-locked effect. The 8π period Möbius rings are used to construct Su–Schrieffer–Heeger configuration, and the configuration can support the topological edge states excited by circularly polarized light, and meanwhile a transition from the topological edge state to the bulk state can be realized by controlling circular polarization. In addition, the spin-controlled topological phase transition in non-Euclidean space is feasible for both Hermitian and non-Hermitian cases in 2D systems. This work provides a new degree of polarization to control topological photonic states based on the spin of Möbius rings and opens a way to tune the topological phase in non-Euclidean space.
Zhuochen Du , Jinze Gao , Qiuchen Yan , Cuicui Lu , Xiaoyong Hu , Qihuang Gong . Spin-controlled topological phase transition in non-Euclidean space[J]. Frontiers of Optoelectronics, 2024 , 17(1) : 7 . DOI: 10.1007/s12200-024-00110-w
| 1 |
Ozawa, T., Price, H.M., Amo, A., Goldman, N., Hafezi, M., Lu, L., Rechtsman, M.C., Schuster, D., Simon, J., Zilberberg, O., Carusotto, I.: Topological photonics. Rev. Mod. Phys. 91(1), 015006 (2019)
|
| 2 |
Khanikaev, A.B., Shvets, G.: Two-dimensional topological photonics. Nat. Photonics 11(12), 763–773 (2017)
|
| 3 |
Smirnova, D., Leykam, D., Chong, Y.D., Kivshar, Y.: Nonlinear topological photonics. Appl. Phys. Rev. 7(2), 021306 (2020)
|
| 4 |
Yan, Q.C., Hu, X.Y., Fu, Y.L., Lu, C.C., Fan, C.X., Liu, Q.H., Feng, X.L., Sun, Q., Gong, Q.H.: Quantum topological photonics. Adv. Opt. Mater. 9(15), 2001739 (2021)
|
| 5 |
Ota, Y., Takata, K., Ozawa, T., Amo, A., Jia, Z., Kante, B., Notomi, M., Arakawa, Y., Iwamoto, S.: Active topological photonics. Nanophotonics 9(3), 547–567 (2020)
|
| 6 |
Kim, M., Jacob, Z., Rho, J.: Recent advances in 2D, 3D and higher-order topological photonics. Light Sci. Appl. 9, 130 (2020)
|
| 7 |
Lustig, E., Segev, M.: Topological photonics in synthetic dimensions. Adv. Opt. Photonics 13(2), 426 (2021)
|
| 8 |
Song, Y.L., Monceaux, Y., Bittner, S., Chao, K., Reynoso de la Cruz, H.M., Lafargue, C., Decanini, D., Dietz, B., Zyss, J., Grigis, A., Checoury, X., Lebental, M.: Möbius strip microlasers: a testbed for non-Euclidean photonics. Phys. Rev. Lett. 127(20), 203901 (2021)
|
| 9 |
Garcia-Etxarri, A.: Optical polarization Möbius strips on all-dielectric optical scatterers. ACS Photonics 4(5), 1159–1164 (2017)
|
| 10 |
Herges, R.: Topology in chemistry: designing Möbius molecules. Chem. Rev. 106(12), 4820–4842 (2006)
|
| 11 |
Starostin, E.L., Van der Heijden, G.H.M.: The shape of a Möbius strip. Nat. Mater. 6(8), 563–567 (2007)
|
| 12 |
Flouris, K., Jimenez, M.M., Herrmann, H.J.: Curvature-induced quantum spin-Hall effect on a Möbius strip. Phys. Rev. B 105(23), 235122 (2022)
|
| 13 |
Xu, X.B., Shi, L., Guo, G.C., Dong, C.H., Zou, C.L.: “Möbius” microring resonator. Appl. Phys. Lett. 114(10), 101106 (2019)
|
| 14 |
Kreismann, J., Hentschel, M.: The optical Möbius strip cavity: tailoring geometric phases and far fields. Europhys. Lett. 121(2), 24001 (2018)
|
| 15 |
Hamilton, J.K., Hooper, I.R., Lawrence, C.R.: Absorption modes of Möbius strip resonators. Sci. Rep. 11(1), 1–7 (2021)
|
| 16 |
Zeng, Y., Wang, Z.Y., Wu, Y., Lu, L.S., Wang, Y.X., Shi, S.J., Qiu, Q.: Plasmonic microcavity formed by the Möbius strip. Chin. Phys. B 26(3), 037303 (2017)
|
| 17 |
Nie, Z.Z., Zuo, B., Wang, M., Huang, S., Chen, X.M., Liu, Z.Y., Yang, H.: Light-driven continuous rotating Möbius strip actuators. Nat. Commun. 12, 2334 (2021)
|
| 18 |
Bogaerts, W., De Heyn, P., Van Vaerenbergh, T., De Vos, K., Kumar Selvaraja, S., Claes, T., Dumon, P., Bienstman, P., Van Thourhout, D., Baets, R.: Silicon microring resonators. Laser Photonics Rev. 6(1), 47–73 (2012)
|
| 19 |
Parto, M., Wittek, S., Hodaei, H., Harari, G., Bandres, M.A., Ren, J., Rechtsman, M.C., Segev, M., Christodoulides, D.N., Khajavikhan, M.: Edge-mode lasing in 1D topological active arrays. Phys. Rev. Lett. 120(11), 113901 (2018)
|
| 20 |
Takata, K., Notomi, M.: Photonic topological insulating phase induced solely by gain and loss. Phys. Rev. Lett. 121(21), 213902 (2018)
|
| 21 |
Yan, Q.C., Cao, E., Sun, Q., Ao, Y.T., Hu, X.Y., Shi, X., Gong, Q.H., Misawa, H.: Near-field imaging and time-domain dynamics of photonic topological edge states in plasmonic nanochains. Nano Lett. 21(21), 9270–9278 (2021)
|
| 22 |
Ao, Y.T., Hu, X.Y., You, Y.L., Lu, C.C., Fu, Y.L., Wang, X.Y., Gong, Q.H.: Topological phase transition in the non-Hermitian coupled resonator array. Phys. Rev. Lett. 125(1), 013902 (2020)
|
/
| 〈 |
|
〉 |