Towards an integrated modeling of the plasma-solid interface

Abstract

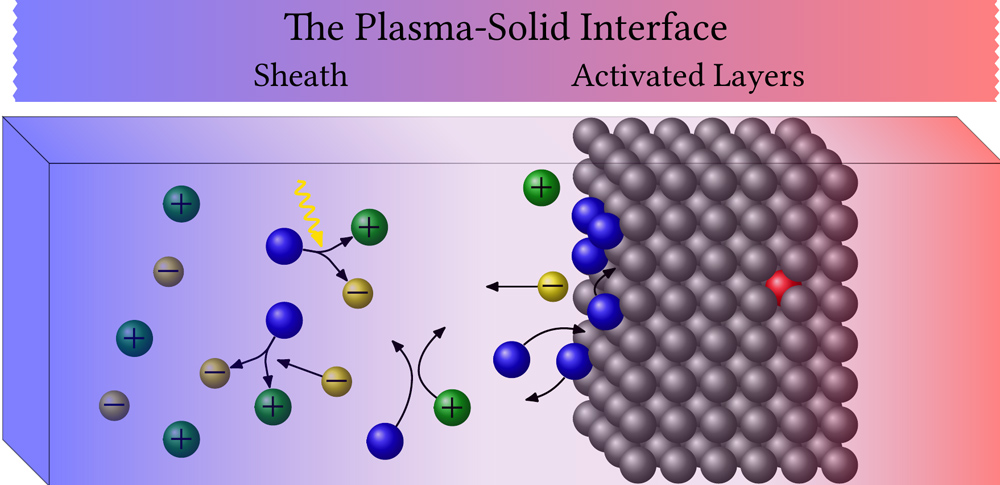
Solids facing a plasma are a common situation in many astrophysical systems and laboratory setups. Moreover, many plasma technology applications rely on the control of the plasma-surface interaction, i.e., of the particle, momentum and energy fluxes across the plasma-solid interface. However, presently often a fundamental understanding of them is missing, so most technological applications are being developed via trial and error. The reason is that the physical processes at the interface of a low-temperature plasma and a solid are extremely complex, involving a large number of elementary processes in the plasma, in the solid as well as fluxes across the interface. An accurate theoretical treatment of these processes is very difficult due to the vastly different system properties on both sides of the interface: Quantum versus classical behavior of electrons in the solid and plasma, respectively; as well as the dramatically differing electron densities, length and time scales. Moreover, often the system is far from equilibrium. In the majority of plasma simulations surface processes are either neglected or treated via phenomenological parameters such as sticking coefficients, sputter rates or secondary electron emission coefficients. However, those parameters are known only in some cases and with very limited accuracy. Similarly, while surface physics simulations have often studied the impact of single ions or neutrals, so far, the influence of a plasma medium and correlations between successive impacts have not been taken into account. Such an approach, necessarily neglects the mutual influences between plasma and solid surface and cannot have predictive power.

In this paper we discuss in some detail the physical processes of the plasma-solid interface which brings us to the necessity of coupled plasma-solid simulations. We briefly summarize relevant theoretical methods from solid state and surface physics that are suitable to contribute to such an approach and identify four methods. The first are mesoscopic simulations such as kinetic Monte Carlo and molecular dynamics that are able to treat complex processes on large scales but neglect electronic effects. The second are quantum kinetic methods based on the quantum Boltzmann equation that give access to a more accurate treatment of surface processes using simplifying models for the solid. The third approach are ab initio simulations of surface process that are based on density functional theory (DFT) and time-dependent DFT. The fourths are nonequilibrium Green functions that able to treat correlation effects in the material and at the interface. The price for the increased quality is a dramatic increase of computational effort and a restriction to short time and length scales. We conclude that, presently, none of the four methods is capable of providing a complete picture of the processes at the interface. Instead, each of them provides complementary information, and we discuss possible combinations.

Key words： plasma physics; surface science; plasma- surface modeling; DFT; nonequilibrium Green functions

Acknowledgements

The authors acknowledge many fruitful discussions with our colleagues in Kiel−in particular, M. Bauer, J. Benedikt, J. Golda, B. Hartke, H. Kersten, O. Magnussen, K. Rossnagel, J. Stettner, and T. Trottenberg—with whom the present concept has been developed. EP is grateful to L. Deuchler for insightful discussions concerning TDDFT-MD simulations. MB and KB are grateful to A. Marini and D. Sangalli for their support in using their AI-NEGF-code Yambo. MB is grateful to K. Becker, A. Bogaerts, P. Bruggeman, Z. Donko, J.G. Eden, U. Fantz, I. Kaganovich, M. Kushner, E. Neyts, G. Oehrlein, K. Ostrikov, and Y. Raitses for many stimulating remarks during presentation of early versions of this work.

References

1 Skiff F, Wurtele J. Plasma: At the Frontier of Science Discovery. Technical Report, U.S. Department of Energy, Office of Sciences, 2017

2 Meyyappan M. Plasma nanotechnology: Past, present and future. Journal of Physics. D, Applied Physics, 2011, 44(17): 174002

3 Ostrikov K, Neyts E C, Meyyappan M. Plasma nanoscience: From nano-solids in plasmas to nano-plasmas in solids. Advances in Physics, 2013, 62(2): 113–224

4 Son Y W, Cohen M L, Louie S G. Energy gaps in graphene nanoribbons. Physical Review Letters, 2006, 97(21): 216803

5 Prezzi D, Varsano D, Ruini A, Marini A, Molinari E. Optical properties of graphene nanoribbons: The role of many-body effects. Physical Review. B, 2008, 77(4): 041404

6 Adamovich I, Baalrud S D, Bogaerts A, Bruggeman P J, Cappelli M, Colombo V, Czarnetzki U, Ebert U, Eden J G, Favia P, . The 2017 plasma roadmap: Low temperature plasma science and technology. Journal of Physics. D, Applied Physics, 2017, 50(32): 323001

7 Hagelaar G J M, Pitchford L C. Solving the Boltzmann equation to obtain electron transport coefficients and rate coefficients for fluid models. Plasma Sources Science & Technology, 2005, 14(4): 722–733

8 Donko Z, Dyatko N. First-principles particle simulation and Boltzmann equation analysis of negative differential conductivity and transient negative mobility effects in xenon. European Physical Journal D, 2016, 70(6): 135

9 Teunissen J, Ebert U. 3D pic-mcc simulations of discharge inception around a sharp anode in nitrogen/oxygen mixtures. Plasma Sources Science & Technology, 2016, 25(4): 044005

10 Becker M M, Kählert H, Sun A, Bonitz M, Loffhagen D. Advanced fluid modeling and PIC/MCC simulations of low-pressure ccrf discharges. Plasma Sources Science & Technology, 2017, 26(4): 044001

11 Derzsi A, Korolov I, Schüngel E, Donkó Z, Schulze J. Effects of fast atoms and energy-dependent secondary electron emission yields in PIC/MCC simulations of capacitively coupled plasmas. Plasma Sources Science & Technology, 2015, 24(3): 034002

12 Phelps A V, Petrović Z L. Cold-cathode discharges and breakdown in argon: Surface and gas phase production of secondary electrons. Plasma Sources Science & Technology, 1999, 8(3): R21–R44

13 Brault P. Multiscale molecular dynamics simulation of plasma processing: Application to plasma sputtering. Frontiers in Physics, 2018, 6: 59

14 Zhao S, Kang W, Xue J, Zhang X, Zhang P. Comparison of electronic energy loss in graphene and BN sheet by means of time-dependent density functional theory. Journal of Physics Condensed Matter, 2015, 27(2): 025401

15 Balzer K, Schlünzen N, Bonitz M. Stopping dynamics of ions passing through correlated honeycomb clusters. Physical Review. B, 2016, 94(24): 245118

16 Graves D B, Brault P. Molecular dynamics for low temperature plasma—surface interaction studies. Journal of Physics. D, Applied Physics, 2009, 42(19): 194011

17 Neyts E C, Brault P. Molecular dynamics simulations for plasma-surface interactions. Plasma Processes and Polymers, 2017, 14(12): 1600145

18 Sheehan J P, Hershkowitz N, Kaganovich I D, Wang H, Raitses Y, Barnat E V, Weatherford B R, Sydorenko D. Kinetic theory of plasma sheaths surrounding electron-emitting surfaces. Physical Review Letters, 2013, 111(7): 075002

19 Bronold F X, Fehske H. Absorption of an electron by a dielectric wall. Physical Review Letters, 2015, 115(22): 225001

20 Sun A, Becker M M, Loffhagen D. PIC/MCC simulation of capacitively coupled discharges in helium: Boundary effects. Plasma Sources Science & Technology, 2018, 27(5): 054002

21 Li Y, Go D B. Using field emission to control the electron energy distribution in high-pressure microdischarges at microscale dimensions. Applied Physics Letters, 2013, 103(23): 234104

22 Helmholtz H. Ueber einige Gesetze der Vertheilung elektrischer Ströme in körperlichen Leitern mit Anwendung auf die thierischelektrischen Versuche. Annalen der Physik, 1853, 165(6): 211–233 (in German)

23 Heinisch R L, Bronold F X, Fehske H. Electron surface layer at the interface of a plasma and a dielectric wall. Physical Review. B, 2012, 85(7): 075323

24 Onida G, Reining L, Rubio A. Electronic excitations: Density-functional versus many-body green’s-function approaches. Reviews of Modern Physics, 2002, 74(2): 601–659

25 Kotliar G, Savrasov S Y, Haule K, Oudovenko V S, Parcollet O, Marianetti C A. Electronic structure calculations with dynamical mean-field theory. Reviews of Modern Physics, 2006, 78(3): 865–951

26 Foulkes W M C, Mitas L, Needs R J, Rajagopal G. Quantum monte carlo simulations of solids. Reviews of Modern Physics, 2001, 73(1): 33–83

27 Dornheim T, Groth S, Bonitz M. The uniform electron gas at warm dense matter conditions. Physics Reports, 2018, 744: 1–86

28 Abraham J W. Formation of metal-polymer nanocomposites by plasma-based deposition methods: Kinetic monte carlo and molecular dynamics simulations. Dissertation for the Doctoral Degree. Kiel: Christian-Albrechts-Universität zu Kiel, 2018

29 Daniil M, Carlos T, Vasco G. Deterministic and monte carlo methods for simulation of plasma-surface interactions. Plasma Processes and Polymers, 2016, 14(1-2): 1600175

30 Guerra V, Loureiro J. Dynamical monte carlo simulation of surface atomic recombination. Plasma Sources Science & Technology, 2004, 13(1): 85–94

31 Abraham J W, Kongsuwan N, Strunskus T, Faupel F, Bonitz M. Simulation of nanocolumn formation in a plasma environment. Journal of Applied Physics, 2015, 117(1): 014305

32 Fujioka K. Kinetic Monte Carlo simulations of cluster growth in magnetron plasmas. Dissertation for the Doctoral Degree. Kiel: Christian-Albrechts-Universität zu Kiel, 2015

33 Polonskyi O, Ahadi A M, Peter T, Fujioka K, Abraham J W, Vasiliauskaite E, Hinz A, Strunskus T, Wolf S, Bonitz M,et al.Plasma based formation and deposition of metal and metal oxide nanoparticles using a gas aggregation source. European Physical Journal D, 2018, 72(5): 93

34 Rosenthal L. Monte Carlo simulations of metal-polymer nanocomposite formation. Dissertation for the Doctoral Degree. Kiel: Christian-Albrechts-Universität zu Kiel, 2013

35 Runge E, Gross E K U. Density-functional theory for time-dependent systems. Physical Review Letters, 1984, 52(12): 997–1000

36 Balzer K, Bonitz M. Nonequilibrium Green’s Functions Approach to Inhomogeneous Systems. Berlin: Springer, 2013

37 Schlünzen N, Bonitz M. Nonequilibrium Green functions approach to strongly correlated fermions in lattice systems. Contributions to Plasma Physics, 2016, 56(1): 5–91

38 Marini A, Hogan C, Grüning M, Varsano D. Yambo: An ab initio tool for excited state calculations. Computer Physics Communications, 2009, 180(8): 1392–1403

39 Jürg H. Car—parrinello molecular dynamics. Wiley Interdisciplinary Reviews. Computational Molecular Science, 2012, 2(4): 604–612

40 Gross A. Theoretical Surface Science. 2nd ed. Berlin: Springer, 2009

41 Bonitz M, Lopez J, Becker K, Thomsen H, eds. Complex plasmas: Scientific Challenges and Technological Opportunities . Berlin: Springer, 2014

42 Ott T, Bonitz M. Diffusion in a strongly coupled magnetized plasma. Physical Review Letters, 2011, 107(13): 135003

43 Abraham J W, Strunskus T, Faupel F, Bonitz M. Molecular dynamics simulation of gold cluster growth during sputter deposition. Journal of Applied Physics, 2016, 119(18): 185301

44 Nakano A, Kalia R K, Nomura K, Sharma A, Vashishta P, Shimojo F, van Duin A C T, Goddard W A, Biswas R, Srivastava D, . De novo ultrascale atomistic simulations on high-end parallel supercomputers. International Journal of High Performance Computing Applications, 2008, 22(1): 113–128

45 Piana S, Lindorff-Larsen K, Shaw D E. Atomic-level description of ubiquitin folding. Proceedings of the National Academy of Sciences of the United States of America, 2013, 110(15): 5915–5920

46 Voter A F. Hyperdynamics: Accelerated molecular dynamics of infrequent events. Physical Review Letters, 1997, 78(20): 3908–3911

47 Laio A, Parrinello M. Escaping free-energy minima. Proceedings of the National Academy of Sciences of the United States of America, 2002, 99(20): 12562–12566

48 Sørensen M R, Voter A F. Temperature-accelerated dynamics for simulation of infrequent events. Journal of Chemical Physics, 2000, 112(21): 9599–9606

49 Bal K M, Neyts E C. Merging metadynamics into hyperdynamics: Accelerated molecular simulations reaching time scales from microseconds to seconds. Journal of Chemical Theory and Computation, 2015, 11(10): 4545–4554

50 Bonitz M, Filinov A, Abraham J W, Loffhagen D. Extending first principle plasma-surface simulations to experimentally relevant scales. Plasma Sources Science & Technology, 2018, 27(6): 064005

51 Abraham J W, Bonitz M. Molecular dynamics simulation of Ag–Cu cluster growth on a thin polymer film. Contributions to Plasma Physics, 2018, 58(2-3): 164–173

52 Franke A, Pehlke E. Diffusion of 1,4-butanedithiol on Au(100)(1x1): A DFT-based master-equation approach. Physical Review. B, 2010, 82(20): 205423

53 Filinov A, Bonitz M, Loffhagen D. Microscopic modeling of gas-surface scattering. I. A combined molecular dynamics-rate equation approach. Plasma Sources Science & Technology, 2018, 27(6): 064003

54 Schwartzkopf M, Santoro G, Brett C J, Rothkirch A, Polonskyi O, Hinz A, Metwalli E, Yao Y, Strunskus T, Faupel F, et al. Real-time monitoring of morphology and optical properties during sputter deposition for tailoring metal-polymer interfaces. ACS Applied Materials & Interfaces, 2015, 7(24): 13547–13556

55 Abraham J W, Hinz A, Strunskus T, Faupel F, Bonitz M. Formation of polymer-based nanoparticles and nanocomposites by plasma-assisted deposition methods. European Physical Journal D, 2018, 72(5): 92

56 Bonitz M. Quantum Kinetic Theory. 2nd ed. Berlin: Springer, 2016

57 Filinov A, Bonitz M, Loffhagen D. Microscopic modeling of gas-surface scattering: II. Application to argon atom adsorption on a platinum (111) surface. Plasma Sources Science & Technology, 2018, 27(6): 064002

58 Lieberman M A, Lichtenberg A J. Principles of Plasma Discharges and Materials Processing. New York: Wiley-Interscience, 2005

59 Rabalais J W. Low Energy Ion-surface Interaction. New York: Wiley and Sons, 1994

60 Winter H. Collision of atoms and ions with surfaces under grazing incidence. Physics Reports, 2002, 367(5): 387–582

61 Winter H P, Burgdörfer J. Slow Heavy-particle Induced Electron Emission from Solid Surfaces. Berlin: Springer, 2007

62 Daksha M, Berger B, Schuengel E, Korolov I, Derzsi A, Koepke M, Donko Z, Schulze J. A computationally assisted spectroscopic technique to measure secondary electron emission coefficients in radio frequency plasmas. Journal of Physics. D, Applied Physics, 2016, 49(23): 234001

63 Marcak A, Corbella C, de los Arcos T, von Keudell A. Note: Ion-induced secondary electron emission from oxidized metal surfaces measured in a particle beam reactor. Review of Scientific Instruments, 2015, 86(10): 106102

64 More W, Merino J, Monreal R, Pou P, Flores F. Role of energy-level shifts on auger neutralization processes: A calculation beyond the image potential. Physical Review. B, 1998, 58(11): 7385–7390

65 Newns D M, Makoshi K, Brako R, van Wunnik J N M. Charge transfer in inelastic ion and atom-surface collisions. Physica Scripta, 1983, T6: 5–14

66 Yoshimori A, Makoshi K. Time-dependent Newns-Anderson model. Progress in Surface Science, 1986, 21(3): 251–294

67 Los J, Geerlings J J C. Charge exchange in atom-surface collisions. Physics Reports, 1990, 190(3): 133–190

68 Pamperin M, Bronold F X, Fehske H. Ion-induced secondary electron emission from metal surfaces. Plasma Sources Science & Technology, 2018, 27(8): 084003

69 Wang N P, García E A, Monreal R, Flores F, Goldberg E C, Brongersma H H, Bauer P. Low-energy ion neutralization at surfaces: Resonant and auger processes. Physical Review A., 2001, 64(1): 012901

70 Valdés D, Goldberg E C, Blanco J M, Monreal R C. Linear combination of atomic orbitals calculation of the auger neutralization rate of He⁺ on Al(111), (100), and (110) surfaces. Physical Review. B, 2005, 71(24): 245417

71 Marbach J, Bronold F X, Fehske H. Resonant charge transfer at dielectric surfaces. European Physical Journal D, 2012, 66(4): 106

72 Marbach J, Bronold F X, Fehske H. Pseudoparticle approach for charge-transferring molecule-surface collisions. Physical Review. B, 2012, 86(11): 115417

73 Iglesias-García A, García E A, Goldberg E C. Role of He excited configurations in the neutralization of He⁺ ions colliding with a HOPG surface. Physical Review. B, 2013, 87(7): 075434

74 Iglesias-García A, García E A, Goldberg E C. Importance of considering helium excited states in He⁺ scattering by aluminum surfaces. Physical Review. B, 2014, 90(19): 195416

75 Pamperin M, Bronold F X, Fehske H. Many-body theory of the neutralization of strontium ions on gold surfaces. Physical Review. B, 2015, 91(3): 035440

76 Pamperin M, Bronold F X, Fehske H. Mixed-valence correlations in charge-transferring atom–surface collisions. Physica Scripta, 2015, T165: 014008

77 Gadzuk J W. Theory of atom-metal interactions I. Alkali atom adsorption. Surface Science, 1967, 6(2): 133–158

78 Gadzuk J W. Theory of atom-metal interactions II. One-electron transition matrix elements. Surface Science, 1967, 6(2): 159–170

79 Propst F M. Energy distribution of electrons ejected from tungsten by He⁺. Physical Review, 1963, 129(1): 7–11

80 Penn D R, Apell P. Theory of spin-polarized metastable-atomdeexcitation spectroscopy: Ni–He. Physical Review. B, 1990, 41(6): 3303–3315

81 Langreth D C, Nordlander P. Derivation of a master equation for charge-transfer processes in atom-surface collisions. Physical Review. B, 1991, 43(4): 2541–2557

82 Shao H, Langreth D C, Nordlander P. Many-body theory for charge transfer in atom-surface collisions. Physical Review. B, 1994, 49(19): 13929–13947

83 Shao H, Langreth D C, Nordlander P. Theoretical description of charge transfer in atom-surface collisions. In Rabalais J W, ed. Low Energy Ion-surface Interaction. New York: Wiley and Sons, 1994, 117

84 Marx D, Hutter J. Ab Initio Molecular Dynamics: Basic Theory and Advanced Methods. Cambridge: Cambridge University Press, 2009

85 Hafner J. Ab-initio simulations of materials using VASP: Density-functional theory and beyond. Journal of Computational Chemistry, 2008, 29(13): 2044–2078

86 Groß A. Ab initio molecular dynamics simulations of the O₂/Pt(111) interaction. Catalysis Today, 2016, 260: 60–65

87 Kühne T D. Second generation car–parrinello molecular dynamics. Wiley Interdisciplinary Reviews. Computational Molecular Science, 2014, 4(4): 391–406

88 Baer M. Beyond Born-Oppenheimer: Electronic Nonadiabatic Coupling Terms and Conical Intersections. New-York: Wiley-Interscience, 2006

89 Nosé S. A unified formulation of the constant temperature molecular dynamics methods. Journal of Chemical Physics, 1984, 81(1): 511–519

90 Henkelman G, Jónsson H. Improved tangent estimate in the nudged elastic band method for finding minimum energy paths and saddle points. Journal of Chemical Physics, 2000, 113(22): 9978–9985

91 Henkelman G, Uberuaga B P, Jónsson H. A climbing image nudged elastic band method for finding saddle points and minimum energy paths. Journal of Chemical Physics, 2000, 113(22): 9901–9904

92 Vineyard G H. Frequency factors and isotope effects in solid state rate processes. Journal of Physics and Chemistry of Solids, 1957, 3(1): 121–127

93 Laio A, Gervasio F L. Metadynamics: A method to simulate rare events and reconstruct the free energy in biophysics, chemistry and material science. Reports on Progress in Physics, 2008, 71(12): 126601

94 Martin R M. Electronic Structure: Basic Theory and Practical Methods. Cambridge: Cambridge University Press, 2004

95 Burke K. Perspective on density functional theory. Journal of Chemical Physics, 2012, 136(15): 150901

96 Becke A D. Perspective: Fifty years of density-functional theory in chemical physics. The Journal of Chemical Physics, 2014, 140(18): 18A301

97 Yu H S, Li S L, Truhlar D G. Perspective: Kohn-Sham density-functional theory descending a staircase. Journal of Chemical Physics, 2016, 145(13): 130901

98 Hohenberg P, Kohn W. Inhomogeneous electron gas. Physical Review, 1964, 136(3B): B864–B871

99 Kohn W, Sham L J. Self-consistent equations including exchange and correlation effects. Physical Review, 1965, 140(4A): A1133–A1138

100 Klimes J, Michaelides A. Perspective: Advances and challenges in treating van der Waals dispersion forces in density functional theory. Journal of Chemical Physics, 2012, 137(12): 120901

101 Perdew J P, Chevary J A, Vosko S H, Jackson K A, Pederson M R, Singh D J, Fiolhais C. Atoms, molecules, solids, and surfaces: Applications of the generalized gradient approximation for exchange and correlation. Physical Review. B, 1992, 46(11): 6671–6687

102 Perdew J P, Burke K, Ernzerhof M. Generalized gradient approximation made simple. Physical Review Letters, 1996, 77(18): 3865–3868

103 Tao J, Perdew J P, Staroverov V N, Scuseria G E. Climbing the density functional ladder: Nonempirical meta–generalized gradient approximation designed for molecules and solids. Physical Review Letters, 2003, 91(14): 146401

104 Kresse G, Furthmüller J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Computational Materials Science, 1996, 6(1): 15–50

105 Kresse G, Hafner J. Ab initio molecular dynamics for liquid metals. Physical Review. B, 1993, 47(1): 558–561

106 Kresse G, Hafner J. Ab initio molecular-dynamics simulation of the liquid-metal-amorphous-semiconductor transition in germanium. Physical Review. B, 1994, 49(20): 14251–14269

107 Kresse G, Furthmüller J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Physical Review. B, 1996, 54(16): 11169–11186

108 Giannozzi P, Baroni S, Bonini N, Calandra M, Car R, Cavazzoni C, Ceresoli D, Chiarotti G L, Cococcioni M, Dabo I, . Quantum espresso: A modular and open-source software project for quantum simulations of materials. Journal of Physics Condensed Matter, 2009, 21(39): 395502

109 Giannozzi P, Andreussi O, Brumme T, Bunau O, Nardelli M B, Calandra M, Car R, Cavazzoni C, Ceresoli D, Cococcioni M, . Advanced capabilities for materials modelling with quantum espresso. Journal of Physics Condensed Matter, 2017, 29(46): 465901

110 Mattsson A E, Schultz P A, Desjarlais M P, Mattsson T R, Leung K. Designing meaningful density functional theory calculations in material science—a primer. Modelling and Simulation in Materials Science and Engineering, 2005, 13(1): R1–R31

111 Hamann D R. Generalized norm-conserving pseudopotentials. Physical Review. B, 1989, 40(5): 2980–2987

112 Trouiller N, Martins J L. Efficient pseudopotentials for plane-wave calculations. Physical Review. B, 1991, 43(3): 1993–2006

113 Fuchs M, Scheffler M. Ab initio pseudopotentials for electronic structure calculations of poly-atomic systems using density-functional theory. Computer Physics Communications, 1999, 119(1): 67–98

114 Vanderbilt D. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism. Physical Review. B, 1990, 41(11): 7892–7895

115 Blöchl P E. Projector augmented-wave method. Physical Review. B, 1994, 50(24): 17953–17979

116 Kresse G, Joubert D. From ultrasoft pseudopotentials to the projector augmented-wave method. Physical Review. B, 1999, 59(3): 1758–1775

117 Modinos A. Resonance charge transfer in atom-surface scattering. Progress in Surface Science, 1987, 26(1): 19–46

118 Brako R, Newns D M. Theory of electronic processes in atom scattering from surfaces. Reports on Progress in Physics, 1989, 52(6): 655–697

119 Kimmel G A, Cooper B H. Dynamics of resonant charge transfer in low-energy alkali-metal-ion scattering. Physical Review. B, 1993, 48(16): 12164–12177

120 Winter H. Collisions of atoms and ions with surfaces under grazing incidence. Physics Reports, 2002, 367(5): 387–582

121 Race C P, Mason D R, Finnis M W, Foulkes W M C, Horsfield A P, Sutton A P. The treatment of electronic excitations in atomistic models of radiation damage in metals. Reports on Progress in Physics, 2010, 73(11): 116501

122 Wucher A, Duvenbeck A. Kinetic excitation of metallic solids: Progress towards a microscopic model. Nuclear Instruments & Methods in Physics Research. Section B, Beam Interactions with Materials and Atoms, 2011, 269(14): 1655–1660

123 Lindenblatt M, Pehlke E, Duvenbeck A, Rethfeld B, Wucher A. Kinetic excitation of solids: The concept of electronic friction. Nuclear Instruments & Methods in Physics Research. Section B, Beam Interactions with Materials and Atoms, 2006, 246(2): 333–339

124 Nienhaus H. Electronic excitations by chemical reactions on metal surfaces. Surface Science Reports, 2002, 45(1): 1–78

125 Diesing D, Hasselbrink E. Chemical energy dissipation at surfaces under uhv and high pressure conditions studied using metal-insulator-metal and similar devices. Chemical Society Reviews, 2016, 45(13): 3747–3755

126 Bünermann O, Jiang H, Dorenkamp Y, Kandratsenka A, Janke S M, Auerbach D J, Wodtke A M. Electron-hole pair excitation determines the mechanism of hydrogen atom adsorption. Science, 2015, 350(6266): 1346–1349

127 Wodtke A M. Electronically non-adiabatic influences in surface chemistry and dynamics. Chemical Society Reviews, 2016, 45(13): 3641–3657

128 Rittmeyer S P, Meyer J, Juaristi J I, Reuter K. Electronic friction-based vibrational lifetimes of molecular adsorbates: Beyond the independent-atom approximation. Physical Review Letters, 2015, 115(4): 046102

129 Rittmeyer S P, Bukas V J, Reuter K. Energy dissipation at metal surfaces. Advances in Physics: X, 2018, 3(1): 1381574

130 Alducin M, Muiño R D, Juaristi J I. Non-adiabatic effects in elementary reaction processes at metal surfaces. Progress in Surface Science, 2017, 92(4): 317–340

131 Janke S M, Auerbach D J, Wodtke A M, Kandratsenka A. An accurate full-dimensional potential energy surface for H-Au(111): Importance of nonadiabatic electronic excitation in energy transfer and adsorption. Journal of Chemical Physics, 2015, 143(12): 124708

132 Kroes G J, Pavanello M, Blanco-Rey M, Alducin M, Auerbach D J. Ab initio molecular dynamics calculations on scattering of hyperthermal H atoms from Cu(111) and Au(111). Journal of Chemical Physics, 2014, 141(5): 054705

133 Monturet S, Saalfrank P. Role of electronic friction during the scattering of vibrationally excited nitric oxide molecules from Au(111). Physical Review. B, 2010, 82(7): 075404

134 Mizielinski M S, Bird D M, Persson M, Holloway S. Electronic nonadiabatic effects in the adsorption of hydrogen atoms on metals. Journal of Chemical Physics, 2005, 122(8): 084710

135 Mizielinski M S, Bird D M, Persson M, Holloway S. Spectrum of electronic excitations due to the adsorption of atoms on metal surfaces. Journal of Chemical Physics, 2007, 126(3): 034705

136 Mizielinski M S, Bird D M, Persson M, Holloway S. Newnsanderson model of chemicurrents in H/Cu and H/Ag. Surface Science, 2008, 602(14): 2617–2622

137 Mizielinski M S, Bird D M. Accuracy of perturbation theory for nonadiabatic effects in adsorbate-surface dynamics. Journal of Chemical Physics, 2010, 132(18): 184704

138 Bird D M, Mizielinski M S, Lindenblatt M, Pehlke E. Electronic excitation in atomic adsorption on metals: A comparison of ab initio and model calculations. Surface Science, 2008, 602(6): 1212–1216

139 Lindenblatt M, van Heys J, Pehlke E. Molecular dynamics of nonadiabatic processes at surfaces: Chemisorption of H/Al(111). Surface Science, 2006, 600(18): 3624–3628

140 Lindenblatt M, Pehlke E. Time-dependent density-functional molecular-dynamics study of the isotope effect in chemicurrents. Surface Science, 2006, 600(23): 5068–5073

141 Lindenblatt M, Pehlke E. Ab initio simulation of the spin transition during chemisorption: H/Al(111). Physical Review Letters, 2006, 97(21): 216101

142 Grotemeyer M, Pehlke E. Electronic energy dissipation during scattering of vibrationally excited molecules at metal surfaces: Ab initio simulations for HCl/Al(111). Physical Review Letters, 2014, 112(4): 043201

143 Timmer M, Kratzer P. Electron-hole spectra created by adsorption on metals from density functional theory. Physical Review. B, 2009, 79(16): 165407

144 Zhao S, Kang W, Xue J, Zhang X, Zhang P H. + (D⁺,T⁺) beryllium collisions studied using time-dependent density functional theory. Physics Letters, 2015, 379(4): 319–326 (Part A)

145 Moss C L, Isborn C M, Li X. Ehrenfest dynamics with a time-dependent density-functional-theory calculation of lifetimes and resonant widths of charge-transfer states of Li⁺ near an aluminum cluster surface. Physical Review A., 2009, 80(2): 024503

146 Castro A, Isla M, Martínez J I, Alonso J A. Scattering of a proton with the Li4 cluster: Non-adiabatic molecular dynamics description based on time-dependent density-functional theory. Chemical Physics, 2012, 399: 130–134

147 Krasheninnikov A V, Miyamoto Y, Tománek D. Role of electronic excitation in ion collisions with carbon nanostructures. Physical Review Letters, 2007, 99(1): 016104

148 Bubin S, Wang B, Pantelides S, Varga K. Simulation of high-energy ion collisions with graphene fragments. Physical Review. B, 2012, 85(23): 235435

149 Ojanperä A, Krasheninnikov A V, Puska M. Electronic stopping power from first-principles calculations with account for core electron excitations and projectile ionization. Physical Review. B, 2014, 89(3): 035120

150 Wang Z, Li S S, Wang L W. Efficient real-time time-dependent density functional theory method and its application to collision of an ion with a 2D material. Physical Review Letters, 2015, 114(6): 063004

151 Yost D C, Yao Y, Kanai Y. Examining real-time time-dependent density functional theory nonequilibrium simulations for the calculation of electronic stopping power. Physical Review. B, 2017, 96(11): 115134

152 Schleife A, Kanai Y, Correa A A. Accurate atomistic first-principles calculations of electronic stopping. Physical Review. B, 2015, 91(1): 014306

153 Correa A A, Kohanoff J, Artacho E, Sánchez-Portal D, Caro A. Nonadiabatic forces in ion-solid interactions: The initial stages of radiation damage. Physical Review Letters, 2012, 108(21): 213201

154 Zeb M A, Kohanoff J, Sánchez-Portal D, Arnau A, Juaristi J I, Artacho E. Electronic stopping power in gold: The role of d electrons and the H/He anomaly. Physical Review Letters, 2012, 108(22): 225504

155 Ullah R, Corsetti F, Sánchez-Portal D, Artacho E. Electronic stopping power in narrow band gap semiconductor from first principles. Physical Review. B, 2015, 91(12): 125203

156 Time-dependent Density Functional Theory. M arques M A L, Ullrich C A, Nogueira F, Rubio A, Burke K, Gross E K U, eds. Berlin: Springer, 2006

157 Fundamentals of Time-Dependent Density Functional Theory. Marques M A L, Maitra N T, Nogueira F M S, Gross E K U, Rubio A, eds. Berlin: Springer, 2012

158 Ullrich C A. Time-Dependent Density-Functional Theory. Oxford: Oxford University Press, 2012

159 Ullrich C A, Yang Z H. A brief compendium of time-dependent density functional theory. Brazilian Journal of Physics, 2014, 44(1): 154–158

160 Maitra N T. Perspective: Fundamental aspects of time-dependent density functional theory. Journal of Chemical Physics, 2016, 144(22): 220901

161 Runge E, Gross E K U. Density-functional theory for time-dependent systems. Physical Review Letters, 1984, 52(12): 997–1000

162 Gross E K U, Kohn W. Local density-functional theory of frequency-dependent linear response. Physical Review Letters, 1985, 55(26): 2850–2852

163 Provorse M R, Isborn C M. Electron dynamics with real-time time-dependent density functional theory. International Journal of Quantum Chemistry, 2016, 116(10): 739–749

164 Nagano R, Yabana K, Tazawa T, Abe Y. Time-dependent mean-field description for multiple charge transfer processes in Ar⁸⁺–Ar collisions. Physical Review A., 2000, 62(6): 062721

165 Nazarov V U, Pitarke J M, Takada Y, Vignale G, Chang Y C. Including nonlocality in the exchange-correlation kernel from time-dependent current density functional theory: Application to the stopping power of electron liquids. Physical Review. B, 2007, 76(20): 205103

166 Tully J C. Molecular dynamics with electronic transitions. Journal of Chemical Physics, 1990, 93(2): 1061–1071

167 Shenvi N, Roy S, Tully J C. Nonadiabatic dynamics at metal surfaces: Independent-electron surface hopping. Journal of Chemical Physics, 2009, 130(17): 174107

168 Marques M A L, Castro A, Bertsch G F, Rubio A. Octopus: A first-principles tool for excited electron-ion dynamics. Computer Physics Communications, 2003, 151(1): 60–78

169 Foglia N O, Morzan U N, Estrin D A, Scherlies D A, Lebrero M C G. Role of core electrons in quantum dynamics using TDDFT. Journal of Chemical Theory and Computation, 2017, 13(1): 77–85

170 Avendaño Franco G. Charge transfer processes in atomic collisions from first principles. Dissertation for the Doctoral Degree. Louvain-la-Neuve: Université Catholique de Louvain, 2013

171 German K A H, Weare C B, Yarmoff J A. Inner-shell promotions in low-energy Li⁺–Al collisions at clean and alkali-covered Al(100) surfaces. Physical Review. B, 1994, 50(19): 14452–14466

172 Castro A, Appel H, Oliveira M, Rozzi C A, Andrade X, Lorenzen F, Marques M A L, Gross E K U, Rubio A. Octopus: A tool for the application of time-dependent density functional theory. Physica Status Solidi. B, Basic Research, 2006, 243(11): 2465–2488

173 Andrade X, Strubbe D, De Giovannini U, Larsen A H, Oliveira M J T, Alberdi-Rodriguez J, Varas A, Theophilou I, Helbig N, Verstraete M J, . Real-space grids and the octopus code as tools for the development of new simulation approaches for electronic systems. Physical Chemistry Chemical Physics, 2015, 17(47): 31371–31396

174 Shukri A A, Bruneval F, Reining L. Ab initio electronic stopping power of protons in bulk materials. Physical Review. B, 2016, 93(3): 035128

175 Markin S N, Primetzhofer D, Spitz M, Bauer P. Electronic stopping of low-energy H and He in Cu and Au investigated by timeof-flight low-energy ion scattering. Physical Review. B, 2009, 80(20): 205105

176 Mason D R, le Page J, Race C P, Foulkes W M C, Finnis M W, Sutton A P. Electronic damping of atomic dynamics in irradiation damage of metals. Journal of Physics Condensed Matter, 2007, 19(43): 436209

177 Grotemeyer M K. Ab initio Berechnungen zur Anregung von Elektronen-Loch-Paaren durch Molekülschwingungen am Beispiel von HCl auf Al(111). Dissertation for the Doctoral Degree. Kiel: Christian-Albrechts-Universität zu Kiel, 2016 (in German)

178 D’Agosta R, Di Ventra M. Foundations of stochastic time-dependent current-density functional theory for open quantum systems: Potential pitfalls and rigorous results. Physical Review. B, 2013, 87(15): 155129

179 Ullrich C A. Time-dependent density-functional theory beyond the adiabatic approximation: Insights from a two-electron model system. Journal of Chemical Physics, 2006, 125(23): 234108

180 Kapoor V. Autoionization in time-dependent density-functional theory. Physical Review. A, 2016, 93(6): 063408

181 Lorente N, Monreal R, Alducin M. Local theory of auger neutralization for slow and compact ions interacting with metal surfaces. Physical Review A., 1994, 49(6): 4716–4725

182 Monreal R C. Auger neutralization and ionization processes for charge exchange between slow noble gas atoms and solid surfaces. Progress in Surface Science, 2014, 89(1): 80–125

183 Balzer K, Rasmussen M, Schlünzen N, Joost J P, Bonitz M. Doublon formation by ions impacting a strongly correlated finite lattice system. Physical Review Letters, 2018, 121(26): 267602

184 Keldysh L. Diagram technique for nonequilibrium processes. . Soviet Physics, JETP, 1965, 20(4): 1018–1026

185 Kadanoff L, Baym G. Quantum Statistical Mechanics. New York: Benjamin, 1962

186 Bonitz M, Kremp D. Kinetic energy relaxation and correlation time of nonequilibrium many-particle systems. Physics Letters, 1996, 212(1-2): 83–90 (Part A)

187 Bonitz M, Kremp D, Scott D C, Binder R, Kraeft W D, Köhler H S. Numerical analysis of non-Markovian effects in charge-carrier scattering: One-time versus two-time kinetic equations. Journal of Physics Condensed Matter, 1996, 8(33): 6057–6071

188 Bonitz M. Correlation time approximation in non-markovian kinetics. Physics Letters, 1996, 221(1-2): 85–93 (Part A)

189 Kremp D, Bonitz M, Kraeft W, Schlanges M. Non-Markovian Boltzmann equation. Annals of Physics, 1997, 258(2): 320–359

190 Danielewicz P. Quantum theory of nonequilibrium processes ii. Application to nuclear collisions. Annals of Physics, 1984, 152(2): 305–326

191 Köhler H S. Memory and correlation effects in nuclear collisions. Physical Review. C, 1995, 51(6): 3232–3239

192 Bányai L, Thoai D B T, Reitsamer E, Haug H, Steinbach D, Wehner M U, Wegener M, Marschner T, Stolz W. Exciton–lophonon quantum kinetics: Evidence of memory effects in bulk gaas. Physical Review Letters, 1995, 75(11): 2188–2191

193 Kwong N, Bonitz M, Binder R, Köhler H. Semiconductor Kadanoff-Baym equations results for optically excited electron-hole plasmas semiconductor quantum wells. Physica Status Solidi. B, Basic Research, 1998, 206: 197

194 Binder R, Köhler H S, Bonitz M, Kwong N. Green’s function description of momentum-orientation relaxation of photoexcited electron plasmas in semiconductors. Physical Review. B, 1997, 55(8): 5110–5116

195 Bonitz M, Balzer K, van Leeuwen R. Invariance of the Kohn center-of-mass mode in a conserving theory. Physical Review. B, 2007, 76(4): 045341

196 Balzer K, Bonitz M, van Leeuwen R, Stan A, Dahlen N E. Nonequilibrium Green’s function approach to strongly correlated few-electron quantum dots. Physical Review. B, 2009, 79(24): 245306

197 Kremp D, Bornath T, Bonitz M, Schlanges M. Quantum kinetic theory of plasmas in strong laser fields. Physical Review. E, 1999, 60(4): 4725–4732

198 Bonitz M, Bornath T, Kremp D, Schlanges M, Kraeft W D. Quantum kinetic theory for laser plasmas. Dynamical screening in strong fields. Contributions to Plasma Physics, 1999, 39(4): 329–347

199 Stefanucci G, van Leeuwen R. Nonequilibrium Many-body Theory of Quantum Systems. Cambridge: Cambridge University Press, 2013

200 Balzer K, Bauch S, Bonitz M. Efficient grid-based method in nonequilibrium Green’s function calculations: Application to model atoms and molecules. Physical Review A., 2010, 81(2): 022510 doi:10.1103/PhysRevA.81.022510

201 Balzer K, Bauch S, Bonitz M. Time-dependent second-order Born calculations for model atoms and molecules in strong laser fields. Physical Review A., 2010, 82(3): 033427

202 Verdozzi C, Wacker A, Almbladh C O, Bonitz M. Progress in nonequilibrium Green’s functions (PNGF VI). Journal of Physics: Conference Series, 2016, 696(1): 011001

203 Schlünzen N, Hermanns S, Bonitz M, Verdozzi C. Dynamics of strongly correlated fermions: Ab initio results for two and three dimensions. Physical Review. B, 2016, 93(3): 035107

204 Bonitz M, Scharnke M, Schlünzen N. Time-reversal invariance of quantum kinetic equations II: Density operator formalism. Contributions to Plasma Physics, 2018, 58(10): 58

205 TRIM and SRIM code packages. Available at the website of srim.org (accessed March 11, 2019)

206 Heese S. Dielectric function of graphene with yambo. Dissertation for the Bachelor Degree. Kiel: Christian-Albrechts-Universität zu Kiel, 2017

207 Bonitz M, Balzer K, Schlünzen N, Rodriguez Rasmussen M, Joost J P. Ion Impact Induced Ultrafast Electron Dynamics in Correlated Materials and Finite Graphene Clusters. Physica Status Solidi, 2019, 1800490: (b)

208 Pamperin M, Bronold F X, Fehske H. Many-body theory of the neutralization of strontium ions on gold surfaces. Physical Review. B, 2015, 91(3): 035440

209 Brenig W. Theory of inelastic atom-surface scattering: Average energy loss and energy distribution. Zeitschrift für Physik B, Condensed Matter, 1979, 36(1): 81–87

210 Bonitz M, Rosenthal L, Fujioka K, Zaporojtchenko V, Faupel F, Kersten H. Towards a particle based simulation of complex plasma driven nanocomposite formation. Contributions to Plasma Physics, 2012, 52(10): 890–898

211 Brenig W, Pehlke E. Reaction dynamics of H₂ on Si. Ab initio supported model calculations. Progress in Surface Science, 2008, 83(5): 263–336

212 Bronold F X, Fehske H. Kinetic modeling of the electronic response of a plasma-facing solid. Journal of Physics. D, Applied Physics, 2017, 50(29): 294003

213 Langmuir I, Mott-Smith H. Studies of electric discharges in gases at low pressure. General Electric Review, 1924, 27: 449

214 Robertson S. Sheaths in laboratory and space plasmas. Plasma Physics and Controlled Fusion, 2013, 55(9): 093001

215 Brinkmann R P. From electron depletion to quasi-neutrality: The sheath-bulk transition in rf modulated discharges. Journal of Physics. D, Applied Physics, 2009, 42(19): 194009

216 Franklin R N. The plasma-sheath boundary region. Journal of Physics. D, Applied Physics, 2003, 36(22): R309–R320

217 Riemann K U. The Bohm criterion and sheath formation. Journal of Physics. D, Applied Physics, 1991, 24(4): 493–518

218 Schwager L A, Birdsall C K. Collector and source sheaths of a finite ion temperature plasma. Physics of Fluids. B, Plasma Physics, 1990, 2(5): 1057–1068

219 Campanell M D, Umansky M V. Strongly emitting surfaces unable to float below plasma potential. Physical Review Letters, 2016, 116(8): 085003

220 Langendorf S, Walker M. Effect of secondary electron emission on the plasma sheath. Physics of Plasmas, 2015, 22(3): 033515

221 Sheehan J P, Hershkowitz N, Kaganovich I D, Wang H, Raitses Y, Barnat E V, Weatherford B R, Sydorenko D. Kinetic theory of plasma sheaths surrounding electron-emitting surfaces. Physical Review Letters, 2013, 111(7): 075002

222 Sydorenko D, Kaganovich I D, Raitses Y, Smolyakov A. Breakdown of a space charge limited regime of a sheath in a weakly collisional plasma bounded by walls with secondary electron emission. Physical Review Letters, 2009, 103(14): 145004

223 Taccogna F, Longo S, Capitelli M. Plasma-surface interaction model with secondary electron emission effects. Physics of Plasmas, 2004, 11(3): 1220–1228

224 Hu P N, Ziering S. Collisionless theory of a plasma sheath near an electrode. Physics of Fluids, 1966, 9(11): 2168–2179

225 Franklin R N. Plasma Phenomena in Gas Discharges. Oxford: Clarendon Press, 1976

226 Becker M M, Grubert G K, Loffhagen D. Boundary conditions for the electron kinetic equation using expansion techniques. European Physical Journal Applied Physics, 2010, 51(1): 11001

227 Kushner M J. Modeling of microdischarge devices: Pyramidal structures. Journal of Applied Physics, 2004, 95(3): 846–859

228 Golubovskii Y B, Maiorov V A, Behnke J, Behnke J F. Influence of interaction between charged particles and dielectric surface over a homogeneous barrier discharge in nitrogen. Journal of Physics. D, Applied Physics, 2002, 35(8): 751–761

229 Dussart R, Overzet L J, Lefaucheux P, Dufour T, Kulsreshath M, Mandra M A, Tillocher T, Aubry O, Dozias S, Ranson P, . Integrated micro-plasmas in silicon operating in helium. European Physical Journal D, 2010, 60(3): 601–608

230 Kulsreshath M K, Schwaederle L, Overzet L J, Lefaucheux P, Ladroue J, Tillocher T, Aubry O, Woytasik M, Schelcher G, Dussart R. Study of dc micro-discharge arrays made in silicon using cmos compatible technology. Journal of Physics. D, Applied Physics, 2012, 45(28): 285202

231 Eden J G, Park S J, Cho J H, Kim M H, Houlahan T J, Li B, Kim E S, Kim T L, Lee S K, Kim K S, . Plasma science and technology in the limit of the small: Microcavity plasmas and emerging applications. IEEE Transactions on Plasma Science, 2013, 41(4): 661–675

232 Tchertchian P A, Wagner C J, Houlahan T J Jr, Li B, Sievers D J, Eden J G. Control of the interface between electron-hole and electron-ion plasmas: Hybrid semiconductor-gas phase devices as a gateway for plasma science. Contributions to Plasma Physics, 2011, 51(10): 889–905

233 Ostrom N P, Eden J G. Microcavity plasma photodetectors: Photosensitivity, dynamic range, and the plasma-semiconductor interface. Applied Physics Letters, 2005, 87(14): 141101

234 Sternovsky Z. The effect of ion-neutral collisions on the weakly collisional plasma-sheath and the reduction of the ion flux to the wall. Plasma Sources Science & Technology, 2005, 14(1): 32–35

235 Riemann K U. Kinetic analysis of the collisional plasma-sheath transition. Journal of Physics. D, Applied Physics, 2003, 36(22): 2811–2820

236 Sheridan T E, Goree J. Collisional plasma sheath model. Physics of Fluids. B, Plasma Physics, 1991, 3(10): 2796–2804

237 Tsankov T V, Czarnetzki U. Information hidden in the velocity distribution of ions and the exact kinetic bohm criterion. Plasma Sources Science & Technology, 2017, 26(5): 055003

238 Lacroix D, Hermanns S, Hinz C M, Bonitz M. Ultrafast dynamics of finite Hubbard clusters: A stochastic mean-field approach. Physical Review. B, 2014, 90(12): 125112

239 Hopjan M, Karlsson D, Ydman S, Verdozzi C, Almbladh C O. Merging features from green’s functions and time dependent density functional theory: A route to the description of correlated materials out of equilibrium? Physical Review Letters, 2016, 116(23): 236402