

Frontiers of Chemical Science and Engineering >
Recent progress in hydrodynamic characteristics research and application of annular centrifugal extractors
Received date: 04 Nov 2021
Accepted date: 31 Dec 2021
Published date: 15 Jun 2022
Copyright
The annular centrifugal extractor (ACE) integrates mixing and separation. It has been widely used in many industrial fields because of its low residence time, compact structure, and high mass transfer efficiency. Most of the literature has focused on flow instabilities, flow visualization, and computational fluid dynamics simulations. More recently, research on hydrodynamic behavior and structural optimization has received widespread attention. With the development of ACE technology, applications have been broadened into several new areas. Hence, this paper reviews research progress regarding ACE in terms of hydrodynamic characteristics and the structural improvements. The latest applications covering hydrometallurgy, nuclear fuel reprocessing, bio-extraction, catalytic reaction, and wastewater treatment are presented. We also evaluate future work in droplet breakup and coalescence mechanisms, structural improvements specific to different process requirements, scaling-up methods, and stability and reliability after scaling-up.
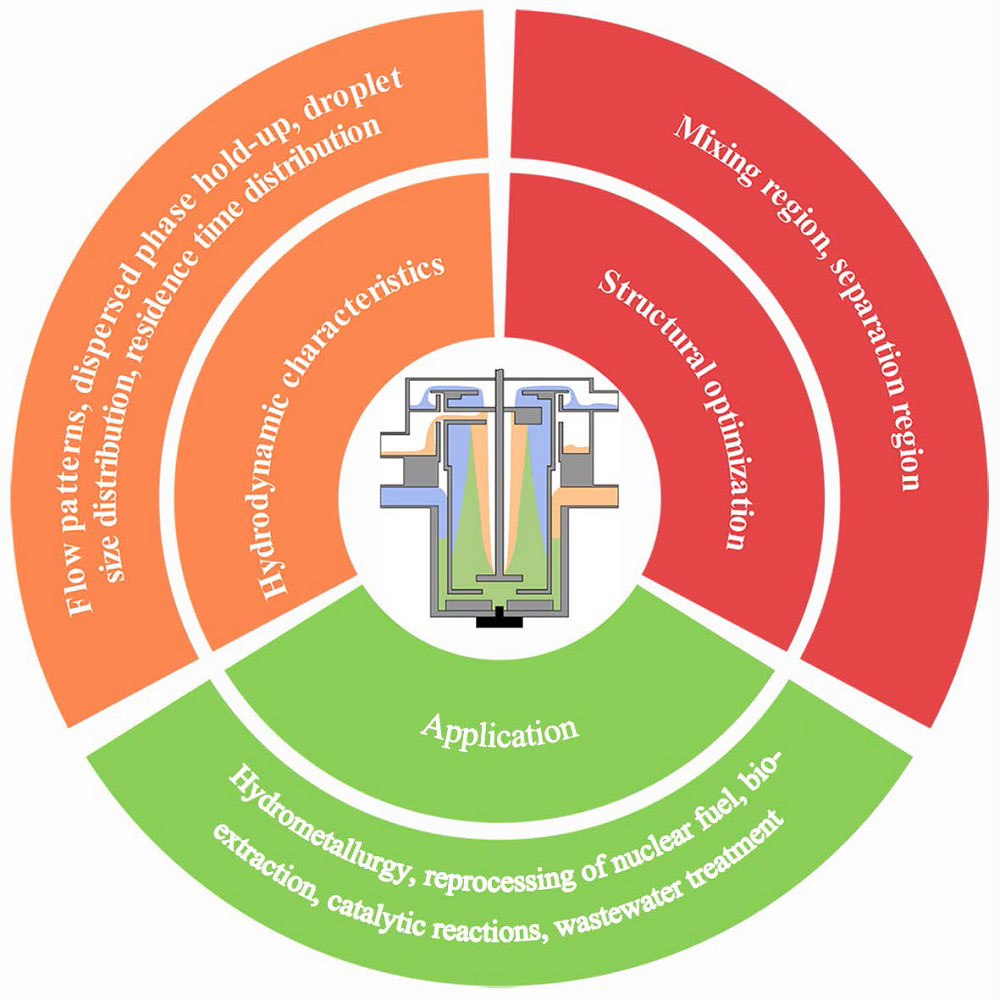
Hang Yang, Xiaoyong Yang, Xiao Dong, Zhaojin Lu, Zhishan Bai, Yinglei Wang, Fulei Gao. Recent progress in hydrodynamic characteristics research and application of annular centrifugal extractors[J]. Frontiers of Chemical Science and Engineering, 2022, 16(6): 854-873. DOI: 10.1007/s11705-022-2156-0
| 1 |
HerediaV, PruvostJ, GonçalvesO, DrouinD, MarchalL. Lipid recovery from nannochloropsis gaditana using the wet pathway: investigation of the operating parameters of bead milling and centrifugal extraction. Algal Research, 2021, 56 : 102318
|
| 2 |
DeshpandeJ B, NavaleG R, DharneM S, KulkarniA A. Continuous interfacial centrifugal separation and recovery of silver nanoparticles. Chemical Engineering & Technology, 2020, 43( 3): 582– 592
|
| 3 |
ZhangP L, WangS C, TangK W, XuW F, QiuY R, XiongB Q, LiuY. Multistage enantioselective reactive extraction of terbutaline enantiomers by hydrophobic phase transfer: experiment and modeling. Separation and Purification Technology, 2018, 191 : 208– 215
|
| 4 |
ZhangP L, WangS C, TangK W, XuW F, HeF, QiuY R. Modeling multiple chemical equilibrium in chiral extraction of metoprolol enantiomers from single-stage extraction to fractional extraction. Chemical Engineering Science, 2018, 177 : 74– 88
|
| 5 |
ZhangP L, FengX F, TangK W, XuW F. Study on enantioseparation of α-cyclopentyl-mandelic acid enantiomers using continuous liquid–liquid extraction in centrifugal contactor separators: experiments and modeling. Chemical Engineering and Processing, 2016, 107 : 168– 176
|
| 6 |
TangK W, WangY Q, ZhangP L, HuangY, HuaJ. Optimization study on continuous separation of equol enantiomers using enantioselective liquid–liquid extraction in centrifugal contactor separators. Process Biochemistry, 2016, 51( 1): 113– 123
|
| 7 |
JingX H, NingP G, CaoH B, SunZ, WangJ Y. Separation of V(V) and Cr(VI) in leaching solution using annular centrifugal contactors. Chemical Engineering Journal, 2017, 315 : 373– 381
|
| 8 |
JingX H, WangJ Y, CaoH B, NingP G, SunZ. Rapid selective extraction of V(V) from leaching solution using annular centrifugal contactors and stripping for NH4VO3. Separation and Purification Technology, 2017, 187 : 407– 414
|
| 9 |
MiaoQ D, SunT X, ChenH L, ZhengQ, DuanW H. Comparison of the hydraulic characteristics of the 30% TRPO/kerosene-HNO3 and iPr-C[4]C-6/n-octanol-HNO3 systems in an annular centrifugal extractor. Progress in Nuclear Energy, 2021, 136 : 103734
|
| 10 |
MohapatraP K, VermaP K, PrabhuD R, RautD R. Extraction of 137Cs from acidic feed by centrifugal contactors using a solution of calix[4]arene-bis-1,2-benzo-crown-6 in phenyltrifluoromethyl sulphone. Nuclear Technology, 2019, 205( 8): 1119– 1125
|
| 11 |
SchuurB, WinkelmanJ G M, de VriesJ G, HeeresH J. Experimental and modeling studies on the enantio-separation of 3,5-dinitrobenzoyl-(R),(S)-leucine by continuous liquid–liquid extraction in a cascade of centrifugal contactor separators. Chemical Engineering Science, 2010, 65( 16): 4682– 4690
|
| 12 |
BernsteinG, GrosvenorD, LencJ, LevitzN. A high-capacity annular centrifugal contactor. Nuclear Technology, 1973, 20( 3): 200– 202
|
| 13 |
VedantamS, JoshiJ B. Annular centrifugal contactors—a review. Chemical Engineering Research & Design, 2006, 84( 7): 522– 542
|
| 14 |
VedantamS, WardleK E, TamhaneT V, RanadeV V, JoshiJ B. CFD simulation of annular centrifugal extractors. International Journal of Chemical Engineering, 2012, 2012 : 759397
|
| 15 |
DuanW H, ZhaoM M, WangC Q, CaoS. Recent advances in the development and application of annular centrifugal contactors in the nuclear industry. Solvent Extraction and Ion Exchange, 2014, 32( 1): 1– 26
|
| 16 |
JingX H, NingP G, CaoH B, WangJ Y, SunZ. A review of application of annular centrifugal contactors in aspects of mass transfer and operational security. Hydrometallurgy, 2018, 177 : 41– 48
|
| 17 |
JosephD D, NguyenK, BeaversG S. Non-uniqueness and stability of the configuration of flow of immiscible fluids with different viscosities. Journal of Fluid Mechanics, 1984, 141 : 319– 345
|
| 18 |
CamperoR J, VigilR D. Spatiotemporal patterns in liquid–liquid Taylor–Couette–Poiseuille flow. Physical Review Letters, 1997, 79( 20): 3897– 3900
|
| 19 |
ZhuX, VigilR D. Banded liquid–liquid Taylor–Couette–Poiseuille flow. AIChE Journal. American Institute of Chemical Engineers, 2001, 47( 9): 1932– 1940
|
| 20 |
SatheM J, DeshmukhS S, JoshiJ B, KogantiS B. Computational fluid dynamics simulation and experimental investigation: study of two-phase liquid–liquid flow in a vertical Taylor–Couette contactor. Industrial & Engineering Chemistry Research, 2010, 49( 1): 14– 28
|
| 21 |
CampbellC, OlsenM G, Dennis VigilR. Flow regimes in two-phase hexane/water semibatch vertical Taylor vortex flow. Journal of Fluids Engineering, 2019, 141( 11): 111203
|
| 22 |
CampbellC, OlsenM G, VigilR D. Jet breakup regimes in liquid–liquid Taylor vortex flow. International Journal of Multiphase Flow, 2020, 131 : 103401
|
| 23 |
BaierG, GrahamM D. Two-fluid Taylor–Couette flow: experiments and linear theory for immiscible liquids between corotating cylinders. Physics of Fluids, 1998, 10( 12): 294– 303
|
| 24 |
NakaseM, MakabeR, TakeshitaK. Relation between oil–water flow and extraction performance in liquid–liquid countercurrent centrifugal extractors with Taylor vortices. Journal of Nuclear Science and Technology, 2013, 50( 3): 287– 295
|
| 25 |
ChenM X, XieT L, XuC. Continuous counter-current centrifugal extraction column with high throughput using a spiral inner cylinder. Chemical Engineering and Processing, 2018, 125 : 1– 7
|
| 26 |
XuY, WangJ G, ZhaoS L, BaiZ S. PIV experimental study on the flow field in the rotor zone of an annular centrifugal contactor. Chemical Engineering Research & Design, 2015, 94 : 691– 701
|
| 27 |
PatraJ, PandeyN K, MuduliU K, NatarajanR, JoshiJ B. Hydrodynamic study of flow in the rotor region of annular centrifugal contactors using CFD simulation. Chemical Engineering Communications, 2013, 200( 4): 471– 493
|
| 28 |
WardleK E, AllenT R, SwaneyR. CFD simulation of the separation zone of an annular centrifugal contactor. Separation Science and Technology, 2009, 44( 3): 517– 542
|
| 29 |
YangX Y. Study on synergetic treatment of sodium sulfate wastewater from coal tar processing by ternary solvent and centrifugal extraction. Dissertation for the Doctoral Degree. Shanghai: East China University of Science and Technology, 2019, 97– 106 (in Chinese)
|
| 30 |
LiS W, DuanW H, ChenJ, WangJ C. CFD simulation of gas-liquid–liquid three-phase flow in an annular centrifugal contactor. Industrial & Engineering Chemistry Research, 2012, 51( 34): 11245– 11253
|
| 31 |
GandhirA, WardleK E. CFD analysis of fluid flow above the upper weir of an annular centrifugal contactor. Separation Science and Technology, 2012, 47( 1): 1– 10
|
| 32 |
AyyappaS V N, BalamuruganM, KumarS, Kamachi MudaliU. Mass transfer and hydrodynamic studies in a 50 mm diameter centrifugal extractor. Chemical Engineering and Processing, 2016, 105 : 30– 37
|
| 33 |
KadamB D, JoshiJ B, KogantiS B, PatilR N. Dispersed phase hold-up, effective interfacial area and Sauter mean drop diameter in annular centrifugal extractors. Chemical Engineering Research & Design, 2009, 87( 10): 1379– 1389
|
| 34 |
DavisM W, WeberE J. Liquid–liquid extraction between rotating concentric cylinders. Industrial & Engineering Chemistry, 1960, 52( 11): 929– 934
|
| 35 |
GrafschafterA, SiebenhoferM. Design rules for the Taylor–Couette disc contactor. Chemie Ingenieur Technik (Weinheim), 2017, 89( 4): 409– 415
|
| 36 |
De SantisA, HansonB C, FairweatherM. Hydrodynamics of annular centrifugal contactors: a CFD analysis using a novel multiphase flow modelling approach. Chemical Engineering Science, 2021, 242 : 116729
|
| 37 |
WyattN B, O’HernT J, SheldenB. Drop-size distributions and spatial distributions in an annular centrifugal contactor. AIChE Journal. American Institute of Chemical Engineers, 2013, 59( 6): 2219– 2226
|
| 38 |
CampbellC, OlsenM, VigilR. Droplet size distributions in liquid–liquid semi-batch Taylor vortex flow. AIP Advances, 2020, 10( 8): 085316
|
| 39 |
WardleK E. Liquid–liquid mixing studies in annular centrifugal contactors comparing stationary mixing vane options. Solvent Extraction and Ion Exchange, 2015, 33( 7): 671– 690
|
| 40 |
TamhaneT V, JoshiJ B, MudaliK, NatarajanR, PatilR N. Measurement of drop size characteristics in annular centrifugal extractors using phase doppler particle analyzer (PDPA). Chemical Engineering Research & Design, 2012, 90( 8): 985– 597
|
| 41 |
SchuurB, KraaiG N, WinkelmanJ G M, HeeresH J. Hydrodynamic features of centrifugal contactor separators: experimental studies on liquid hold-up, residence time distribution, phase behavior and drop size distributions. Chemical Engineering and Processing, 2012, 55 : 8– 19
|
| 42 |
KumarS, MudaliK. Experimental measurements of drop size distributions in 30 mm diameter annular centrifugal contactor with 30% TBP-nitric acid biphasic system. International Journal of Nuclear Energy, 2013, 2013 : 402505
|
| 43 |
DreweryS, HeeresE, PercivalS, RhodesC, GeorgeM S, WilbersE, WoodheadD A. Experimental and modelling studies of mass transfer in centrifugal contactors. Procedia Chemistry, 2012, 7 : 341– 348
|
| 44 |
NakaseM, KinuhataH, TakeshitaK. Multi-staging for extraction of cesium from nitric acid by a single liquid–liquid countercurrent centrifugal extractor with Taylor vortices. Journal of Nuclear Science and Technology, 2013, 50( 11): 1089– 1098
|
| 45 |
NakaseM, TakeshitaK. Numerical and experimental study on oil-water dispersion in new countercurrent centrifugal extractor. Procedia Chemistry, 2012, 7 : 288– 294
|
| 46 |
HaasP A. Turbulent dispersion of aqueous drops in organic liquids. AIChE Journal. American Institute of Chemical Engineers, 1987, 33( 6): 987– 995
|
| 47 |
FarzadR, PuttingerS, PirkerS, SchneiderbauerS. Investigation of droplet size distribution for liquid–liquid emulsions in Taylor−Couette flows. Journal of Dispersion Science and Technology, 2017, 39( 2): 250– 258
|
| 48 |
CoulaloglouC A, TavlaridesL L. Description of interaction processes in agitated liquid–liquid dispersions. Chemical Engineering Science, 1977, 32( 11): 1289– 1297
|
| 49 |
EskinD, TaylorS D, YangD. Modeling of droplet dispersion in a turbulent Taylor–Couette flow. Chemical Engineering Science, 2017, 161 : 36– 47
|
| 50 |
EggertA, SibirtsevS, MenneD, JupkeA. Liquid–liquid centrifugal separation—new equipment for optical (photographic) evaluation at laboratory scale. Chemical Engineering Research & Design, 2017, 127 : 170– 179
|
| 51 |
FridjonssonE, ChandrasekeraT, SedermanA J, JohnsM, ZhaoC, MiddelbergA. Imaging the effects of peptide bio-surfactants on droplet deformation in a Taylor–Couette shear cell. Soft Matter, 2011, 7( 6): 2961– 2967
|
| 52 |
QiaoJ, DengR S, WangC H. Droplet behavior in a Taylor vortex. International Journal of Multiphase Flow, 2014, 67 : 132– 139
|
| 53 |
WardleK. Hybrid multiphase CFD simulation for liquid–liquid interfacial area prediction in annular centrifugal contactors. In: Proceedings of International Nuclear Fuel Cycle Conference, GLOBAL 2013: Nuclear Energy at a Crossroads. La Grange Pork, IL: Americen Nuclear Society, 2013
|
| 54 |
MartinA D. Interpretation of residence time distribution data. Chemical Engineering Science, 2000, 55( 23): 5907– 5917
|
| 55 |
TamhaneT V, JoshiJ B, Kamachi MudaliU, NatarajanR, PatilR N. Axial mixing in annular centrifugal extractors. Chemical Engineering Journal, 2012, 207-208 : 462– 472
|
| 56 |
LevenspielO. Chemical Reaction Engineering. New York: John Wiley & Sons, 1999: 321– 324
|
| 57 |
DeshmukhS S, SatheM J, JoshiJ B, KogantiS B. Residence time distribution and flow patterns in the single-phase annular region of annular centrifugal extractor. Industrial & Engineering Chemistry Research, 2009, 48( 1): 37– 46
|
| 58 |
VedantamS, JoshiJ B, KogantiS B. CFD Simulation of RTD and mixing in the annular region of a Taylor–Couette contactor. Industrial & Engineering Chemistry Research, 2006, 45( 18): 6360– 6367
|
| 59 |
DuanW H, SunT X, WangJ H. Separation of Nd3+ and Fe3+ by non-equilibrium solvent extraction using an annular centrifugal contactor. Separation and Purification Technology, 2015, 146 : 108– 113
|
| 60 |
ZhaoM M, CaoS, DuanW H. Effects of some parameters on mass-transfer efficiency of a ϕ20 mm annular centrifugal contactor for nuclear solvent extraction processes. Progress in Nuclear Energy, 2014, 74 : 154– 159
|
| 61 |
BaiZ S, ZhangJ, GanR W, XuY, LiuW J. Effect of cylinder rotation on flow characteristics of the annular region in annular centrifugal extractor. Separation Science and Technology, 2015, 50( 6): 813– 819
|
| 62 |
BrownM A, WardleK E, LumettaG, GelisA V. Accomplishing equilibrium in ALSEP: demonstrations of modified process chemistry on 3D printed enhanced annular centrifugal contactors. Procedia Chemistry, 2016, 21 : 167– 173
|
| 63 |
ManavalanB, JoshiJ B, PandeyN K. Design modification in the stationary bowl of annular centrifugal extractors to handle adverse conditions. Industrial & Engineering Chemistry Research, 2020, 59( 25): 11757– 11766
|
| 64 |
WardleK E, AllenT R, AndersonM H, SwaneyR E. Analysis of the effect of mixing vane geometry on the flow in an annular centrifugal contactor. AIChE Journal. American Institute of Chemical Engineers, 2009, 55( 9): 2244– 2259
|
| 65 |
DengR, Yohanes ArifinD, Ye ChynM, WangC H. Taylor vortex flow in presence of internal baffles. Chemical Engineering Science, 2010, 65( 16): 4598– 4605
|
| 66 |
DeshmukhS S, JoshiJ B, KogantiS B. Flow visualization and three-dimensional CFD simulation of the annular region of an annular centrifugal extractor. Industrial & Engineering Chemistry Research, 2008, 47( 10): 3677– 3686
|
| 67 |
SuY G, TangJ X, YangX X, WangR J. Effect of geometrical parameters on extraction efficiency of the annular centrifugal contactor. Separations, 2021, 8( 102): 1– 11
|
| 68 |
FanZ Bai Z S XuY YangX Y. Effects of centrifugal extractor cylinder inlet radius on the cylinder flow field. Chemical Industry and Engineering Progress, 2015, 34(5): 1232– 1235 (in Chinese)
|
| 69 |
GhayaH, GuizaniR, MhiriH, BournotP. CFD study of the effect of geometrical shape of separation blades on the rotor performance of an annular centrifugal extractor (ACE). Journal of Applied Fluid Mechanics, 2019, 12( 4): 1189– 1202
|
| 70 |
FanX B, YuX P, GuoY F, DengT L. Recovery of boron from underground brine by continuous centrifugal extraction with 2-ethyl-1,3-hexanediol (EHD) and its mechanism. Journal of Chemistry, 2018, 2018 : 7530837
|
| 71 |
YuX P, FanX B, GuoY F, DengT L. Recovery of lithium from underground brine by multistage centrifugal extraction using tri-isobutyl phosphate. Separation and Purification Technology, 2019, 211 : 790– 798
|
| 72 |
AbramovA M, VolobuevO I, GalievaZ N, Sobol’Y B, SolodovnikovA V, YachmenovA A, KuznetsovG I, KosogorovA V, TrusilovN N. Separating rare-earth elements on centrifugal extractors: developing technology, designing equipment, and engineering production. Theoretical Foundations of Chemical Engineering, 2020, 54( 4): 762– 768
|
| 73 |
HeQ Qiu J ChenJ F ZanM M XiaoY F. Progress in green and efficient enrichment of rare earth from the leaching liquor of ion adsorption type rare earth ores. Journal of Rare Earths, 2021, (in press)
|
| 74 |
WangL S, YuY, LiuY, LongZ Q. Centrifugal extraction of rare earths from wet-process phosphoric acid. Rare Metals, 2011, 30( 3): 211– 215
|
| 75 |
ChenH L, ZhengQ, DuanW H. Hydraulic characteristics of n-octanol/aqueous solution systems in a 25-mm annular centrifugal extractor. Separation Science and Technology, 2020, 55( 8): 1515– 1523
|
| 76 |
ChenH L, WangJ C, DuanW H, ChenJ. Hydrodynamic characteristics of 30% TBP/kerosene-HNO3 solution system in an annular centrifugal contactor. Nuclear Science and Techniques, 2019, 30( 89): 1– 12
|
| 77 |
DuanW H, SunT X, WangJ C. An industrial-scale annular centrifugal extractor for the TRPO process. Nuclear Science and Techniques, 2018, 29( 46): 1– 9
|
| 78 |
TakeuchiM, OginoH, NakabayashiH, AraiY, WashiyaT, KaseT, NakajimaY. Extraction and stripping tests of engineering-scale centrifugal contactor cascade system for spent nuclear fuel reprocessing. Journal of Nuclear Science and Technology, 2009, 46( 3): 217– 225
|
| 79 |
MeikrantzD H, GarnT G, LawJ D, MacalusoL L. A centrifugal contactor design to facilitate remote replacement. Nuclear Technology, 2011, 173( 3): 289– 299
|
| 80 |
LawJ D, MeikrantzD H, GarnT G, MacalusoL L. Advanced remote maintenance design for pilot-scale centrifugal contactors. Nuclear Technology, 2011, 173( 2): 191– 199
|
| 81 |
WangW R, ZhangP L, OuJ, LiuF S, TangK W, XuW F. Selective extraction of ECG from tea polyphenols by one step in centrifugal contactor separators: modeling and application. Industrial & Engineering Chemistry Research, 2019, 58( 5): 2027– 2035
|
| 82 |
MichailidisD, AngelisA, AligiannisN, MitakouS, SkaltsounisL. Recovery of sesamin, sesamolin, and minor lignans from sesame oil using solid support-free liquid–liquid extraction and chromatography techniques and evaluation of their enzymatic inhibition properties. Frontiers in Pharmacology, 2019, 10 : 1– 13
|
| 83 |
AngelisA, MichailidisD, AntoniadiL, StathopoulosP, TsantilaV, NuzillardJ M, RenaultJ H, SkaltsounisL A. Pilot continuous centrifugal liquid–liquid extraction of extra virgin olive oil biophenols and gram-scale recovery of pure oleocanthal, oleacein, MFOA, MFLA and hydroxytyrosol. Separation and Purification Technology, 2021, 255 : 117692
|
| 84 |
ZhangY C, LiuR Y, LiuC M, LiS N, TsaoR. Development of ultrasound-assisted mixture extraction and online extraction solution concentration coupled with countercurrent chromatography for the preparation of pure phytochemicals from Phellinus vaninii. Journal of Chromatography. B, Analytical Technologies in the Biomedical and Life Sciences, 2021, 1171 : 122619
|
| 85 |
ZhangY C, LiuR Y, LiuC M, LiS N, HouW C. Development of ultrasound-assisted centrifugal extraction combined with two countercurrent chromatography systems for the simultaneous extraction and isolation of phytochemicals. Journal of Separation Science, 2021, 44( 11): 2279– 2289
|
| 86 |
ZhangY C, LiuC M, LiS N, HouW C, TsaoR. Development of ultrasound-assisted centrifugal extraction and online solvent concentration coupled with parallel countercurrent chromatography for the preparation of purified phytochemicals: application to Lycium ruthenicum. Industrial Crops and Products, 2021, 162 : 113266
|
| 87 |
KraaiG N, van ZwolF, SchuurB, HeeresH J, de VriesJ G. Two-phase (bio)catalytic reactions in a table-top centrifugal contact separator. Angewandte Chemie, 2007, 120( 21): 3969– 3972
|
| 88 |
KraaiG N, SchuurB, van ZwolF, van de BovenkampH H, HeeresH J. Novel highly integrated biodiesel production technology in a centrifugal contactor separator device. Chemical Engineering Journal, 2009, 154( 1): 384– 389
|
| 89 |
AbduhM Y, van UldenW, KalpoeV, van de BovenkampH H, ManurungR, HeeresH J. Biodiesel synthesis from Jatropha curcas L. oil and ethanol in a continuous centrifugal contactor separator. European Journal of Lipid Science and Technology, 2013, 115( 1): 123– 131
|
| 90 |
AbduhM Y, van UldenW, van de BovenkampH H, BuntaraT, PicchioniF, ManurungR, HeeresH J. Synthesis and refining of sunflower biodiesel in a cascade of continuous centrifugal contactor separators. European Journal of Lipid Science and Technology, 2015, 117( 2): 242– 254
|
| 91 |
FayyaziE, GhobadianB, Safieddin ArdebiliS M, NajafiG, MousaviS M, Hosseinzadeh SamaniB, YueJ. Biodiesel fuel purification in a continuous centrifugal contactor separator: an environmental-friendly approach. Sustainable Energy Technologies and Assessments, 2021, 47 : 101511
|
| 92 |
IlmiM, KloekhorstA, WinkelmanJ G M, EuverinkG J W, HidayatC, HeeresH J. Process intensification of catalytic liquid–liquid solid processes: continuous biodiesel production using an immobilized lipase in a centrifugal contactor separator. Chemical Engineering Journal, 2017, 321 : 76– 85
|
| 93 |
FayyaziE, GhobadianB, van de BovenkampH H, NajafiG, HosseinzadehsamaniB, HeeresH J, YueJ. Optimization of biodiesel production over chicken eggshell-derived CaO catalyst in a continuous centrifugal contactor separator. Industrial & Engineering Chemistry Research, 2018, 57( 38): 12742– 12755
|
| 94 |
XuY, TangK, BaiZ S, WangH L. Hydrodynamic and mass-transfer characteristics of annular centrifugal contactors on the caprolactam recovery from waste liquor. Applied Mechanics and Materials, 2013, 330 : 792– 798
|
| 95 |
HaoM M, BaiZ S, WangH L, LiuW J. Removal of oil from electric desalting wastewater using centrifugal contactors. Journal of Petroleum Science Engineering, 2013, 111 : 37– 41
|
| 96 |
YangX Y, WangB J, LuoH Q, YanS L, DaiJ, BaiZ S. Efficient recovery of phenol from coal tar processing wastewater with tributylphosphane/diethyl carbonate/cyclohexane: extraction cycle and mechanism study. Chemical Engineering Research & Design, 2020, 157 : 104– 113
|
| 97 |
YangX Y, BaiZ S, WangB J, JieF P. Optimal ternary extractant for phenol removal from wastewater: modeling and application. Separation Science and Technology, 2019, 54( 1): 69– 78
|
| 98 |
JieF P, BaiZ S, YangX Y. Study on extraction of cobalt(II) by sodium laurate/pentan-1-ol/heptane/NaCl microemulsion system. Journal of Radioanalytical and Nuclear Chemistry, 2018, 315( 3): 581– 593
|
| 99 |
JieF P, BaiZ S, YangX Y. Extraction of Mn(II) from NaCl solution by NaCl/sodium oleate/n-pentanol/n-heptane microemulsion system. Separation Science and Technology, 2018, 53( 9): 1351– 1360
|
| 100 |
YangX Y, JieF P, WangB J, BaiZ S. High-efficient synergistic extraction of Co(II) and Mn(II) from wastewater via novel microemulsion and annular centrifugal extractor. Separation and Purification Technology, 2019, 209 : 997– 1006
|
| 101 |
EggertA, KoglT, ArltW, JupkeA. Computer tomographic detection of the liquid–liquid mixing and separation within the annular centrifugal contactor/extractor. Chemical Engineering Research & Design, 2019, 142 : 143– 153
|
/
| 〈 |
|
〉 |