UPFC setting to avoid active power flow loop considering wind power uncertainty

Abstract

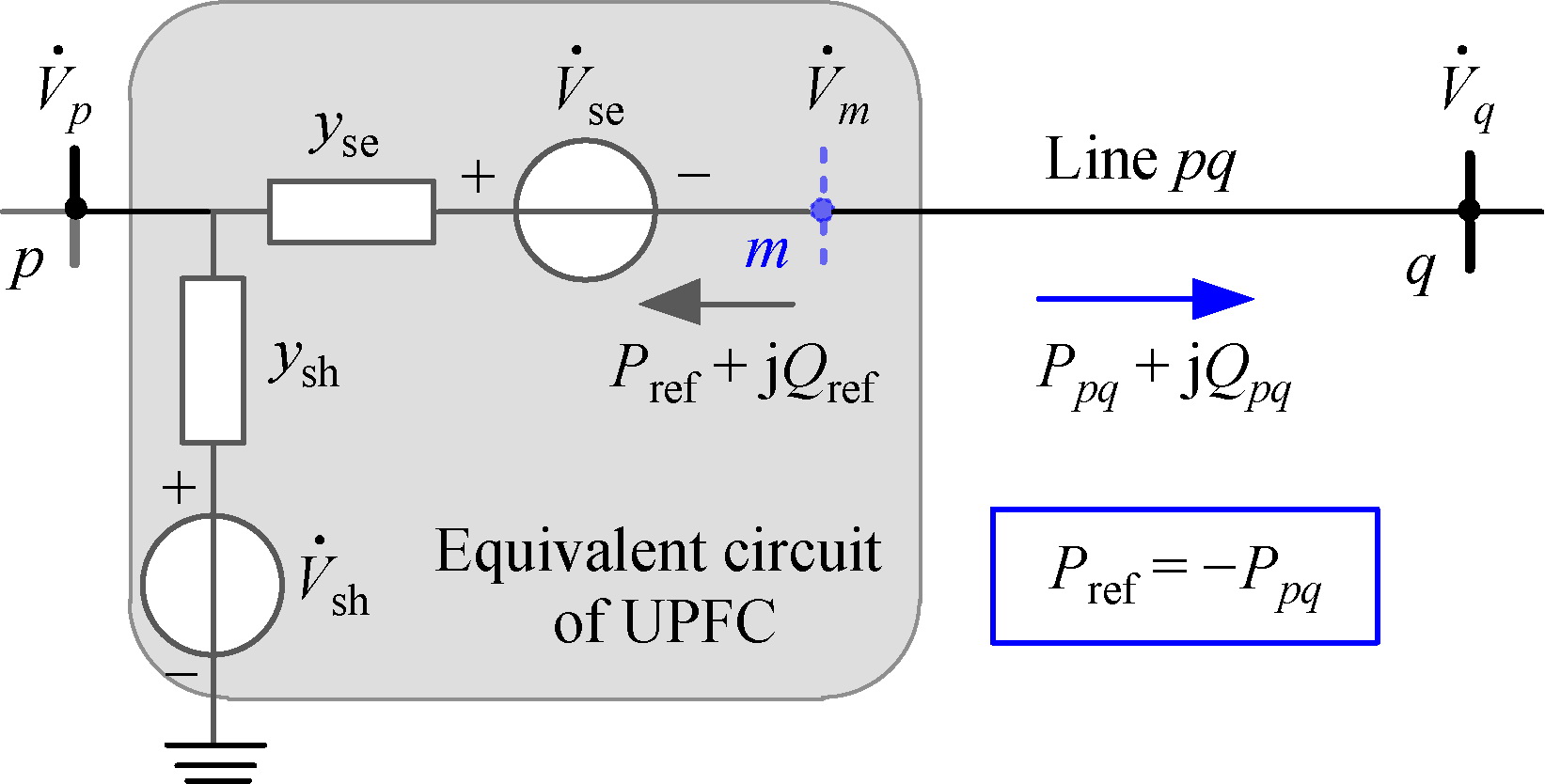
The active power loop flow (APLF) may be caused by impropriate network configuration, impropriate parameter settings, and/or stochastic bus powers. The power flow controllers, e.g., the unified power flow controller (UPFC), may be the reason and the solution to the loop flows. In this paper, the critical existence condition of the APLF is newly integrated into the simultaneous power flow model for the system and UPFC. Compared with the existing method of alternatively solving the simultaneous power flow and sensitivity-based approaching to the critical existing condition, the integrated power flow needs less iterations and calculation time. Besides, with wind power fluctuation, the interval power flow (IPF) is introduced into the integrated power flow, and solved with the affine Krawcyzk iteration to make sure that the range of active power setting of the UPFC not yielding the APLF. Compared with Monte Carlo simulation, the IPF has the similar accuracy but less time.

Key words： active power loop flow (APLF); unified power flow controller (UPFC); wind power uncertainty; interval power flow (IPF)

Acknowledgments

This work was supported by the National Natural Science Foundation of China (Grant No. 51877061).

References

1 Sadjad G, Mehrdad T H, Mohamad B B S. Unified power flow controller impact on power system predictability. IET Generation, Transmission & Distribution, 2013, 8(5): 819–827

2 Golshannavaz S, Aminifar F, Nazarpour D. Application of UPFC to enhancing oscillatory response of series-compensated wind farm integrations. IEEE Transactions on Smart Grid, 2014, 5(4): 1961–1968

3 Lin W M, Lu K H, Ou T C. Design of a novel intelligent damping controller for unified power flow controller in power system connected offshore power applications. IET Generation, Transmission & Distribution, 2015, 9(13): 1708–1717

4 Wang L, Li H W, Wu C T. Stability analysis of an integrated offshore wind and seashore wave farm fed to a power grid using a unified power flow controller. IEEE Transactions on Power Systems, 2013, 28(3): 2211–2221

5 Wei P, Ni Y X, Wu F F. Load flow tracing in power systems with circulating power. International Journal of Electrical Power & Energy Systems, 2002, 24(10): 807–813

6 Marinakis A, Glavic M, Van Cutsem T. Minimal reduction of unscheduled flows for security restoration: application to phase shifter control. IEEE Transactions on Power Systems, 2010, 25(1): 506–515

7 Lauria S, Palone F. Maximum undergrounding degree of HV sub transmission networks as dictated by unscheduled power flows. IET Generation, Transmission & Distribution, 2013, 7(11): 1202–1209

8 Cvijić S, Ilić M D. Part II: PAR flow control based on the framework for modelling and tracing of bilateral transactions and corresponding loop flows. IEEE Transactions on Power Systems, 2014, 29(6): 2715–2722

9 Sayed M A, Takeshita T. All nodes voltage regulation and line loss minimization in loop distribution systems using UPFC. IEEE Transactions on Power Electronics, 2011, 26(6): 1694–1703

10 Miller J M, Ballamat B M, Morris K N, Malinowski J H, Pastermack B M, Eilts L E. Operating problems with parallel flow. IEEE Transactions on Power Systems, 1991, 6(3): 1024–1034

11 Li S, Wang T, Zhang H, Wang L, Jiang Y, Xue J. Sensitivity-based coordination to controllable ranges of UPFCs to avoid active power loop flows. International Journal of Electrical Power & Energy Systems, 2020, 114: 105383

12 Hajian M, Rosehart W D, Zareipour H. Probabilistic power flow by Monte Carlo simulation with Latin supercube sampling. IEEE Transactions on Power Systems, 2013, 28(2): 1550–1559

13 Wang Z A, Alvarado F L. Interval arithmetic in power flow analysis. IEEE Transactions on Power Systems, 1992, 7(3): 1341–1349

14 Moore R E, Cloud M J, Kearfott R B. Introduction to Interval Analysis. Philadelphia: Society for Industrial and Applied Mathematics, 2009

15 Duan C, Jiang L, Fang W L, Liu J. Moment-SOS approach to interval power flow. IEEE Transactions on Power Systems, 2017, 32(1): 522–530

16 Ding T, Bo R, Li F X, Guo Q, Sun H, Gu W, Zhou G. Interval power flow analysis using linear relaxation and optimality-based bounds tightening (OBBT) methods. IEEE Transactions on Power Systems, 2015, 30(1): 177–188

17 Ding T, Li X, Li F, Bo R, Sun H. Interval radial power flow using extended DistFlow formulation and Krawcyzk iteration method with sparse approximate inverse preconditioner. IET Generation, Transmission & Distribution, 2015, 9(14): 1998–2006

18 Wang Y, Wu Z, Dou X, Hu M, Xu Y. Interval power flow analysis via multi-stage affine arithmetic for unbalanced distribution network. Electric Power Systems Research, 2017, 142: 1–8

19 Vaccaro A, Canizares C A. An affine arithmetic-based framework for uncertain power flow and optimal power flow studies. IEEE Transactions on Power Systems, 2017, 32(1): 274–288

20 Pereira L E S, da Costa V M. Interval analysis applied to the maximum loading point of electric power systems considering load data uncertainties. International Journal of Electrical Power & Energy Systems, 2014, 54: 334–340

21 Pereira L E S, da Costa V M, Rosa A L S. Interval arithmetic in current injection power flow analysis. International Journal of Electrical Power & Energy Systems, 2012, 43(1): 1106–1113

22 Fuerte-Esquivel C R, Acha E, Ambriz-Perez H. A comprehensive Newton-Raphson UPFC model for the quadratic power flow solution of practical power networks. IEEE Transactions on Power Systems, 2000, 15(1): 102–109

23 Ding T, Cui H T, Gu W, Wan Q L. An uncertainty power flow algorithm based on interval and affine arithmetic. Automation of Electric Power Systems, 2012, 36(13): 51–55

24 Wolfram M, Schlegel S, Westermann D. Closed loop flow detection in power system based on Floyd-Warshall algorithm. In: IEEE Manchester PowerTech, Manchester, UK, 2017, 18–22

25 Hiskens I. IEEE PES task force on benchmark systems for stability controls report on the 39-bus system (New England Reduced Model). 2020–6-9, available at website of Universidade de Sao Paulo